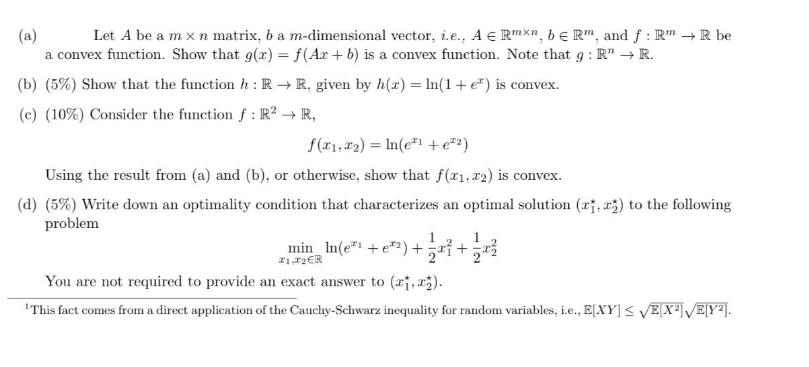
(a) Let A be a mx n matrix, b a m-dimensional vector, i.e., A Rmxn, be Rm, and f: Rm R be a convex function. Show that g(x) = f(Ar+b) is a convex function. Note that g: R" R. (b) (5%) Show that the function h: R R, given by h(x) = ln(1 + e*) is convex. (c) (10%) Consider the function f : R R, f(x1,2)= ln(e1 + e2) Using the result from (a) and (b), or otherwise, show that f(x, x2) is convex. (d) (5%) Write down an optimality condition that characterizes an optimal solution (x,x) to the following problem min In(e + ) + 2/2a + 21,72ER You are not required to provide an exact answer to (1,2). This fact comes from a direct application of the Cauchy-Schwarz inequality for random variables, i.e., E[XY] E[X]E[Y].