Answerallquestionscorrectly.

Part A

: Use the economic theories discussed in class to determine whether the statement in Italics in each of the following questions (Q1, Q2, and Q3) is True, False or Uncertain. Please explain using both words, graph(s) and where appropriate math. A word limit is given for each question - you must stick this word limit. , insert your handdrawn graphs. No points are granted for stating True, False or Uncertain, but rather for the quality of your explanation.

Question 3

If we assume that Cloud 9 is a perfectly competitive firm operating in a perfectly competitive market and initially at the long run equilibrium point of operations, increasing the wages of workers will lead to the firm shutting down in the short run and not producing in the long run.

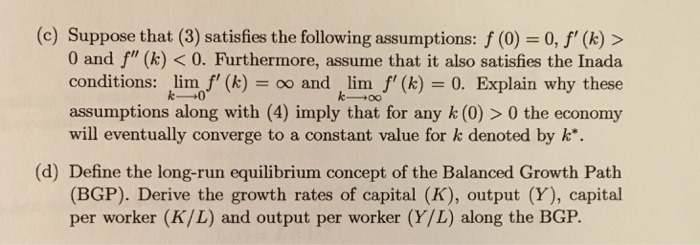
You are trying to model the number of people who own mobile phones as a Markov jump process. You assume that once someone has bought a mobile phone they will never give it up, but they can only ever own one mobile phone. You also assume that the number of potential phone owners is infinite and every individual will live forever. Finally, you assume that only one individual oan buy a phone at any instant, and that the average length of time pf minutes before the next person buys one depends on the eurrent number of mobile phone owners 1' , but not the time t. (i) Write down the generator matrix (transition rate matrix) for this process. [1] (ii) Write down the integral form of the Kolmogorov forward equations for F; [r] , where F; by time .s + t, given that there were i at time s. [3] [t] denotes the probability that there will be j mobile phone owners (iii) Obtain an expression for the probability that exaetly one mobile phone will be sold in the next minute if there are eunently i people with mobile phones. [2] (iv) After fitting the model to recent data it looks like 3111 = (0.9999991105f. Calculate the expected time before the whole population has a mobile phone if 1300.000 do at present. Comment on your answer. [4] [Total in] A pension scheme only allows retirement at exact age 65. An investigation of the mortality of the retired members of the scheme was carried out over the period 1 January 2001 to 31 December 2006. The following data were obtained: 1 April 1998 30 April 2005 1 August 2000 1 February 2001 1 June 2002 31 August 2004 1 August 2002 31 December 2006 1 March 2004 1 May 2004 30 November 2006 1 January 2005 All months should be assumed to be of equal length. (i) Explain the form or forms of censoring that are present in these data and in this observational plan. [2] (ii) Calculate the Kaplan-Meier [product-limit) estimate of the survival function 3,55 (1') om these data, stating clearly any additional assumptions that you make. [10] (iii) Estimate the force of transition from alive to dead for a two-state Markov model for age 67 last birthday, using the data given. The exposed to risk should be calculated exactly. State all assumptions required, and state the age to which the estimate would be assumed to apply. [7] [Total 19] Consider the standard version of the Solow growth model discussed in class. The production function is Y (t) = F(K(t), A(t) L(t) ), (1) while the rate of change of capital is given by K (t ) = sy (t) - 6K (t) . (2) Labor L (t) and the "effectiveness of labor" A (t) are assumed to grow at the exogenous rates n and g, respectively. (a) Assuming that (1) exhibits constant returns to scale, show that the production function can be written in intensive form as y (t ) = f (k(t)), (3 where y (t) = Y (t) / (A (t) L (t)) and k (t) = K (t) / (A(t) L (t)). (b) Show that (2) can be written in intensive form as k ( t ) = sf ( k (t) ) - (n+ g + 8)k(t). (4) What is the interpretation of (4)?(c) Suppose that (3) satisfies the following assumptions: f (0) = 0, f' (k) > 0 and f" (k) 0 the economy will eventually converge to a constant value for k denoted by k*. (d) Define the long-run equilibrium concept of the Balanced Growth Path (BGP). Derive the growth rates of capital (K), output (Y), capital per worker (K/L) and output per worker (Y/L) along the BGP.2. Consider a simplified version of the Solow model discussed in class with no depreciation of the capital stock (i.e. 6 = 0). Assume that the production function is given by: Y (t) = A (t) F (K (t ) , L (t ) ). Since this specification implies that A (t) affects both capital and la- bor symmetrically, this case is typically referred to as one of "neutral technological change" as opposed to the case of "labor-augmenting technological change" considered in class. Furthermore, assume that function F (.) is Cobb-Douglas: F (K, L) = KoLI-, where 0