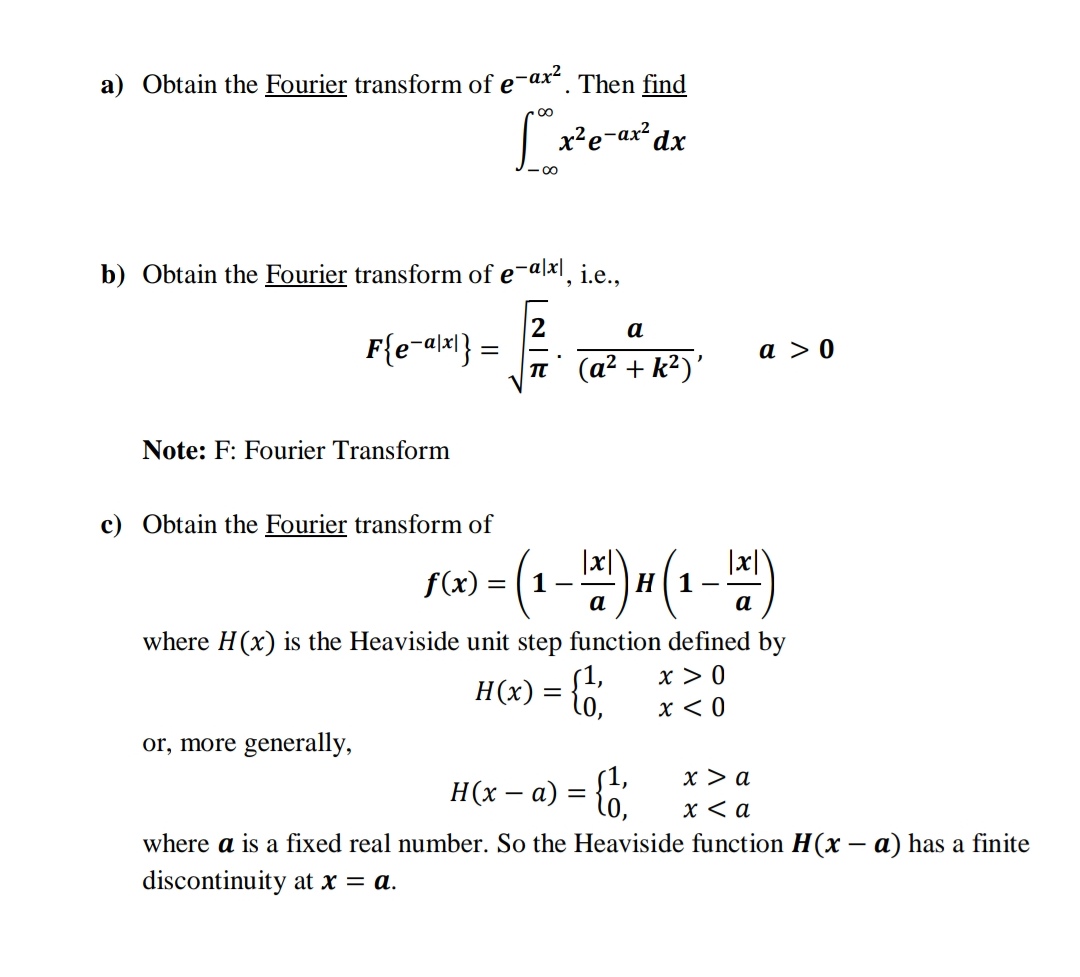
a) Obtain the Fourier transform of e-ax. Then find So x -0 b) Obtain the Fourier transform of e-alx, i.e., F{e-axl}: Note: F: Fourier Transform = c) Obtain the Fourier transform of or, more generally, NIE a (a+k)' . a> 0 F(x) = (1-1) H (1-x1) a a where H (x) is the Heaviside unit step function defined by H(x) = {1, x > 0 x < 0 x > a H(x a) = {1 x < a where a is a fixed real number. So the Heaviside function H(x a) has a finite discontinuity at x = a. 2.3 Definition of the Fourier Transform and Examples We use the Fourier integral formula (2.2.4) to give a formal definition of the Fourier transform. DEFINITION 2.3.1 The Fourier transform of f(x) is denoted by F{f(x)} = F(k), kER, and defined by the integral 1 2 F{f(x)} = F(k)=- - e-ika f(x)dx, where is called the Fourier transform operator or the Fourier transfor- mation and the factor is obtained by splitting the factor involved in Fourier Transforms and Their Applications (2.2.4). This is often called the complex Fourier transform. A sufficient condi- tion for f(x) to have a Fourier transform is that f(x) is absolutely integrable on (-, ). The convergence of the integral (2.3.1) follows at once from the fact that f(x) is absolutely integrable. In fact, the integral converges uniformly with respect to k. Physically, the Fourier transform F(k) can be interpreted as an integral superposition of an infinite number of sinusoidal oscillations with different wavenumbers k (or different wavelengths A=2). 1 {F(k)} = f(x) = - S eikz F(k) dk, 2 Thus, the definition of the Fourier transform is restricted to absolutely inte- grable functions. This restriction is too strong for many physical applications. Many simple and common functions, such as constant function, trigonomet- ric functions sin ax, cos ax, exponential functions, and "H(x) do not have Fourier transforms, even though they occur frequently in applications. The integral in (2.3.1) fails to converge when f(x) is one of the above elemen- tary functions. This is a very unsatisfactory feature of the theory of Fourier transforms. However, this unsatisfactory feature can be resolved by means of a natural extension of the definition of the Fourier transform of a general- ized function, f(x) in (2.3.1). We follow Lighthill (1958) and Jones (1982) to discuss briefly the theory of the Fourier transforms of good functions. The inverse Fourier transform, denoted by F-1{F(k)} = f(x), is defined by F{f(t)}=F(v) = (2.3.1) -00 where is called the inverse Fourier transform operator. 19 [ f(t)e-2 vit dt, Clearly, both and 1 are linear integral operators. In applied math- ematics, a usually represents a space variable and k(-2) is a wavenum- ber variable where A is the wavelength. However, in electrical engineering, z is replaced by the time variable t and k is replaced by the frequency vari- able w(27v) where is the frequency in cycles per second. The function F(w) F{f(t)} is called the spectrum of the time signal function f(t). In elec- trical engineering literature, the Fourier transform pairs are defined slightly differently by (2.3.2) (2.3.3) and 1 2ivt F-{F(v)} = f(t) = [ F(v)erive du = 2 - 20 j - F(w)eiwt dw, (2.3.4) where w=2TV is called the angular frequency. The Fourier integral formula implies that any function of time f(t) that has a Fourier transform can be equally specified by its spectrum. Physically, the signal f(t) is represented as INTEGRAL TRANSFORMS and THEIR APPLICATIONS an integral superposition of an infinite number of sinusoidal oscillations with different frequencies w and complex amplitudes F(w). Equation (2.3.4) is called the spectral resolution of the signal f(t), and (w) is called the spectral density. In summary, the Fourier transform maps a function (or signal) of time t to a function of frequency w. In the same way as the Fourier series expansion of a periodic function decomposes the function into harmonic components, the Fourier transform generates a function (or signal) of a continuous variable whose value represents the frequency content of the original signal. This led to the successful use of the Fourier transform to analyze the form of time-varying signals in electrical engineering and seismology. Next we give examples of Fourier transforms.