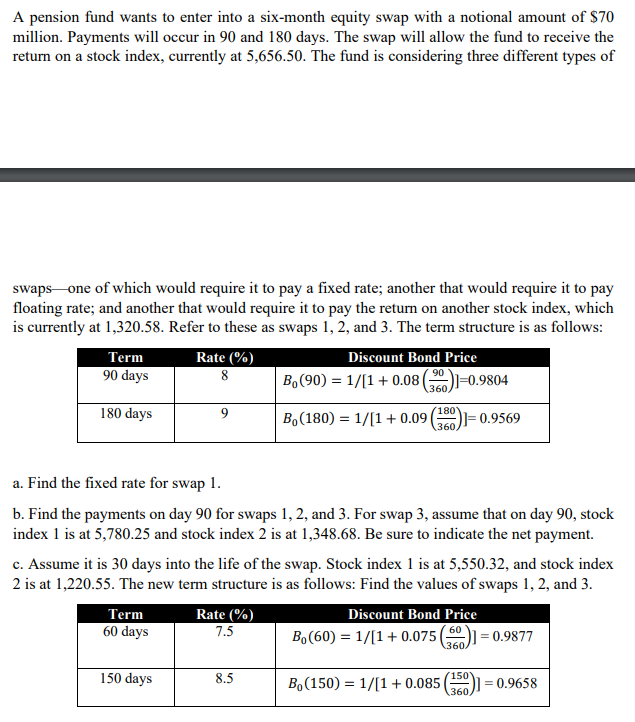
A pension fund wants to enter into a six-month equity swap with a notional amount of $70 million. Payments will occur in 90 and 180 days. The swap will allow the fund to receive the return on a stock index, currently at 5,656.50. The fund is considering three different types of swaps-one of which would require it to pay a fixed rate; another that would require it to pay floating rate; and another that would require it to pay the return on another stock index, which is currently at 1,320.58. Refer to these as swaps 1, 2, and 3. The term structure is as follows: Term Rate(%) Discount Bond Price 90 days 8 B.(90) = 1/[1 + 0.08 1=0.9804 90 360 9 180 days 180 B.(180) = 1/(1 + 0.09 (360)=0.9569 a. Find the fixed rate for swap 1. b. Find the payments on day 90 for swaps 1, 2, and 3. For swap 3, assume that on day 90, stock index 1 is at 5,780.25 and stock index 2 is at 1,348.68. Be sure to indicate the net payment. c. Assume it is 30 days into the life of the swap. Stock index 1 is at 5,550.32, and stock index 2 is at 1,220.55. The new term structure is as follows: Find the values of swaps 1, 2, and 3. Term Rate (%) Discount Bond Price 60 days 7.5 B (60) = 1/(1+0.075 = 0.9877 60 360 150 days 8.5 B.(150) = 1/(1+0.085 150 360 = 0.9658 A pension fund wants to enter into a six-month equity swap with a notional amount of $70 million. Payments will occur in 90 and 180 days. The swap will allow the fund to receive the return on a stock index, currently at 5,656.50. The fund is considering three different types of swaps-one of which would require it to pay a fixed rate; another that would require it to pay floating rate; and another that would require it to pay the return on another stock index, which is currently at 1,320.58. Refer to these as swaps 1, 2, and 3. The term structure is as follows: Term Rate(%) Discount Bond Price 90 days 8 B.(90) = 1/[1 + 0.08 1=0.9804 90 360 9 180 days 180 B.(180) = 1/(1 + 0.09 (360)=0.9569 a. Find the fixed rate for swap 1. b. Find the payments on day 90 for swaps 1, 2, and 3. For swap 3, assume that on day 90, stock index 1 is at 5,780.25 and stock index 2 is at 1,348.68. Be sure to indicate the net payment. c. Assume it is 30 days into the life of the swap. Stock index 1 is at 5,550.32, and stock index 2 is at 1,220.55. The new term structure is as follows: Find the values of swaps 1, 2, and 3. Term Rate (%) Discount Bond Price 60 days 7.5 B (60) = 1/(1+0.075 = 0.9877 60 360 150 days 8.5 B.(150) = 1/(1+0.085 150 360 = 0.9658