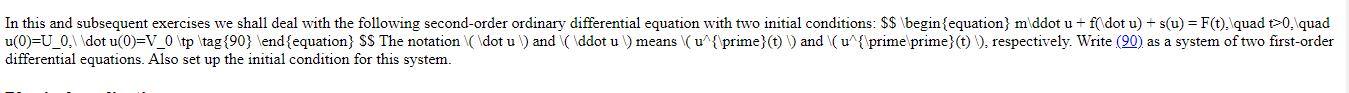
In this and subsequent exercises we shall deal with the following second-order ordinary differential equation with two initial conditions: $$ \begin{equation} m\ddot u + f(\dot u) + s(u) = F(t).\quad t>0,\quad u(0) U_0,\\\dot u(0)=V_0 \tp \tag (90) \end{equation) SS The notation \(\dot u) and \(\ddot u \) means \(u^{\prime} (t) \) and \(u^{\prime\prime} (t) \), respectively. Write (90) as a system of two first-order differential equations. Also set up the initial condition for this system.