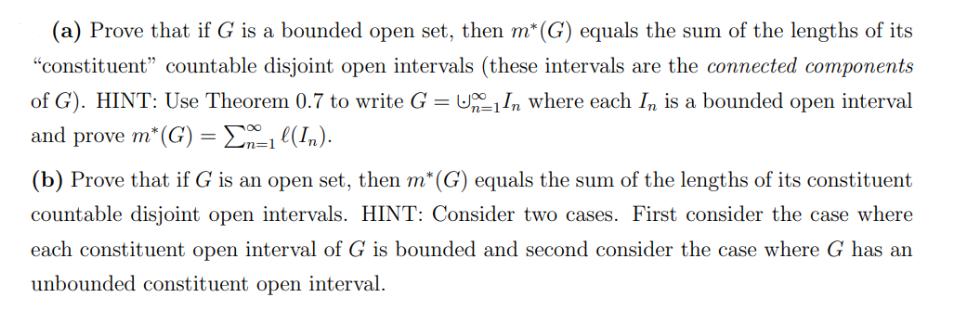
(a) Prove that if G is a bounded open set, then m*(G) equals the sum of the lengths its "constituent" countable disjoint open intervals (these intervals are the connected components of G). HINT: Use Theorem 0.7 to write G = U1In where each In is a bounded open interval and prove m*(G) = -1 (In). (b) Prove that if G is an open set, then m* (G) equals the sum of the lengths of its constituent countable disjoint open intervals. HINT: Consider two cases. First consider the case where each constituent open interval of G is bounded and second consider the case where G has an unbounded constituent open interval.