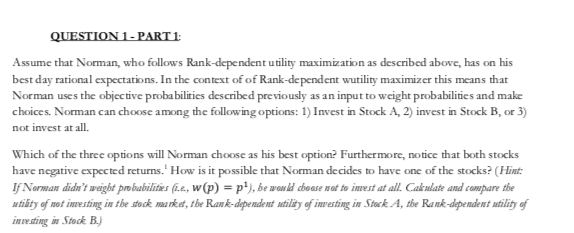
a QUESTION 1: DECISION UNDER RISK Norman maximizes expected happiness. He is a rich person with a wealth of W=5,000,000 DKK, with utility function u(z) = ln(2) and is deciding how to invest his portfolio. Norman is a Rank-dependent utility maximizer with a probability weighting function given by w(p) = p.1. If a given stock costs and pays either X or Y, with X greater than Y, with objective probability pand 1-p, respectively, then Norman maximizes the following Rank-dependent utility valuation: RDU =w(p) u(W + x 2) + (1 (P2)(W +Y C) Norman can choose from two different stocks, stock A or stock B. Each Stock A costs 500, and cach A stock pays out either 600 or 200 DKK per stock, with 70% and 30% probability, respectively. Stock B also is priced at 500 per share but yields either 1500 or 100 DKK per stock with 10% and 90% probability, respectively. These probabilities represent the actual objective probability distributions of returns of each stock. Therefore, someone with rational expectations should use such probabilities when deciding whether or not to buy the stocks. Norman will invest all his wealth in either Stock A or in Stock B, or in just cash. This means that he will buy 10,000 shares of either Stock A or Stock B if he decides to invest at all. For example, if he buys Stock A and the good outcome is realized, Norman will receive 10.000*600 = 6,000,000 DKK. This question is divided in 2 parts. QUESTION 1 - PART 1 Assume that Norman, who follows Rank-dependent utility maximization as described above, has on his best day rational expectations. In the context of of Rank-dependent wutility maximizer this means that Norman uses the objective probabilities described previously as an input to weight probabilities and make choices. Norman can choose among the following options: 1) Invest in Stock A, 2) invest in Stock B, or 3) not invest at all. Which of the three options will Norman choose as his best option? Furthermore, notice that both stocks have negative expected returns. How is it possible that Norman decides to have one of the stocks? (Hint If Norman didn't weight probabilites (.e, w(p) = p'), be would choose not to invest at all. Calmlate and compare the utility of not investing in the stock market, the Rank-dependent utility of investing in Stock A, the Rank-dependent utility of investing in Stock B.) a QUESTION 1: DECISION UNDER RISK Norman maximizes expected happiness. He is a rich person with a wealth of W=5,000,000 DKK, with utility function u(z) = ln(2) and is deciding how to invest his portfolio. Norman is a Rank-dependent utility maximizer with a probability weighting function given by w(p) = p.1. If a given stock costs and pays either X or Y, with X greater than Y, with objective probability pand 1-p, respectively, then Norman maximizes the following Rank-dependent utility valuation: RDU =w(p) u(W + x 2) + (1 (P2)(W +Y C) Norman can choose from two different stocks, stock A or stock B. Each Stock A costs 500, and cach A stock pays out either 600 or 200 DKK per stock, with 70% and 30% probability, respectively. Stock B also is priced at 500 per share but yields either 1500 or 100 DKK per stock with 10% and 90% probability, respectively. These probabilities represent the actual objective probability distributions of returns of each stock. Therefore, someone with rational expectations should use such probabilities when deciding whether or not to buy the stocks. Norman will invest all his wealth in either Stock A or in Stock B, or in just cash. This means that he will buy 10,000 shares of either Stock A or Stock B if he decides to invest at all. For example, if he buys Stock A and the good outcome is realized, Norman will receive 10.000*600 = 6,000,000 DKK. This question is divided in 2 parts. QUESTION 1 - PART 1 Assume that Norman, who follows Rank-dependent utility maximization as described above, has on his best day rational expectations. In the context of of Rank-dependent wutility maximizer this means that Norman uses the objective probabilities described previously as an input to weight probabilities and make choices. Norman can choose among the following options: 1) Invest in Stock A, 2) invest in Stock B, or 3) not invest at all. Which of the three options will Norman choose as his best option? Furthermore, notice that both stocks have negative expected returns. How is it possible that Norman decides to have one of the stocks? (Hint If Norman didn't weight probabilites (.e, w(p) = p'), be would choose not to invest at all. Calmlate and compare the utility of not investing in the stock market, the Rank-dependent utility of investing in Stock A, the Rank-dependent utility of investing in Stock B.)