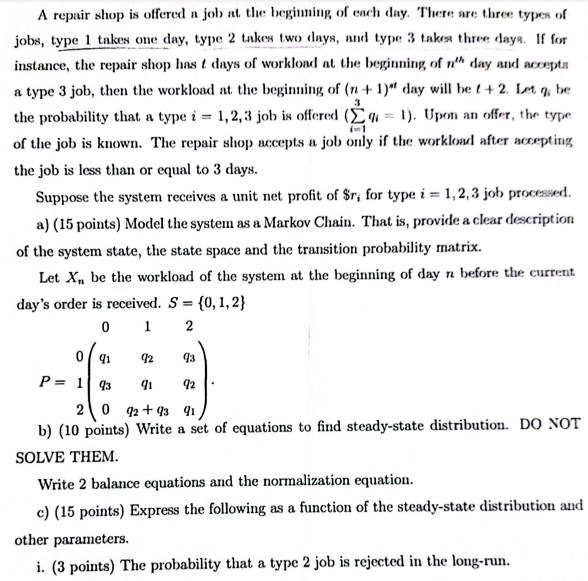
A repair shop is offered a job at the beginning of each day. There are three types of jobs, type 1 takes one day, type 2 takes two days, and type 3 takes three days. If for instance, the repair shop hast days of workload at the beginning of n day and accepts a type 3 job, then the workload at the beginning of (n+1)" day will be t + 2. Let q, be the probability that a type i = 1,2,3 job is offered ( = 1). Upon an offer, the type of the job is known. The repair shop accepts a job only if the workload after accepting the job is less than or equal to 3 days. 3 Suppose the system receives a unit net profit of $r, for type i= 1,2,3 job processed. a) (15 points) Model the system as a Markov Chain. That is, provide a clear description of the system state, the state space and the transition probability matrix. Let X be the workload of the system at the beginning of day n before the current day's order is received. S= {0, 1,2} 0 1 2 0 91 92 93 P= 1 93 91 92 2 092 +93 91 b) (10 points) Write a set of equations to find steady-state distribution. DO NOT SOLVE THEM. Write 2 balance equations and the normalization equation. c) (15 points) Express the following as a function of the steady-state distribution and other parameters. i. (3 points) The probability that a type 2 job is rejected in the long-run.