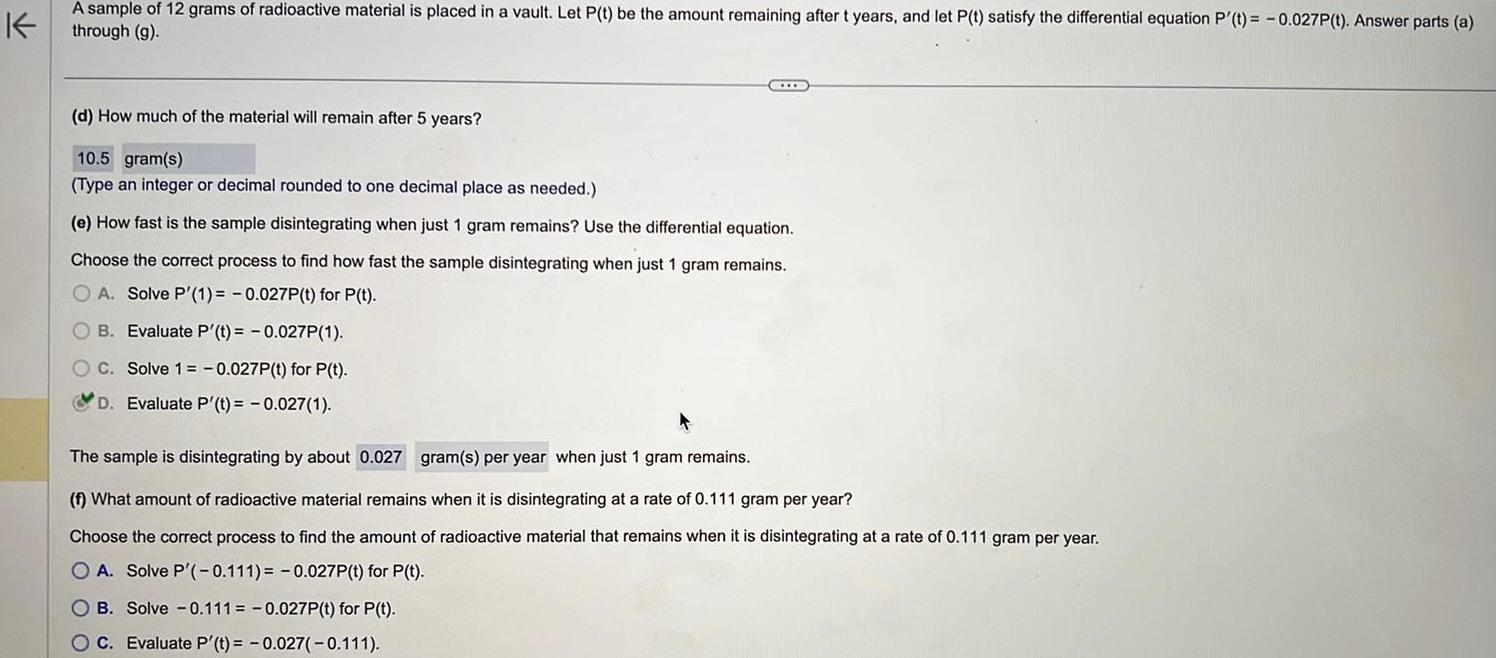
A sample of 12 grams of radioactive material is placed in a vault. Let P(t) be the amount remaining after t years, and let P(t) satisfy the differential equation P'(t) = -0.027P(t). Answer parts (a) through (g). (d) How much of the material will remain after 5 years? 10.5 gram(s) (Type an integer or decimal rounded to one decimal place as needed.) (e) How fast is the sample disintegrating when just 1 gram remains? Use the differential equation. Choose the correct process to find how fast the sample disintegrating when just 1 gram remains. OA. Solve P'(1) -0.027P(t) for P(t). OB. Evaluate P'(t) = -0.027P(1). C. Solve 1=-0.027P(t) for P(t). D. Evaluate P'(t)= -0.027(1). The sample is disintegrating by about 0.027 gram(s) per year when just 1 gram remains. (f) What amount of radioactive material remains when it is disintegrating at a rate of 0.111 gram per year? Choose the correct process to find the amount of radioactive material that remains when it is disintegrating at a rate of 0.111 gram per year. OA. Solve P'(-0.111) = -0.027P(t) for P(t). B. Solve -0.111 = -0.027P(t) for P(t). OC. Evaluate P'(t)= -0.027(-0.111).