Answered step by step
Verified Expert Solution
Question
1 Approved Answer
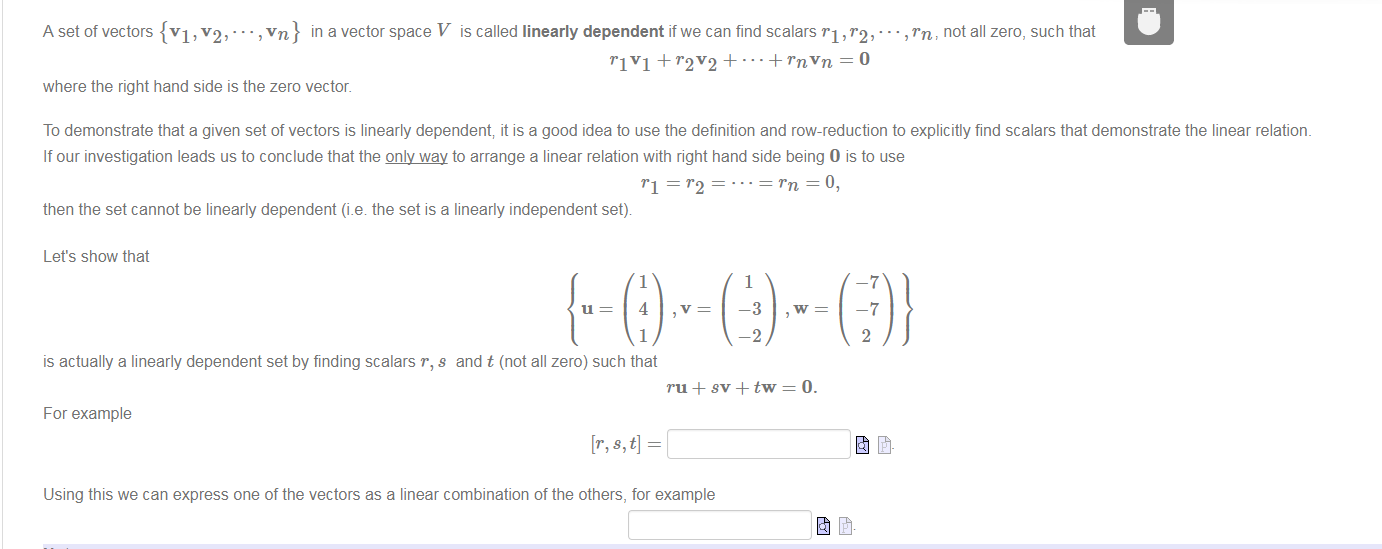
A set of vectors{v 1 ,v 2 ,,v n } in a vector spaceV is called linearly dependent if we can find scalarsr 1 ,r
A set of vectors{v
1
,v
2
,,v
n
}
in a vector spaceV
is calledlinearly dependentif we can find scalarsr
1
,r
2
,,r
n
,not all zero, such that
r
1
v
1
+r
2
v
2
++r
n
v
n
=0
where the right hand side is the zero vector.
To demonstrate that a given set of vectors is linearly dependent, it is a good idea to use the definition and row-reduction to explicitlyfind scalars that demonstrate the linear relation.
If our investigation leads us to conclude that theonly wayto arrange a linear relation with right hand side being0
is to use
r
1
=r
2
==r
n
=0
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


