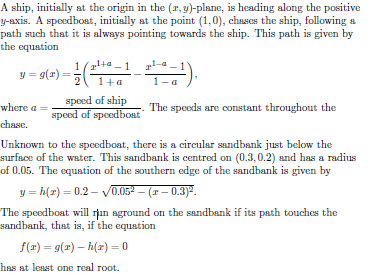
A ship, initially at the origin in the r,y)-plane, is heading along the positive y-axis. A speedboat, initially at the point (1,0), chases the ship, following a path such that it is always pointing towards the ship. This path is given by the equation 1/10-1 y=9(*) 2 1+ 1- speed of ship where a = The speeds are constant throughout the speed of speedboat chase. Unknown to the speedboat, there is a circular sandbank just below the surface of the water. This sandbank is centred on (0.3, 0.2) and has a radius of 0.05. The equation of the southern edge of the sandbank is given by y=h(x) = 0.2 - 0.062 - (1 -0.3) The speedboat will sin aground on the sandbank if its path touches the sandbank, that is, if the equation f(1) = 9(a) -h(x) = 0 has at least one real root. [4] [1] The speedboat travels at a constant speed of 40 km h, and the ship has a maximum speed of 25 km h-1 (a) Show graphically that if the ship travels at its maximum speed, then the speedboat will run aground on the sandbank. Use the Newton-Raphson method to find the point at which it runs aground, to an accurncy of three decimal places (b) Show graphically that if a = 0.225, then the speedboat will miss the sandbank. (c) The captain of the ship wants to know the minimum speed of the ship for which the speedboat will run aground (assuming that the speedboat maintains its constant speed). Your results from parts (a) and (b) give you an interval for a within which the minimum value of a must lie. By repeatedly bisecting this interval, find this minimum value of a, correct to one decimal place, and give the corresponding speed of the ship. Your solution should include suitable ertracts from Mozima worksheets, showing the problem input and the key results, in addition to an interpretation of the relevant Morimo output and how it relates to answering the questions. In part (c) there is no need to show every Marima output, as long as your method and final results are clear. [5] A ship, initially at the origin in the r,y)-plane, is heading along the positive y-axis. A speedboat, initially at the point (1,0), chases the ship, following a path such that it is always pointing towards the ship. This path is given by the equation 1/10-1 y=9(*) 2 1+ 1- speed of ship where a = The speeds are constant throughout the speed of speedboat chase. Unknown to the speedboat, there is a circular sandbank just below the surface of the water. This sandbank is centred on (0.3, 0.2) and has a radius of 0.05. The equation of the southern edge of the sandbank is given by y=h(x) = 0.2 - 0.062 - (1 -0.3) The speedboat will sin aground on the sandbank if its path touches the sandbank, that is, if the equation f(1) = 9(a) -h(x) = 0 has at least one real root. [4] [1] The speedboat travels at a constant speed of 40 km h, and the ship has a maximum speed of 25 km h-1 (a) Show graphically that if the ship travels at its maximum speed, then the speedboat will run aground on the sandbank. Use the Newton-Raphson method to find the point at which it runs aground, to an accurncy of three decimal places (b) Show graphically that if a = 0.225, then the speedboat will miss the sandbank. (c) The captain of the ship wants to know the minimum speed of the ship for which the speedboat will run aground (assuming that the speedboat maintains its constant speed). Your results from parts (a) and (b) give you an interval for a within which the minimum value of a must lie. By repeatedly bisecting this interval, find this minimum value of a, correct to one decimal place, and give the corresponding speed of the ship. Your solution should include suitable ertracts from Mozima worksheets, showing the problem input and the key results, in addition to an interpretation of the relevant Morimo output and how it relates to answering the questions. In part (c) there is no need to show every Marima output, as long as your method and final results are clear. [5]