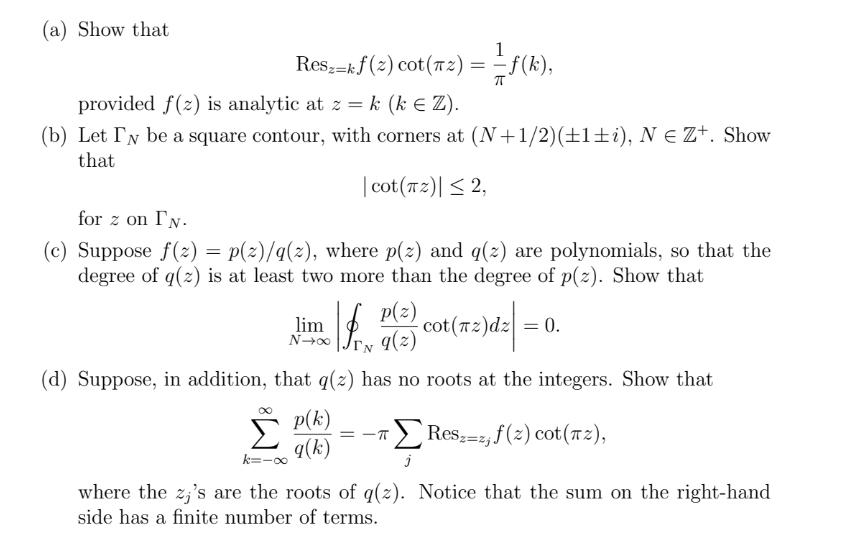
(a) Show that 1 = Resz-kf(2) cot(T2) = f(k), provided f(z) is analytic at z = k (k Z). (b) Let I be a square contour, with corners at (N+1/2)(1+i), N = Z+. Show that for z on TN. |cot(TZ)|2, (c) Suppose f(z) = p(2)/q(2), where p(z) and q(2) are polynomials, so that the degree of q(2) is at least two more than the degree of p(z). Show that | cot(T2)dz = 0. p(z) lim N TN 9(2) (d) Suppose, in addition, that a(z) has no roots at the integers. Show that p(k) q(k) k=- == T Resz=z, f(2) cot (TZ), j where the zi's are the roots of q(2). Notice that the sum on the right-hand side has a finite number of terms.