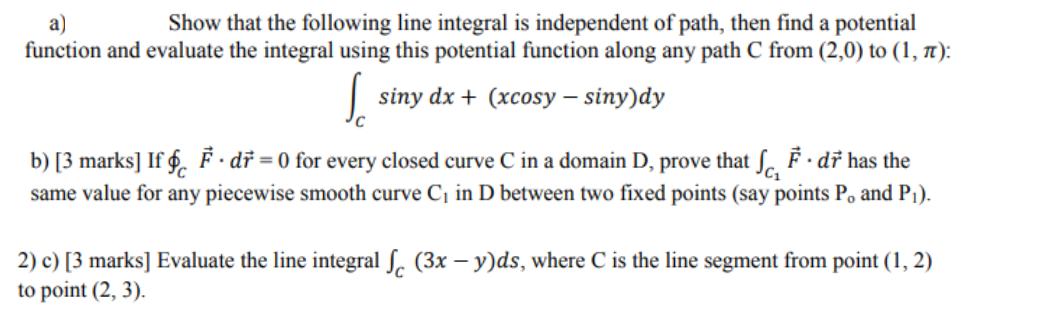
a) Show that the following line integral is independent of path, then find a potential function and evaluate the integral using this potential function along any path C from (2,0) to (1, ): So siny dx + (xcosy - siny)dy b) [3 marks] If F d = 0 for every closed curve C in a domain D, prove that c d has the same value for any piecewise smooth curve C in D between two fixed points (say points P, and P). 2) c) [3 marks] Evaluate the line integral (3x - y)ds, where C is the line segment from point (1, 2) to point (2, 3).