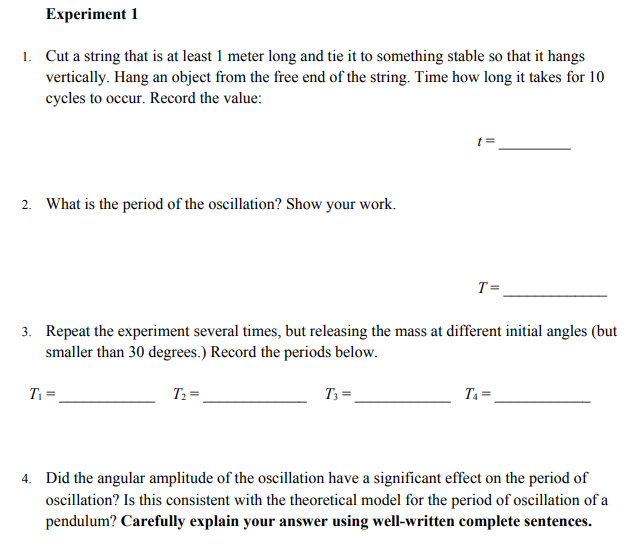
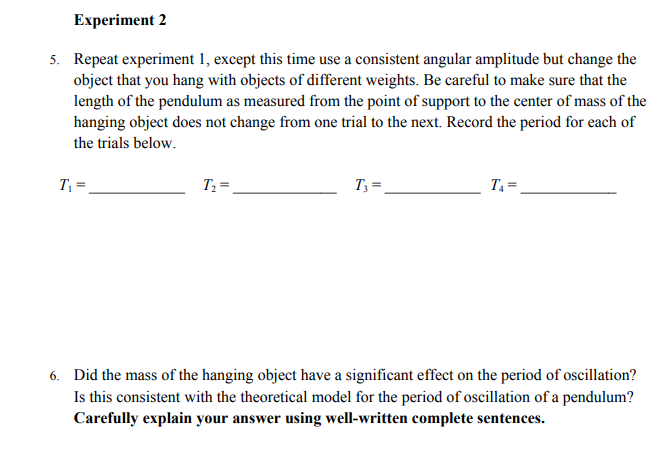
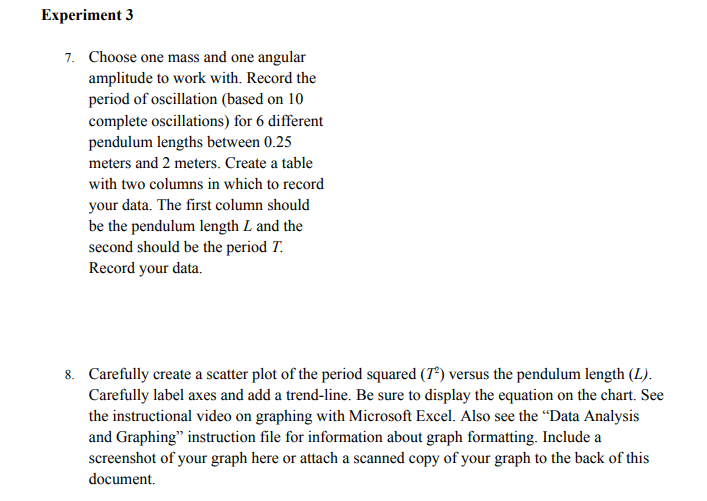
A simple pendulum takes the same amount of time to complete each cycle of its motion. When considering what could affect the time per cycle or period of the motion, we can consider at least three factors: The amplitude of the swing The mass of the pendulum bob The length of the pendulum To determine how these factors affect the pendulum period, you can do a controlled experiment in which the period of oscillation is measured while only changing one variable at a time. In this experiment, you will use a stopwatch to measure the period of a pendulum and determine how it is affected by each of the described factors. Learning Objectives 1. Students will determine how the period of a simple pendulum depends on its amplitude, mass, and length. Materials You will need string and some objects to hang from it. For string you can use dental floss, thread from a sewing kit, fishing line, shoe laces, etc. Be creative, but be sure that the mass you hang from it is much heavier than the string. To make measurements you will need a stopwatch (such as the one on your cell phone) and a ruler or some other precise measurement tool. If you cannot find a ruler, remember that US letter paper is 8.5 X 11.0 inches and 1 inch = 2.54 cm. You should be able to do a fine job making length measurements if you put in the effort. Experiment 1 1. Cut a string that is at least 1 meter long and tie it to something stable so that it hangs vertically. Hang an object from the free end of the string. Time how long it takes for 10 cycles to occur. Record the value: 2. What is the period of the oscillation? Show your work. t= T= 3. Repeat the experiment several times, but releasing the mass at different initial angles (but smaller than 30 degrees.) Record the periods below. T = T = T = T = 4. Did the angular amplitude of the oscillation have a significant effect on the period of oscillation? Is this consistent with the theoretical model for the period of oscillation of a pendulum? Carefully explain your answer using well-written complete sentences. Experiment 2 5. Repeat experiment 1, except this time use a consistent angular amplitude but change the object that you hang with objects of different weights. Be careful to make sure that the length of the pendulum as measured from the point of support to the center of mass of the hanging object does not change from one trial to the next. Record the period for each of the trials below. T = T = T = T = 6. Did the mass of the hanging object have a significant effect on the period of oscillation? Is this consistent with the theoretical model for the period of oscillation of a pendulum? Carefully explain your answer using well-written complete sentences. Experiment 3 7. Choose one mass and one angular amplitude to work with. Record the period of oscillation (based on 10 complete oscillations) for 6 different pendulum lengths between 0.25 meters and 2 meters. Create a table with two columns in which to record your data. The first column should be the pendulum length L and the second should be the period T. Record your data. 8. Carefully create a scatter plot of the period squared (72) versus the pendulum length (L). Carefully label axes and add a trend-line. Be sure to display the equation on the chart. See the instructional video on graphing with Microsoft Excel. Also see the "Data Analysis and Graphing" instruction file for information about graph formatting. Include a screenshot of your graph here or attach a scanned copy of your graph to the back of this document. 9. Use the model for how the period of a pendulum squared depends on its length along with the fit parameters of your linear fit to determine an experimental value for the free- fall gravitation acceleration on Earth. Show your work. (You may find it helpful to review the "Data Analysis and Graphing" instruction file to assist in understanding how to compare models to fit equations.) 10. What is the percent error in your experimental value for the free-fall gravitational acceleration (g) of Earth? Show your work. 11. Conclusion Question: According to the model, how does the period of a pendulum swing depend on its length? Carefully state the mathematical relationship between them. Does your experiment confirm this relationship? Justify your answer by referencing important features in your graph. Carefully explain your answer using well-written complete sentences. 12. Take a picture of your experimental setup (including the objects used) and put it in the space below.