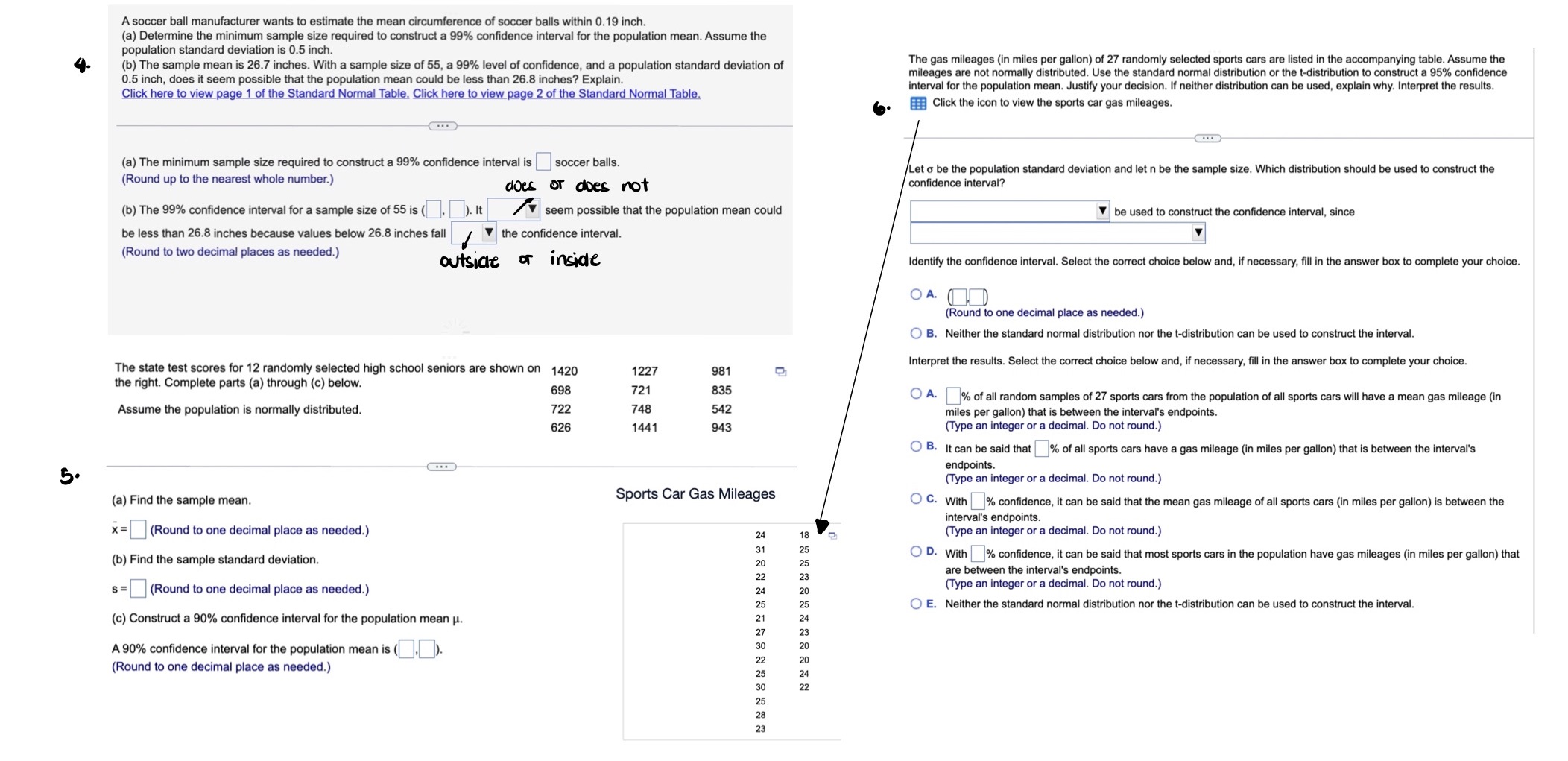
A soccer ball manufacturer wants to estimate the mean circumference of soccer balls within 0.19 inch. (a) Determine the minimum sample size required to construct a 99% confidence interval for the population mean. Assume the population standard deviation is 0.5 inch. 4. (b) The sample mean is 26.7 inches. With a sample size of 55, a 99% level of confidence, and a population standard deviation of The gas mileages (in miles per gallon) of 27 randomly selected sports cars are listed in the accompanying table. Assume the 0.5 inch, does it seem possible that the population mean could be less than 26.8 inches? Explain. mileages are not normally distributed. Use the standard normal distribution or the t-distribution to construct a 95% confidence interval for the population mean. Justify your decision. If neither distribution can be used, explain why. Interpret the results. Click here to view page 1 of the Standard Normal Table. Click here to view page 2 of the Standard Normal Table. Click the icon to view the sports car gas mileages. (a) The minimum sample size required to construct a 99% confidence interval is soccer balls. Let o be the population standard deviation and let n be the sample size. Which distribution should be used to construct the (Round up to the nearest whole number.) does Or does not confidence interval? (b) The 99% confidence interval for a sample size of 55 is (. ). it seem possible that the population mean could be used to construct the confidence interval, since be less than 26.8 inches because values below 26.8 inches fall the confidence interval. (Round to two decimal places as needed.) outside or inside Identify the confidence interval. Select the correct choice below and, if necessary, fill in the answer box to complete your choice. OAD Round to one decimal place as needed.) O B. Neither the standard normal distribution nor the t-distribution can be used to construct the interval. The state test scores for 12 randomly selected high school seniors are shown on 1420 Interpret the results. Select the correct choice below and, if necessary, fill in the answer box to complete your choice. 1227 981 the right. Complete parts (a) through (c) below. 698 721 835 O A. % of all random samples of 27 sports cars from the population of all sports cars will have a mean gas mileage (in Assume the population is normally distributed. 722 748 542 miles per gallon) that is between the interval's endpoints. 626 1441 943 Type an integer or a decimal. Do not round.) O B. It can be said that % of all sports cars have a gas mileage (in miles per gallon) that is between the interval's endpoints. 5. (Type an integer or a decimal. Do not round.) (a) Find the sample mean. Sports Car Gas Mileages O C. With % confidence, it can be said that the mean gas mileage of all sports cars (in miles per gallon) is between the interval's endpoints. x= (Round to one decimal place as needed.) 18 (Type an integer or a decimal. Do not round.) (b) Find the sample standard deviation. O D. With % confidence, it can be said that most sports cars in the population have gas mileages (in miles per gallon) that are between the interval's endpoints. s= (Round to one decimal place as needed.) Type an integer or a decimal. Do not round.) O E. Neither the standard normal distribution nor the t-distribution can be used to construct the interval. (c) Construct a 90% confidence interval for the population mean u. A 90% confidence interval for the population mean is (). Round to one decimal place as needed.)








