Question
A sphere of radius R rotates steadily about its vertical axis with an angular velocity (rad/sec) in an infinite Newtonian liquid of viscosity . Assume
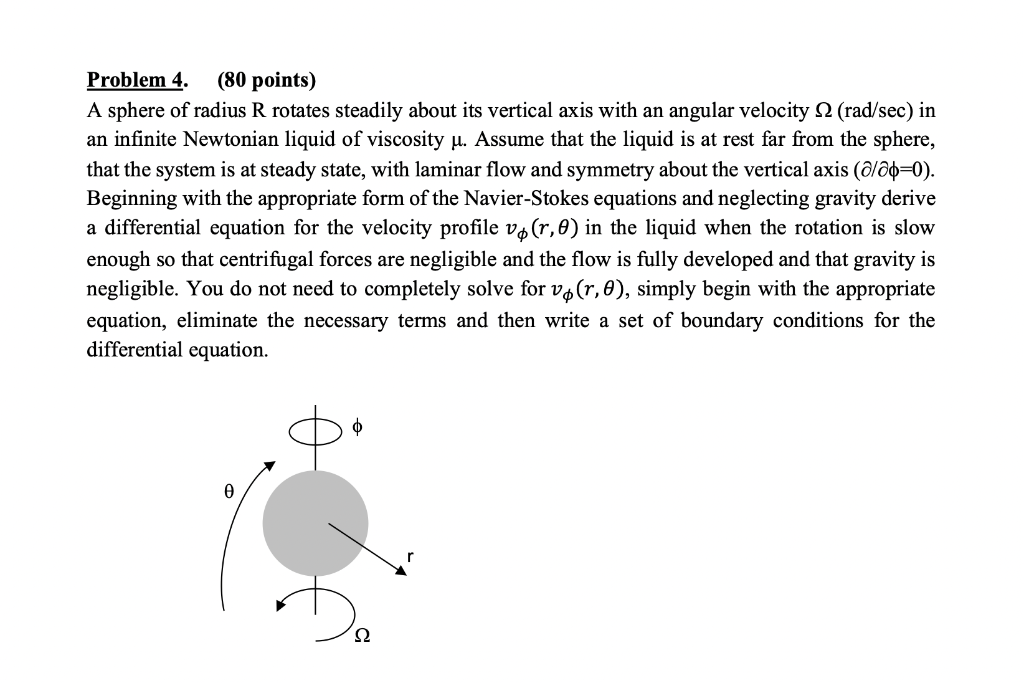 A sphere of radius R rotates steadily about its vertical axis with an angular velocity (rad/sec) in an infinite Newtonian liquid of viscosity . Assume that the liquid is at rest far from the sphere, that the system is at steady state, with laminar flow and symmetry about the vertical axis (/=0). Beginning with the appropriate form of the Navier-Stokes equations and neglecting gravity derive a differential equation for the velocity profile (, ) in the liquid when the rotation is slow enough so that centrifugal forces are negligible and the flow is fully developed and that gravity is negligible. You do not need to completely solve for (, ), simply begin with the appropriate equation, eliminate the necessary terms and then write a set of boundary conditions for the differential equation.
A sphere of radius R rotates steadily about its vertical axis with an angular velocity (rad/sec) in an infinite Newtonian liquid of viscosity . Assume that the liquid is at rest far from the sphere, that the system is at steady state, with laminar flow and symmetry about the vertical axis (/=0). Beginning with the appropriate form of the Navier-Stokes equations and neglecting gravity derive a differential equation for the velocity profile (, ) in the liquid when the rotation is slow enough so that centrifugal forces are negligible and the flow is fully developed and that gravity is negligible. You do not need to completely solve for (, ), simply begin with the appropriate equation, eliminate the necessary terms and then write a set of boundary conditions for the differential equation.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


