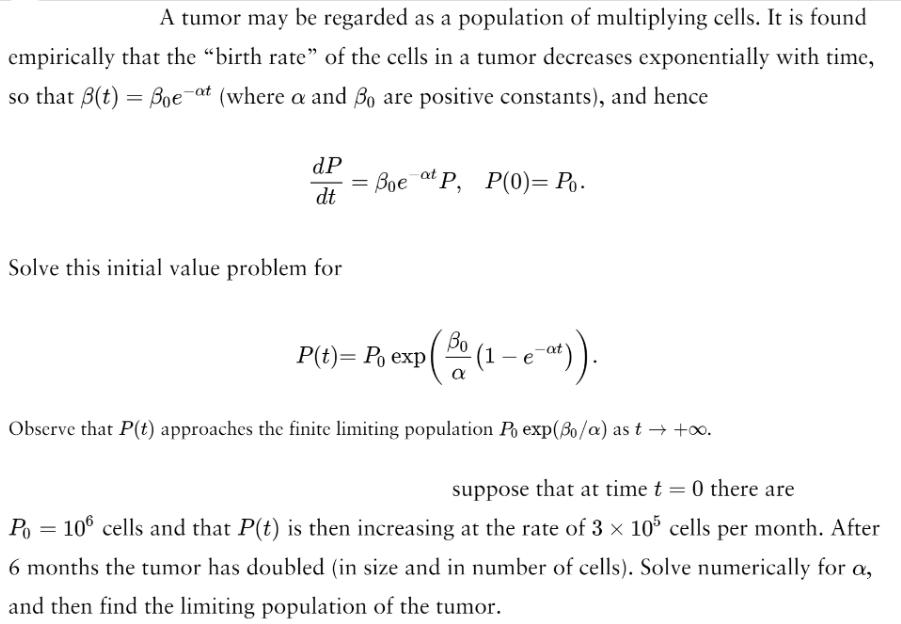
A tumor may be regarded as a population of multiplying cells. It is found empirically that the "birth rate" of the cells in a tumor decreases exponentially with time, so that B(t) = Boe-at (where a and Bo are positive constants), and hence dP dt Solve this initial value problem for = Boe at P, P(0)= Po. P(t)= Po exp (Bo (1-e-a)). Observe that P(t) approaches the finite limiting population Po exp(Bo/a) as t +0. suppose that at time t = 0 there are Po 106 cells and that P(t) is then increasing at the rate of 3 x 105 cells per month. After = 6 months the tumor has doubled (in size and in number of cells). Solve numerically for a, and then find the limiting population of the tumor.