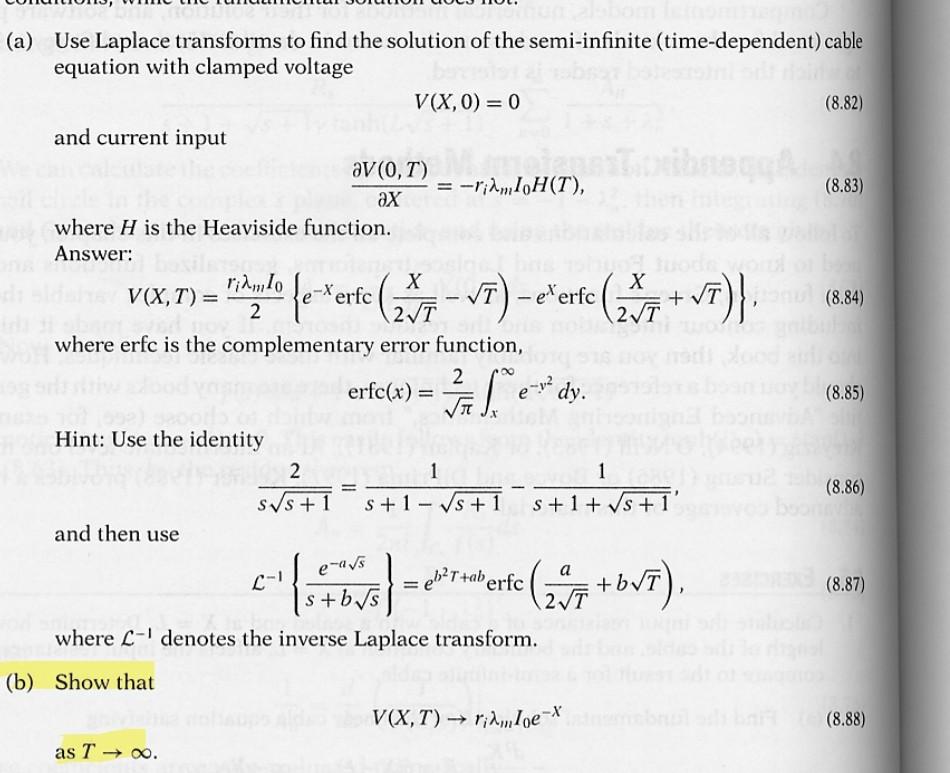
(a) Use Laplace transforms to find the solution of the semi-infinite (time-dependent) cable equation with clamped voltage V(X,0)=0 and current input XV(0,T)=rimI0H(T) where H is the Heaviside function. Answer: V(X,T)=2rimI0{eXerfc(2TXT)eXerfc(2TX+T)}, where erfc is the complementary error function, erfc(x)=2xey2dy. Hint: Use the identity ss+12=s+1s+11s+1+s+11 and then use L1{s+bseas}=eb2T+aberfc(2Ta+bT) where L1 denotes the inverse Laplace transform. (b) Show that V(X,T)rimI0eX asT