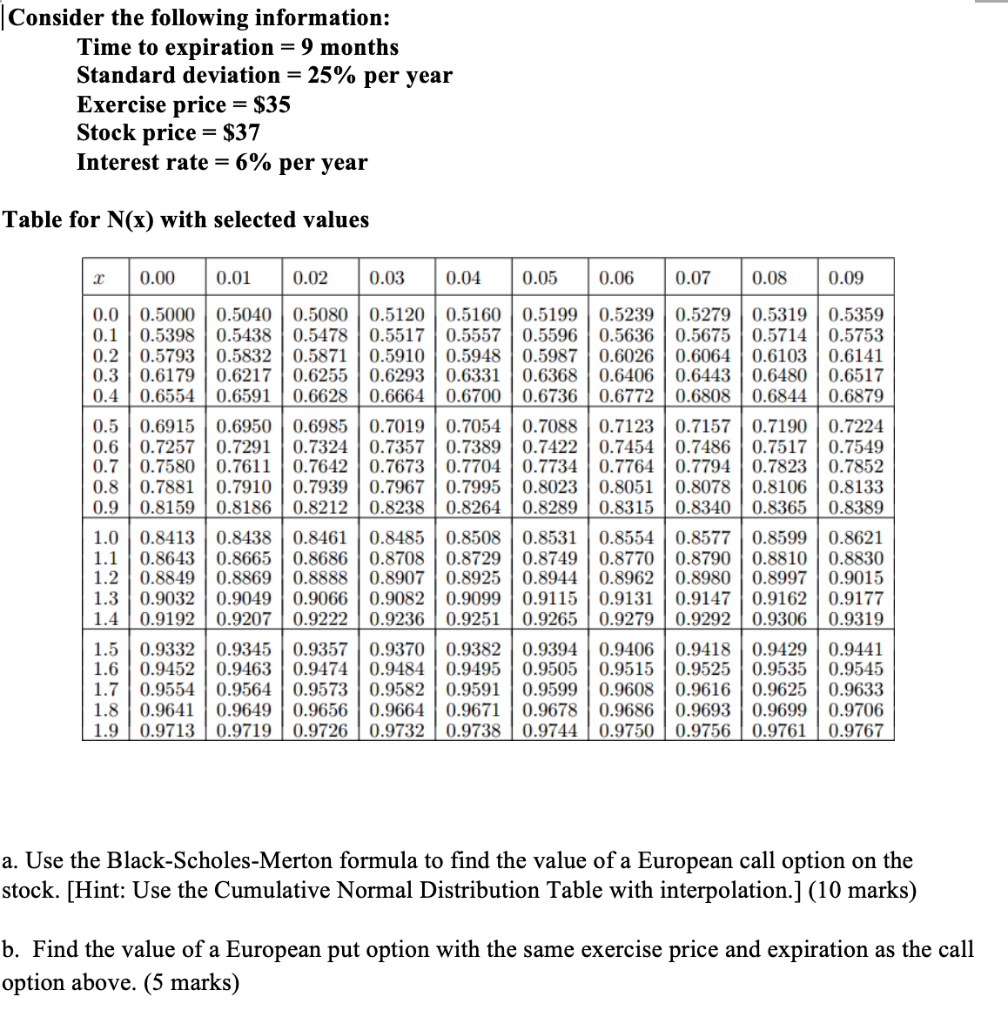
a. Use the Black-Scholes-Merton formula to find the value of a European call option on the stock. [Hint: Use the Cumulative Normal Distribution Table with interpolation.] (10 marks)

b. Find the value of a European put option with the same exercise price and expiration as the call option above. (5 marks)

Consider the following information: Time to expiration = 9 months Standard deviation = 25% per year Exercise price = $35 Stock price = $37 Interest rate = 6% per year Table for N(x) with selected values 0.00 0.01 0.02 0.03 0.04 0.05 0.06 0.07 0.08 0.09 0.0 0.1 0.2 0.3 0.4 0.5000 0.5040 0.50800.5120 0.5160 0.51990.52390.5279 0.5319 0.5398 0.5438 0.5478 | 0.5517 | 0.5557 0.5596 0.5636 0.5675 0.5714 0.5793 0.5832 0.5871 | 0.5910 0.5948 0.5987 | 0.6026 0.6064 0.6103 0.6179 0.6217 | 0.6255 0.6293 0.6331 0.6368 0.6406 0.6443 0.6480 0.6554 | 0.6591 0.6628 0.6664 0.6700 0.6736 0.6772 0.6808 0.6844 0.5359 0.5753 0.6141 0.6517 0.6879 0.5 0.6915 0.6950 0.6985 0.7019 0.7054 0.70880.71230.7157 0.7190 0.7224 0.6 0.7257 0.7291 0.7324 0.7357 0.7389 0.7422 0.7454 0.7486 0.7517 0.7549 0.7 0.7580 0.7611 0.76420.76730.77040.77340.7764 0.77940.78230.7852 0.8 0.7881 0.7910 | 0.7939 0.7967 0.7995 0.8023 0.8051 0.8078 0.8106 0.8133 0.9 0.8159 0.8186 0.8212 0.8238 0.8264 0.8289 0.8315 0.8340 0.8365 0.8389 1.0 0.8413 0.8438 0.8461 0.8485 0.8508 0.8531 0.8554 0.8577 0.8599 0.8621 1.1 0.8643 0.8665 0.8686 0.8708 0.8729 0.8749 0.8770 0.8790 0.8810 0.8830 1.2 0.8849 0.8869 0.8888 0.8907 0.8925 0.8944 0.89620.89800.8997 0.9015 1.3 0.9032 0.9049 0.9066 0.9082 0.9099 0.91150.9131 0.9147 0.9162 0.9177 1.4 0.91920.9207 0.9222 0.92360.9251 0.9265 0.9279 0.92920.9306 0.9319 1.5 0.9332 0.9345 0.9357 0.9370 0.9382 0.9394 0.9406 0.9418 0.9429 0.9441 1.6 0.94520.9463 0.9474 0.94840.9495 0.9505 0.9515 0.9525 0.9535 0.9545 1. 70 .9554 0.95640 .9573 0.95820 .9591 0.9599 0.9608 0.96160.9625 0.9633 1.8 0.9641 0.9649 0.9656 0.9664 0.9671 | 0.9678 0.9686 0.9693 0.9699 0.9706 1.9 0.9713 0.9719 0.9726 0.9732 0.9738 0.9744 0.9750 0.97560.9761 0.9767 a. Use the Black-Scholes-Merton formula to find the value of a European call option on the stock. (Hint: Use the Cumulative Normal Distribution Table with interpolation. (10 marks) b. Find the value of a European put option with the same exercise price and expiration as the call option above