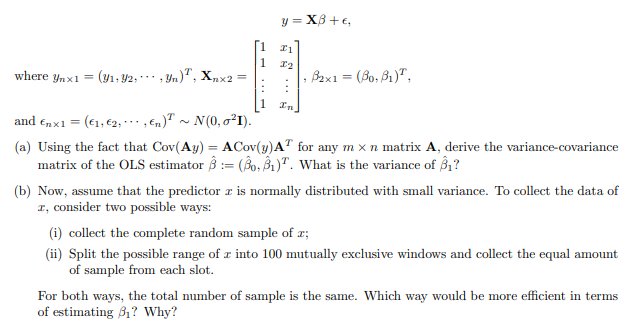
Question
(a) Using the fact that Cov(Ay) = ACov(y)AT for any m n matrix A, derive the variance-covariance matrix of the OLS estimator := ( 0,
(a) Using the fact that Cov(Ay) = ACov(y)AT for any m n matrix A, derive the variance-covariance matrix of the OLS estimator := ( 0, 1) T . What is the variance of 1? (b) Now, assume that the predictor x is normally distributed with small variance. To collect the data of x, consider two possible ways: (i) collect the complete random sample of x; (ii) Split the possible range of x into 100 mutually exclusive windows and collect the equal amount of sample from each slot. For both ways, the total number of sample is the same. Which way would be more efficient in terms of estimating 1? Why?
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


