



AAnwer written works act 1 A and B only all information are givem zoom if blurred
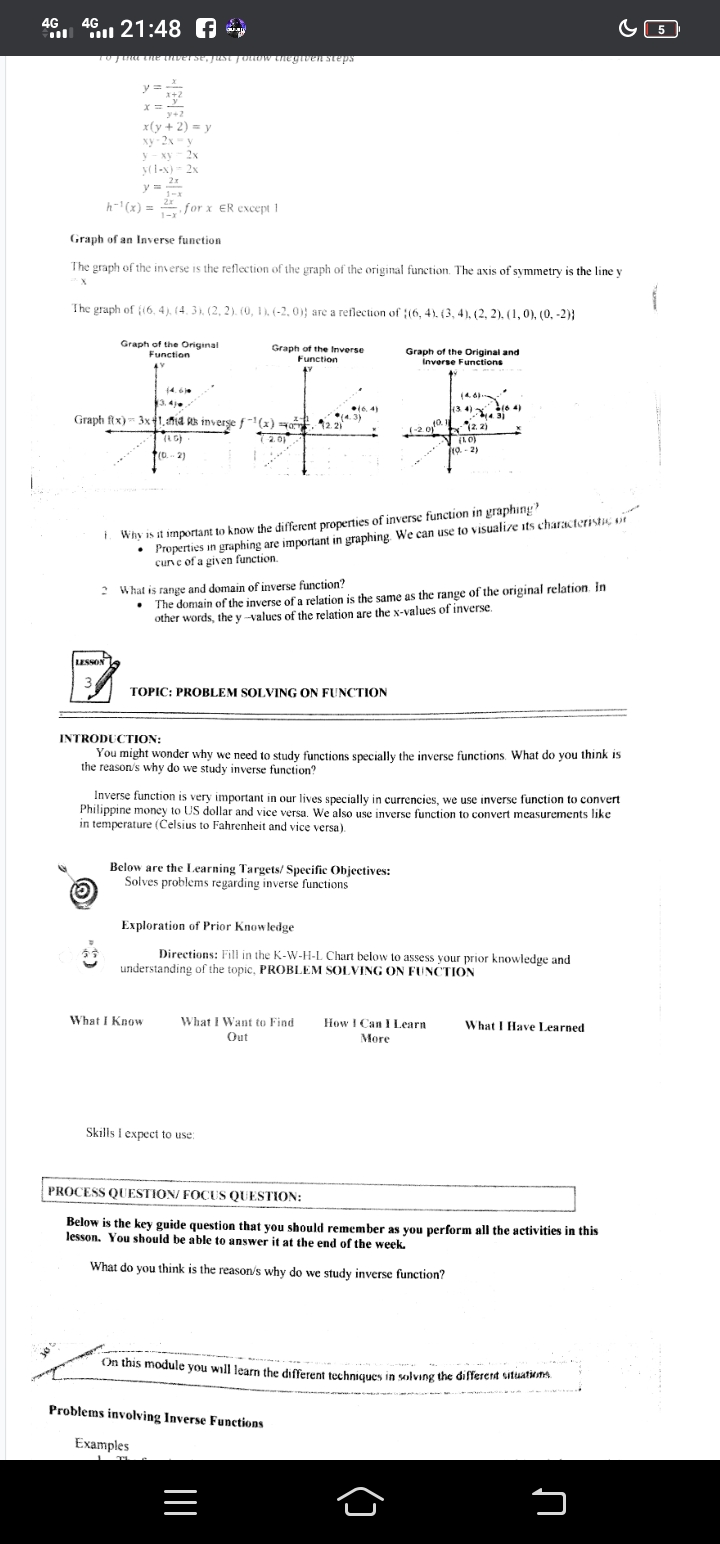
4G in 21:47 f- read the comments section and answer this ; Written Work/s: Activity 1 A. Tell whether the given situation is a one-to-one function or not. Write your answer before the number. 1. Teachers to students 2. Mother and her child 3. Student with his school ID 4. A person with his cellular phone 5. A cellular phone and sim card B. Draw the graph of the function and its invers using the same set of axes. Label the graphs. Then, determine whether the inverse function is also a function or not. 1. f(x) = + 4 2. f (x) = = explanation before answering; CONTENT DISCUSSION: In this lesson, you will learn how to represent one-to-one function in real-life situation. You will be able to identify how to solve and identify one-to-one functions in different methods. The following real-life situations illustrate important type of function- the one-to-one function: 1. Deoxyribonucleic acid is a molecule that carries most of the genetic structures used in the development and functioning of all known living organisms and microorganisms. It is hereditary materials in the humans and almost all other organisms. Every human being has a unique DNA molecule, and every existing DNA molecule is unique to human being. In other words, no two individual ha exactly the same DNA molecule. 2. DepEd is developing a system of identification for all learners of the Philippines public schools. This is the Learner's Identification Number (LIS) System that aims to provide a unique LIS to every public school learner. Its aim is to ensure that no two LIS is assigned to a Filipino learner, and that no two Filipino learners have the same LIS. 3. One of the primary moral values that is advocated and taught by the Catholic Church is the sanctity of the marriage vow. It aims to promote happy marriage between a living Catholic man and a living Catholic woman who have entered into marriage contract, that is, one man-one woman relationship. Definition of a one-to-one Function . A function fis 1 -to- 1 if no two elements in the domain of f correspond to the same element in the range of f. In other words, each x in the domain has exactly one image in the range. And, no y in the range is the image of more than one x in the domain. A one-to-one function is a function in which for each value of y in the range of f, there is just one value x in the domain of f such that y f(x). . [ is one-to-one if fix;) = ((x2) implies x,"x2 How do we know if the given function is a one-to-one? . If the graph of a function f is known, it is easy to determine if the function is 1 -to- 1 . Use the Horizontal Line Test. If no horizontal line intersects the graph of the function f in more than point, then the function is 1 -to- 1 (a) (b) In the given example, which do you think is a one-to-one function? Why did you say so? Answer: figure a is a one-to-one function while figure b is not a one-to-one function, because if we draw a horizontal line through the graphs. the horizontal line crosses the graph in a once, while the horizontal line crosses the second graph at two points Are linear functions one-to-one? Why did you say so? ANSWER: Because for every value of x, there exists one and only one value for the function fix) Inverse Functions Definition: If f is a one-to-one function, then the inverse of f is denoted by "is the function formed by reversing all the ordered pairs in f Thus, f = ((y. xMix, y) is in / A relation reversing the process performed by any function f(x) is called inverse of fix).4G in 21:47 69 -- Definition: If f is a one-to-one function, then the inverse of f is denoted by "is the function formed by reversing all the ordered pairs in f Thus, f = [(y. xMix, y) is in / A relation reversing the process performed by any function f(x) is called inverse of f(x). This means that the domain of the inverse is the range of the original function and that the range of the function is the domain of the original function Examples: Original function Inverse Function 20 30 50 100 68 86 122 212 68 86 122 212 20 30 50 100 From the definition, the following properties of an inverse function follows Properties of an Inverse function If f inverse function exists. f is a one-to-one function; f is also one-to-one 2. Domain of f - Range of f 3 Range of f - Domain of f Examples: 1. Find the inverse of the function described by the set of ordered pairs [(0. -2). (1, 0), (2. 2). (3. 4), (4. 6); Solution: Which interchange the coordinates of each ordered pair. 1(0. -2). (1, 0). (2, 2). (3, 4), (4, 6); original function 42 ((-2, 0) (0. 1). (2, 2). (4, 3), (6, 4)] Inverse function 2 Find the inverse of the function fix) = 3x +6 Solution: To find the inverse of a function defined by an equation, we interchange the independent variable x and the dependent variable y. We know that f(x) = y; hence, f(x) - 3x +6 y = 3x +6 substitute y to f(x) x o 3y+ 6 interchanging x and y 3y = x-6 solve the new equation in terms of y y = =x-2 Divide both sides of the equation by 3 f-1 = -x-2 Replace y to f'(x) 3. Find the inverse of the function f - ((3, 2), (-3, -1), (-6, 3), (6, -1)] Solution: Interchange the coordinates of each ordered pair, we have f (x) = 1(3. 2). (-1. -3). (3, -6), (-1. 6)} Note: f is not a function, why? Because the first element (-1) is paired/ matched to two second clements (-3) and (6) Rules if Writing the inverse of Functions 1. Replace fix) with y 2. Interchange x and y 3. Solve for the new y from the equation in step 2 4. Replace the new y with f (x) if the inverse is a function REVISED KNOWLEDGE: Actual answer to the process questions/ focus questions. 1. What is one-to-one function? A function fis I-to-1 if no two elements in the domain of f correspond to the same element in the range of f. in other words, each x in the domain has exactly one image in the range. And, no y in the range is the image of more than one x in the domain. 2. How to determine if a function is one-to-one function? An easy way to determine whether a function is a one-to-one function is to use the horizontal line test on the graph of a function. To do this, draw horizontal lines through the graph. If any horizontal line intersects the graph more than once, then the graph does not epresent a one-to-one function. LESSON TOPIC: PROPERTIES AND GRAPH OF INVERSE FUNCTION INTRODUCTION: In mathematics, a property is any characteristics that applies to a given set. As you read this module. you are going to understand different properties of an inverse function, you are given an idea about the use of these. Why should we all know this? In this lesson, these properties will guide us to graph inverse function. Below are the Learning Targets/ Specific Objectives: Represents an inverse function through its. a Makes Table of Values b. Sketches a Cartesian Plane 2. finds the domain and range of an inverse function Exploration of Prior Knowledge Directions: Fill in the K-W-H-L. Chart below to assess your prior knowledge and understanding of the topic, PROPERTIES AND GRAPH OF INVERSE FUNCTION What I Know What I Want to Bim4G 1 21:47 f -H 43 Represents an inverse function through its. a Makes Table of Values b. Sketches a Cartesian Plane 2. finds the domain and range of an inverse function Exploration of Prior Knowledge Directions: Fill in the K-W-H-L Chart below to assess your prior knowledge and understanding of the topic, PROPERTIES AND GRAPH OF INVERSE FUNCTION What I Know What I Want to Find How I Can I Learn What I Have Learned Out More Skills I expect to use PROCESS QUESTIONS/ FOCUS QUESTIONS: Below are the key guide questions that you should remember as you perform all the activities in this lesson, You should be able to answer them at the end of the week. 1. Why is it important to know the different properties of inverse function in graphing? 2. What is range and domain of inverse function CONTENT DISCUSSION: When you are in grade 8, you have already heard range, domain intercepts, table of values. Learning more about these properties will help you to graph inverse function easily. Domain and Range of inverse function Examples. 1. Find the range and the domain of the inverse function f-1(x) = $to Solution To find the range and domain of the inverse function, go back to the original function and then interchange the domain and range of the original function f-1(x) = 145; 5y = x +6 multiply both side of the equation by 5 5x - y +6 interchange y and x 5x - 6-y Add both sides of the equation by -6 y = 5x - 6 f(x) = 5x - 6 Original function The Domain of fix) -5x -6 is the set of all real numbers and the range is also the set of all real numbers. Thus, the domain and range of f -1(x) = Ets is the set of all real numbers. 2. Find the Domain and Range of the luminous 2 Solution: Go back to the original function f*1 (x) = 1 2y = 1-x 2x * 1-y y " -2x +1 Domain: (x/x is real number) Range: [x/x is real number) 3. g(x) = 4x2 g(x) = 4x is a quadratic function, therefore it is not a one-to-one function, so it has no inverse, but restricting its domain where it is one-to-one gives the inverse? We first identify a restriction of its domain for itto become one - to - one function One restriction of the domain of g(x) is ( x/x 2 0;: this means that the range of g"(x) ty/y2 0]. Then, 2(x) 4x y = 4x change g(x) with y 4y interchange x and y divide both sides by 4 extract the square root to both sides therefore. g '(x) = 4. for x 20 Another restriction of the domain of g(x) = 4x is {x/x
















