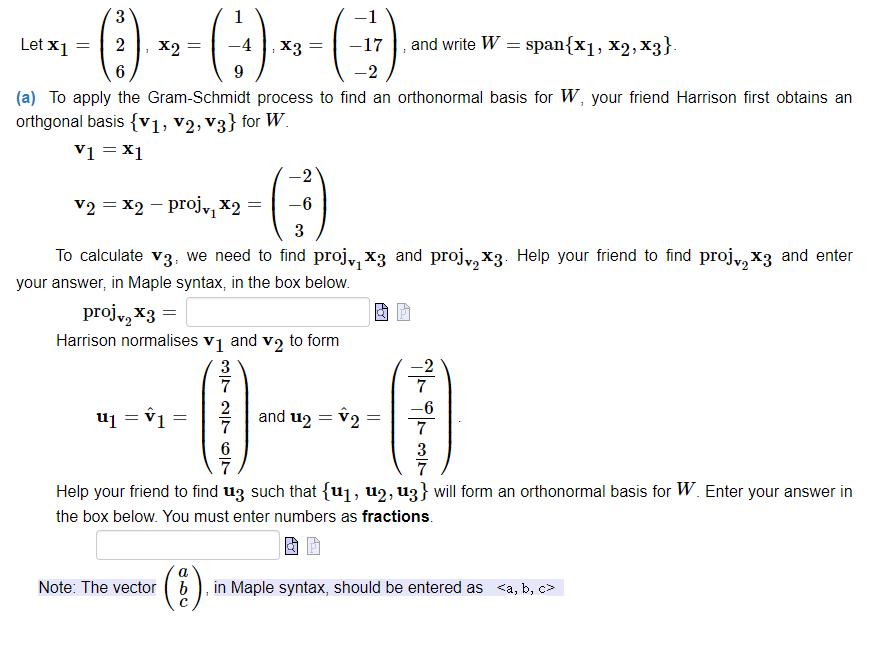
(c) Write down a Jordan form J of the matrix A, i.e. A = PJP-1 for some invertible matrix P and J is a Jordan matrix. (Hint: you should not have to do any calculation for this part.) Write your answer for J, using equation editor, in the box below. 3 2 6 (a) To apply the Gram-Schmidt process to find an orthonormal basis for W, your friend Harrison first obtains an orthgonal basis {V1, V, V3} for W. V1 = x1 Let x1 = X2 = = v2 = x2 - projv X2 1 9 3 27 67 (1) = projvx3 = Harrison normalises v and v to form Note: The vector b X3 = To calculate v3, we need to find proj, X3 and projX3. Help your friend to find projx3 and enter your answer, in Maple syntax, in the box below. -2 () 6 3 7 -0- = -1 -17 and write Wspan{x1, x2, x3}. & and u2 = 2 = 7 Help your friend to find u3 such that {u, 12, 13} will form an orthonormal basis for W. Enter your answer in the box below. You must enter numbers as fractions. 307 in Maple syntax, should be entered as