all one question!
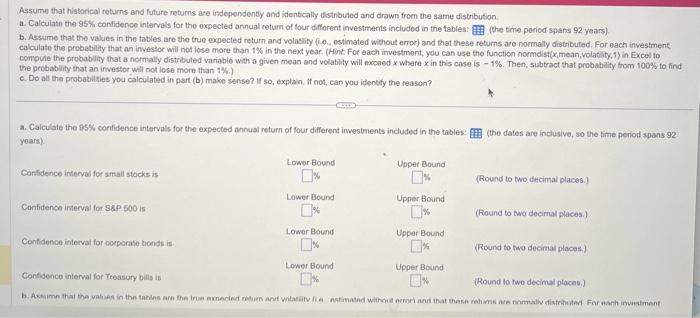
Assume that historical retums and future retums are independently and identically distribuled and drawn from the same distribution. a. Caiculate the 85% confidence intervals for the expocted innual return of four different investments included in the tables: (the time period spans 92 years) b. Assume that the values in the tabies are the true expected return and volatility (1.e, eatinated without error) and that these returns are nomally distributed For each investment. calculate the probability that an investar will not lose more than 1% in the next year. (Hint. For each investment, you can use the function nomitistix,meen, volatity. 1 ) in Excel to compule the probability that a nomelly distributed variable with a given mean and volatility wif erceed x where x in this case is - 1%. Then, subtract thet probability from 100% to find the probability that an investoc will not lose more than 1%.) c. Do all the peotabilities you criculated in part (b) make sense? It so, explain, If not, can you identify the reason? b. Assume that the values in the tabies are the true expected return and volatility (cie. ostimated without efror) and that these retums are normaliy distributed. For each investment. cabculate the probability that an investor will not lase moce than 1% in the next year. (Hint. For eash inbestment, you can use the function normdist (x, mean, volatify, 1 ) in Excel to the probability that an incertuse mitt not lote more than 1%.) The probablity of not losing room than 1% for smail ulocks is V. (Round to two decinal places.) The probabiliny of not losiry more than 1% for the S8P 500 is \%. (Round to two decinal plinote.) The probability of not iosing more than 18 for corpornte bonds is k. (Round to two decimel places.) The probabiliny of not boting more than 1% for Teeasury bilts if W. (Round to twe decinal places) c. De all the probablities you calculated in part ib) make snose? If b0, explain. If not, can you identify the mason? (Seloct the best choise beion) itsiribitent Assume that historical teturns and future returns are independently and identically distributed and drawn from the same distribution. a. Calculate the 95% confidence intervals for the expected annual retum of four different investments included in the tablos: (the time period spans 92 years). b. Assume that the values in the tables are the true expected return and volatifity (1.e., estimated without error) and that these returns are normally distributed. For each investment, calculate the probability that an investor will not lose more than 1% in the next year. (Hint. For oach investment, you can use the function normdist( x,mean, wolatility. 1) in Excel to compute the probablity that a normally distributed variable with a given mean and volatility will exceed x where x in this case is 1%. Then, subtract that probabify from too\% to find the probability that an investor.will not lose more than 1%.) c. Do all the probabilities you calculated in part (b) make sense? If so, oxplain, If not, can you identify the reason? The probability of not losing more than 1% for the SsP 500 is V. (Round to two docimal placos.) The probability of not losing more than 14 for corporate bonds is N. (Round to two decimal places.) The probablity of not losing more than 1W for Treasury bills is 16. (Round to two decimat placen) c. Do ail the probabilities you calculated in part (b) make sense? If so, explain. If not, can you identify the reason? (Select the best choice below) Gistrituted. B. Yes, Alt these imvestments involve risk, and so you expect to lase money some of the time. C. No. You cannot iose money on Treasury bilis. The problem is that the retums on Treasury bils aro not normally distributed. D. No. The probability that you can have a retum on small stocks less than 1% is not nearly as high as 69.24%. The probiem is that the cetum of snall stocks are not normally distributed Data table (Click on the following icon D in order to copy its contents into a spreadsheet.) Average Annual Returns for U.S. Small Stocks, Larqe Stocks (S\&.P 500). (Click on the following icon p in order to copy its contents into a spreadsheet) the dates are inclusive, so th Assume that historical returns and future retums are independenty and identically distributed and drawn from the same distribution. a. Calculate the 95% confidence intervals for tho oxpected armual return of four difforent investments included in the tables: (the trme period spans 92 years). b. Assume that the values in the tables are the true expected return and volatily (0.0., estimated without erroc) and that these returns are normally distributed. For each investment. calculate the probability that an imvestor will not lose more than 1% in the next year. (Hint. For each irvestment, you can use the function normdist( x, mean, volatily, 1 ) in Excel to compule the probablity that a normally distrbuted variablio with a given mean and volatility will exceed x where x in this case is - 1%. Then, subtract that probahilify trom 100%, to find the probability that an investor will not lose more than 1%.) c. Do alt the probabiltsles you calculated in part (b) make sense? If so, explain. If not, can you identify the reason? a. Calculate the 95% confidence intervals for the expected annual return of four different investments included in the tables: (the dates are inclusive, so the time period spans 92











