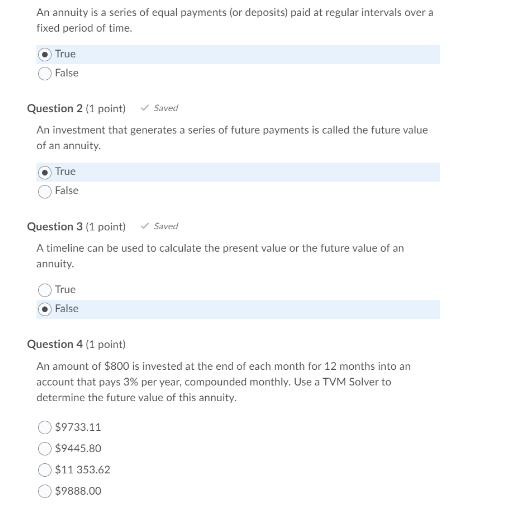
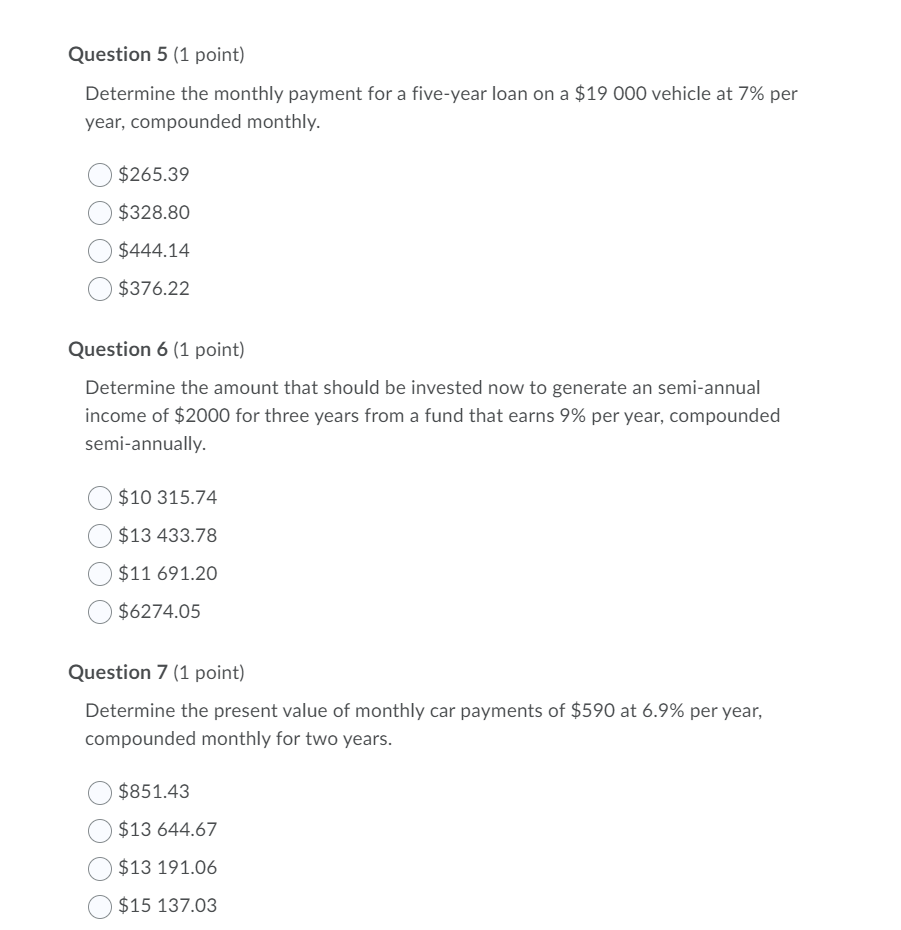
An annuity is a series of equal payments (or deposits) paid at regular intervals over a fixed period of time. True False Question 2 (1 point) Saved An investment that generates a series of future payments is called the future value of an annuity. True False Question 3 (1 point) Saved A timeline can be used to calculate the present value or the future value of an annuity. True False Question 4 (1 point) An amount of $800 is invested at the end of each month for 12 months into an account that pays 3% per year, compounded monthly. Use a TVM Solver to determine the future value of this annuity. $9733.11 $9445.80 $11 353.62 $9888.00 Question 5 (1 point) Determine the monthly payment for a five-year loan on a $19 000 vehicle at 7% per year, compounded monthly. $265.39 $328.80 $444.14 $376.22 Question 6 (1 point) Determine the amount that should be invested now to generate an semi-annual income of $2000 for three years from a fund that earns 9% per year, compounded semi-annually. $10 315.74 $13 433.78 $11 691.20 $6274.05 Question 7 (1 point) Determine the present value of monthly car payments of $590 at 6.9% per year, compounded monthly for two years. $851.43 $13 644.67 $13 191.06 $15 137.03 Question 8 (1 point) Hayden recently graduated from college and owes $24 000 on a student loan that she must begin to repay. Payments are to be made at the end of each month for the next five years. Interest is 8.5% per year, compounded monthly. Calculate the total amount needed to repay the loan. $24 512.40 $25 119.24 $29 544.00 $19 344.00 Question 9 (1 point) Maya would like to borrow $4500. Her bank offered her a two-year personal loan with monthly payments. The interest rate on the loan is 8% per year, compounded monthly. How much interest will Maya pay over the life of the loan? $219.81 $384.48 $197.40 $778.00 Question 10 (1 point) Katie has a part-time job at a local convenience store and earns $700 per month. She plans to deposit $350 at the end of each month into an account that pays 3.75% per year, compounded monthly. How much will be in Katie's account after three years? $26 628.22 $17 766.86 $11 899.54 $13 314.11 $384.48 $197.40 $778.00 Question 10 (1 point) Katie has a part-time job at a local convenience store and earns $700 per month. She plans to deposit $350 at the end of each month into an account that pays 3.75% per year, compounded monthly. How much will be in Katie's account after three years? $26 628.22 $17 766.86 $11 899.54 $13 314.11 Question 11 (1 point) What amount should be invested now at 6% per year, compounded annually, to generate a retirement income of $35 000 per year for 15 years? $339 929 $504 582 $814 659 $543 779 Question 1 (1 point) Determine the monthly payment on $7500 borrowed at 9.6% per year, compounded monthly for one year and for five years. What is the difference between the two monthly payments? approximately $500 approximately $200 approximately $400 approximately $100 Question 2 (1 point) Calculate the total interest paid on $1800 borrowed at 8.4% per year, compounded monthly, for one year and for five years. What is the difference between the total amount of interest paid on the two loans? approximately $525 approximately $425 approximately $325 approximately $780 Question 3 (1 point) Mallory began saving $100 per month 15 years ago. Her savings have earned 7% per year, compounded monthly. Edward believes that if he saves $200 per month in an account paying 14% interest per year, compounded monthly, he will have as much as Mallory in 15 years. Which statement best describes the value of each investment at the end of 15 years (assume Mallory continues to contribute throughout the next 15 years)? Edward's investment will be worth more than Mallory's investment. Edward's investment will be worth less than Mallory's investment. Edward's investment will be worth the same as Mallory's investment. More information is needed to determine the value of each investment in 15 years. Question 2 (1 point) A mortgage is an annuity where the future value is the amount borrowed to purchase a home. True False Question 3 (1 point) Determine the monthly payment for a five-year fixed rate mortgage of $260 000 amortized over 25 years at an annual interest rate of 6.49%. $1753.92 $922.92 $1739.97 $1723.93 Question 4 (1 point) Calculate the total amount paid over the term of a five-year fixed rate mortgage of $260 000 amortized over 25 years at an annual interest rate of 6.49%. $105 235.20 $106 072.20 $55 375.20 $104 398.20