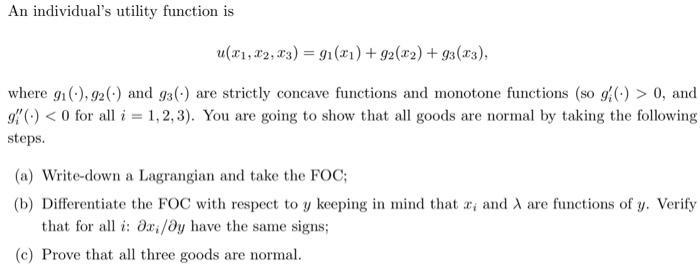
An individual's utility function is u(x1, x2, x3)=91(x1)+92(x2) + 93(x3), where 91(), 92() and 93(-) are strictly concave functions and monotone functions (so g'() > 0, and g() < 0 for all i=1,2,3). You are going to show that all goods are normal by taking the following steps. (a) Write-down a Lagrangian and take the FOC; (b) Differentiate the FOC with respect to y keeping in mind that x; and A are functions of y. Verify that for all i: Ox; /Oy have the same signs; (c) Prove that all three goods are normal.