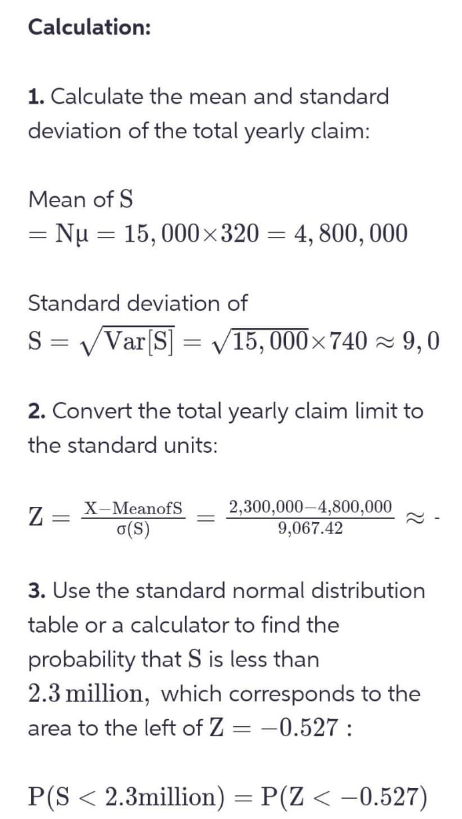
An insurance company has 15,000 life insurance policy holders. If the yearly claim of a policy holder is a random variable with mean 320 and standard deviation 740, what is the probability that total yearly claim will less than 2.3 million. Given: Number of life insurance policy holders, N = 15,000. Mean yearly claim of a policy holder, = 320. Standard deviation of yearly claim, = 740. Total yearly claim limit, X = 2.3 million. To Find: Probability that the total yearly claim will be less than 2.3 million. Concept/Formula Used: N Total yearly claim, S = , where X; is the yearly claim of the ith policy holder. Mean of total yearly claim, E[S] = N. Variance of total yearly claim, Var[S] = No. Standard deviation of total yearly claim, (S) = Var[S]. Standardizing the value using the standard normal distribution, Z X-E[S] (S) Probability calculation using the standard normal distribution table or calculator. Explanation: With a mean claim of 320 and a standard variation of 740, the issue affects 15,000 policyholders. Using a conventional normal distribution, are to determine the likelihood that the total annual claim, represented as a sum, is less than 2.3 million. Calculation: 1. Calculate the mean and standard deviation of the total yearly claim: Mean of S = Nu = 15,000320 = 4,800,000 Standard deviation of S = Var[S] = 15,000740 9,0 2. Convert the total yearly claim limit to the standard units: Z X-Meanofs = = (S) 2,300,000-4,800,000 9,067.42 3. Use the standard normal distribution table or a calculator to find the probability that S is less than 2.3 million, which corresponds to the area to the left of Z = -0.527: P(S2.3million) = P(Z < -0.527)