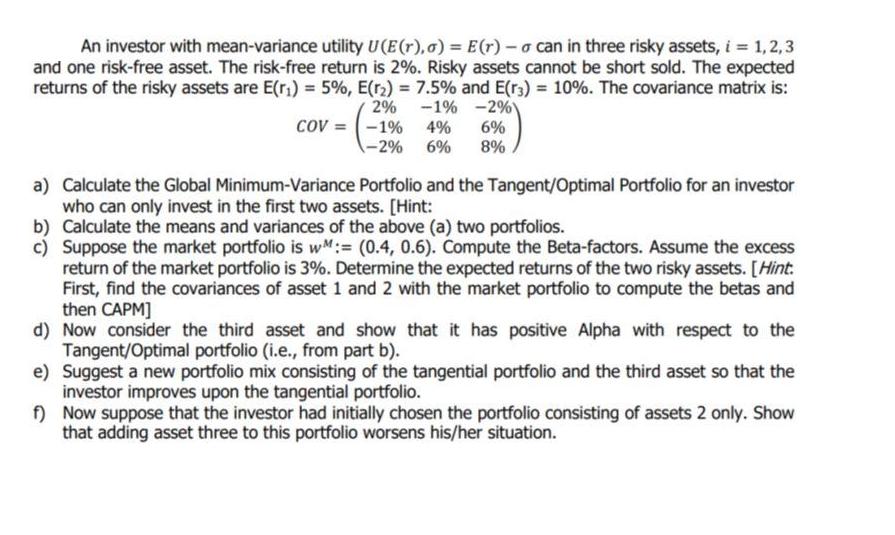
An investor with mean-variance utility U(E(r), o) = E(r)-o can in three risky assets, i = 1,2,3 and one risk-free asset. The risk-free return is 2%. Risky assets cannot be short sold. The expected returns of the risky assets are E(r) = 5%, E(r) = 7.5% and E(r3) = 10%. The covariance matrix is: 2% -1% COV=-1% -2% -2% 6% 4% 6% 8% a) Calculate the Global Minimum-Variance Portfolio and the Tangent/Optimal Portfolio for an investor who can only invest in the first two assets. [Hint: b) Calculate the means and variances of the above (a) two portfolios. c) Suppose the market portfolio is wM:= (0.4, 0.6). Compute the Beta-factors. Assume the excess return of the market portfolio is 3%. Determine the expected returns of the two risky assets. [Hint. First, find the covariances of asset 1 and 2 with the market portfolio to compute the betas and then CAPM] d) Now consider the third asset and show that it has positive Alpha with respect to the Tangent/Optimal portfolio (i.e., from part b). e) Suggest a new portfolio mix consisting of the tangential portfolio and the third asset so that the investor improves upon the tangential portfolio. f) Now suppose that the investor had initially chosen the portfolio consisting of assets 2 only. Show that adding asset three to this portfolio worsens his/her situation.