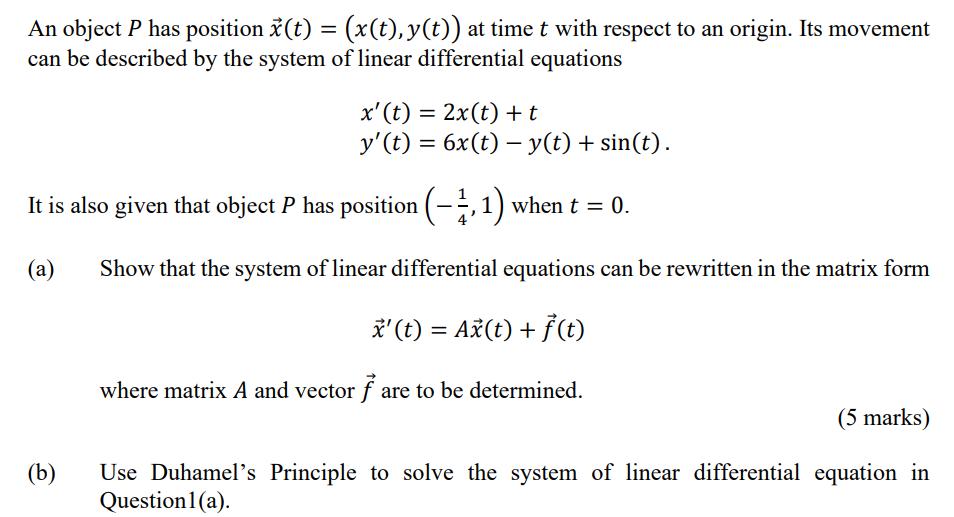
An object P has position x(t) = (x(t), y(t)) at time t with respect to an origin. Its movement can be described by the system of linear differential equations x'(t) = 2x(t) +t y' (t) = 6x(t)- y(t) + sin(t). It is also given that object P has position (-1, 1) when t = 0. (a) Show that the system of linear differential equations can be rewritten in the matrix form x' (t) = Ax(t) + f(t) where matrix A and vector f are to be determined. (b) (5 marks) Use Duhamel's Principle to solve the system of linear differential equation in Question1(a).