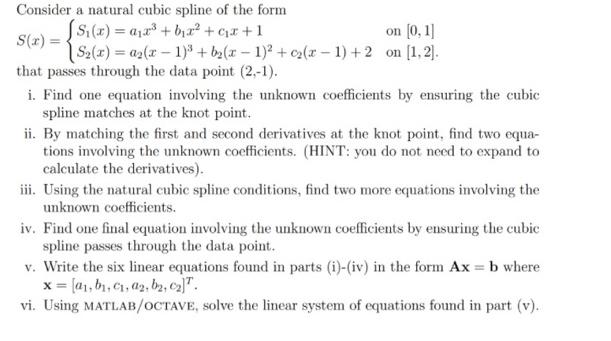
Consider a natural cubic spline of the form JS(x)= a2 + bx+x+1 on [0, 1] S(x) = S(x)=a(x-1) + b(x - 1) +0(x-1) +2 on [1,2]. that passes through the data point (2,-1). i. Find one equation involving the unknown coefficients by ensuring the cubic spline matches at the knot point. ii. By matching the first and second derivatives at the knot point, find two equa- tions involving the unknown coefficients. (HINT: you do not need to expand to calculate the derivatives). iii. Using the natural cubic spline conditions, find two more equations involving the unknown coefficients. iv. Find one final equation involving the unknown coefficients by ensuring the cubic spline passes through the data point. v. Write the six linear equations found in parts (i)-(iv) in the form Ax = b where x= [a, b, c, a2, b2, 0]. vi. Using MATLAB/OCTAVE, solve the linear system of equations found in part (v).