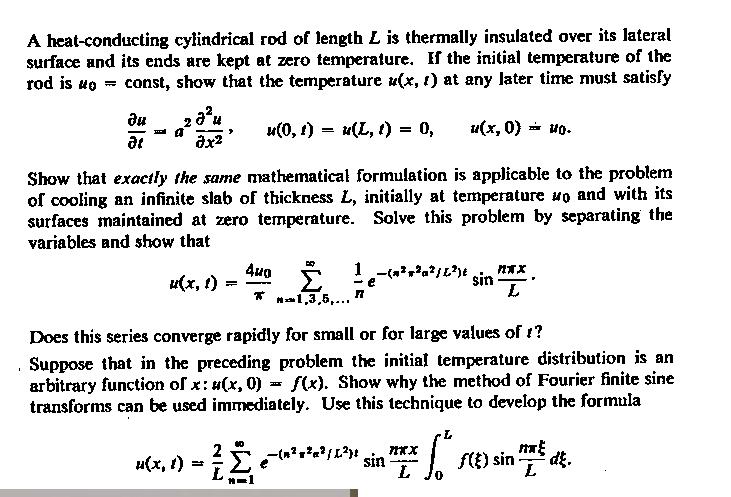
A heat-conducting cylindrical rod of length L is thermally insulated over its lateral surface and its ends are kept at zero temperature. If the initial temperature of the rod is uo const, show that the temperature z(x, 1) at any later time must satisfy u(0, 1) = u(L, 1) = 0, u(x, 0) #o. Show that exactly the same mathematical formulation is applicable to the problem of cooling an infinite slab of thickness L, initially at temperature wo and with its surfaces maintained at zero temperature. Solve this problem by separating the variables and show that = t 2 2 Ju x u(x, t) u(x, t) 4ug = -- T = D 1 -(xa/L) nxx sin L A 1,3,5,... Does this series converge rapidly for small or for large values of !? Suppose that in the preceding problem the initial temperature distribution is an arbitrary function of x: u(x, 0) = f(x). Show why the method of Fourier finite sine transforms can be used immediately. Use this technique to develop the formula 12.. P [, f(t) sin de. n L XXX -(nx/1) sin L