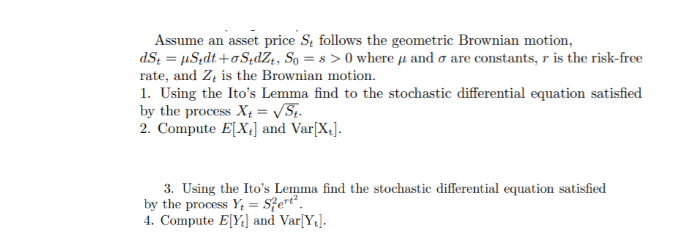
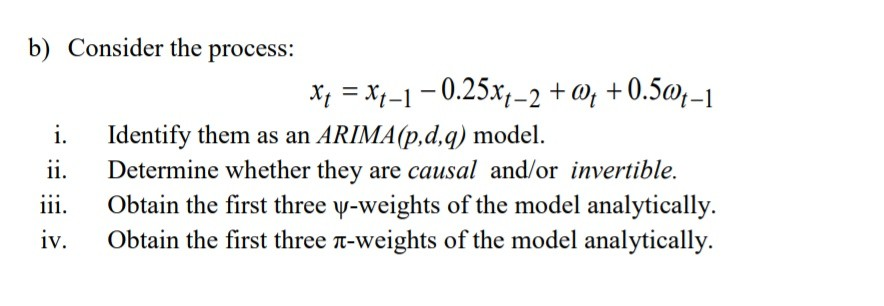
Another use of the transition matrix is in stochastic modeling. An example is the following: Imagine a park with three locations: a lake; a picnic area; and a playground. Every hour, on the hour, the parkgoers move according to the following rules: . Half of those at the lake move to the picnic area, and one-quarter of those at the lake move to the playground. Half of those at the picnic area go to the lake, and the other half go to the playground. . Half of those at the playground go to the picnic area. Once they arrive, the parkgoers stay until the next hour, at which point they move again, according to the same rules. Assume an asset price S, follows the geometric Brownian motion, dS = S+dt+SdZt, So=8>0 where and are constants, r is the risk-free rate, and Z is the Brownian motion. 1. Using the Ito's Lemma find to the stochastic differential equation satisfied by the process X = St. 2. Compute E[X+] and Var[X+]. 3. Using the Ito's Lemma find the stochastic differential equation satisfied by the process Y Sert = 4. Compute E[Y] and Var[Y]. b) Consider the process: i. 11. 111. iv. x = xt-1 -0.25x-2 + @ +0.5@-1 Identify them as an ARIMA (p,d,q) model. Determine whether they are causal and/or invertible. Obtain the first three y-weights of the model analytically. Obtain the first three -weights of the model analytically.