

Answer all questions .,,,
1. A perfectly competitive firm produces output q with capital K and labor Laccording to the production
function: 9 = f(K,L)= 4K "L/4)*L "1/4) Ihe price of labor is w = 4, and the price of capital is r= 4. Ihe firm
has fixed costs equal to 8. Ihe current market price is 8.
(a) Prove that this production function has decreasing returns to scale.
(6) Find the optimal (cost-minimizing) ratio of capital to labor inputs K/L for any level of output (use scale
expansion path).
(C)For any q, find the cost minimizing inputs as functions of output: 1e, derive functions L[q) and K[a).
(d) Find cost functions TC(q), ATClg), and MC(g), i.e. as functions of output. At what value of q does the
minimum of ATC occur?
(e) Find total and marginal revenue as functions of output, i.e. TR{q) and MR[q).
) Find the profit maximizing output q and use that to find TR{q-), TCq-), Trq*), Liq), and Kiq).
g) Will other firms have an incentive to enter or exit this market? What will be the new market price that
results from the entry/exit of firms? At the new price, what is the firms new Output? What is the firms
profit?
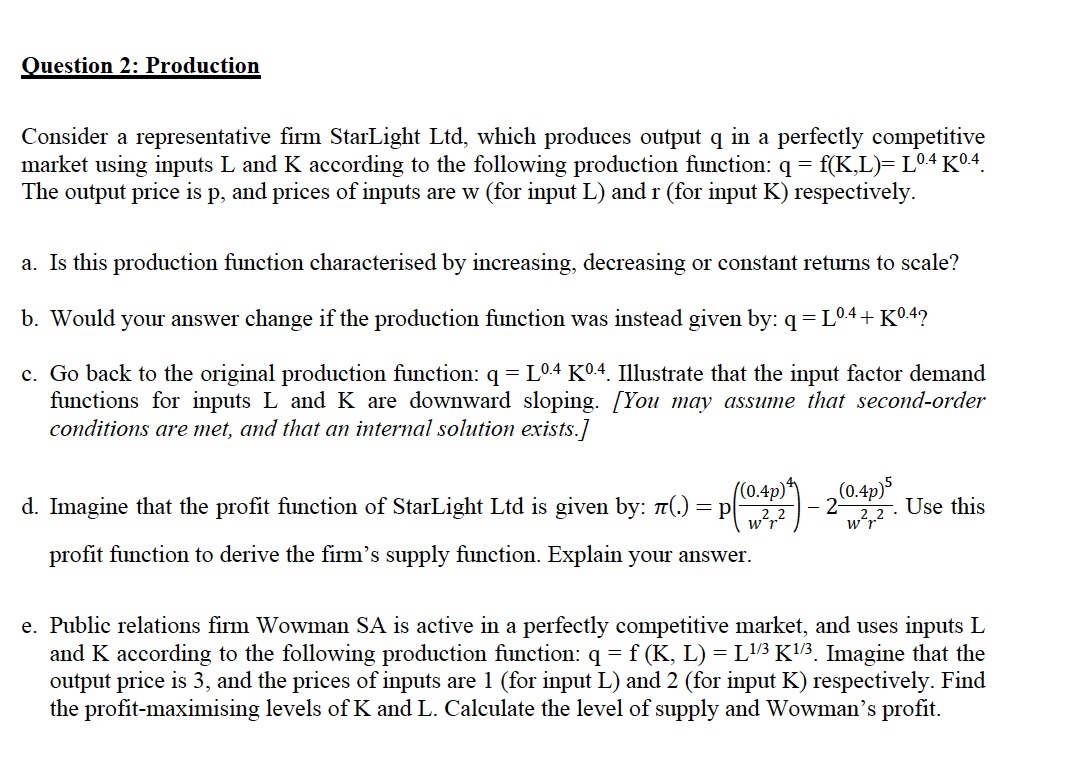
Question 2: Production Consider a representative rm StarLight Ltd, which produces output q in a perfectly competitive market using inputs L and K according to the following production function: q = f(K,L)= L\"4 K\". The output price is p, and prices of inputs are w (for input L) and r (for input K) respectively. a. Is this production function characterised by increasing, decreasing or constant returns to scale? b. Would your answer change if the production function was instead given by: q = L\"4 + K\"? c. Go back to the original production function: q = L'M K04. Illustrate that the input factor demand functions for inputs L and K are downward sloping. [You may assume that second-order conditions are met, and that an internal solution exists] (0.419?) _ (0.41305 d. Imagine that the profit function of StarLight Ltd is given by: n(.) = p( 2 2 2 . Use this W T 22 WT prot function to derive the rm's supply function. Explain your answer. e. Public relations rm Wowman SA is active in a perfectly competitive market, and uses inputs L and K according to the following production function: q = f (K, L) = L\"3 K13. Imagine that the output price is 3, and the prices of inputs are 1 (for input L) and 2 (for input K) respectively. Find the profit-maximising levels of K and L. Calculate the level of supply and Wowman's profit. Consider a firm with production function F( L, K) = min {AL, 5K) . Input prices are W = 1, R= 5. a) Does this function have constant, increasing or decreasing returns to scale? /constant/increasing/ decreasing/ Why? (show with math) (5) b) What is the cost-minimizing bundle of inputs that the firm can use to produce Q = 407 umbers for L. K / /5/ c) The firm incurs an additional avoidable fired cost of 40 to start production. What are the total, average and marginal cost functions? fequation/ 15/Question 1: Suppose someone offers you the opportunity to play the following game. This person will toss a fair coin repeatedly until heads appear. If this happens on the very first toss, you get $2. If on the second (that is, tails on the first toss is followed by heads on the second toss), you get $4. And so on; if (n - 1) tails in succession are followed by heads on the noth toss, you get $2" . a ) Calculate the mathematical expectation of your (random variable) winnings W. (b) What sure sum would you be willing to pay in advance to be given the opportunity of participating in this game? (c) Suppose your von Neumann-Morgenstern utility function is vW. Calculate your expected utility. What sure sum, if offered to you instead of the game, would give you the same utility? (d) Suppose your von Neumann-Morgenstern utility function is In W. Calculate your expected utility. What sure sum, if offered to you instead of the game, would give you the same utility? (e) Suppose your von Neumann-Morgenstern utility function is - 1/W. Calculate your expected utility. What sure sum, if offered to you instead of the game, would give you the same utility? (f) What is the coefficient of relative risk aversion for each of the utility functions in (c)-(e)? Question 2: Consider the following simplified version of "Who Wants To Be A Millionaire". You have reached the $32,000 level. (Any Princeton student should be able to do that.) Now you face a succession of questions, each with two possible answers. If you answer a question correctly, whether because you know the correct answer or because you make a lucky guess, you proceed to the next higher prize level. We call your first question (the one that if correctly answered takes you to the $64,000 level) "the $64,000 question" for short; similar abbreviations apply to the following levels. The prize doubles at each level. If you answer the $64,000 question correctly and so reach the $64,000 level, you face the $128,000 question, and so on to $256,000, and $512,000. If you reach the $512,000 level, you face one final question, and the correct answer to it will win you $1,024,000. At any level, when you get the question that can take you to the next level, you may choose not to answer it, and leave with the prize of the level you have already reached. At any level, if you answer the next question and your answer is wrong, you will leave with only $32,000. At each level, before you have seen the question that can take you to the next level, there is a probability that you know the correct answer. The questions get successively harder, so these probabilities decline from one level to the next. Before you see the $64000 question, the probability that you know the answer is 40 percent. If you answer this question successfully to reach the $64,000 level, and before you see the $128,000 question, the probability that you know the answer to that is 35 percent. Similarly, the probabilities are 30 percent for the $256,000 question, 25 percent for the $512,000 question, and 20 percent for the $1,024,000 question. At each level, once you have seen the question, you will know for sure whether you know the correct answer. Thus there is no possibility that you are confident but wrong, or that you know the correct answer but fail to realize that you know. At each level, after you see the question, if you know the correct answer, of course you will give it. If you don't, you have to decide whether to make a guess, which at any level has a 50 percent chance of being correct, or to walk, that is, leave with the amount of the level you have reached. Remember that if you choose to guess and are lucky, you proceed to the next level, but if you choose to guess and are unlucky, you will have to leave with only $32,000, no matter what level you had reached. Your von Neumann-Morgenstern utility function is log, (W/32000), where W is the amount of dollars of your prize. (Note that logs are to base 2, not 10 or e.) (a ) What are the utility numbers corresponding to the various possible levels of prizes? (b) Find your optimal strategy, namely your plan of action that prescribes, at each level, whether to make a guess if you don't know the answer to the question, or to walk with the prize of the level you have reached. You have to begin at the end (where you have already reached the $512000 level) and work your way backward. At each stage you will find it useful to draw a mini "decision tree" like the one shown here, with the appropriate probability and utility values filled where ... is shown: Know; Probability = ... Utility = ... Utility = ... Walk Don't know; Right Probability = ... Probability 0.5 Utility = ... Guess Wrong; Probability 0.5 Utility = ... (c) What is your expected utility at the initial situation where you have reached the $32,00 level and are about to receive the $64,000 question? What amount of sure dollars would give you the same utility












