ANSWER ALL QUESTIONS! SHOW ALL WORK! THANK YOU! I will leave an amazing review afterwards :)
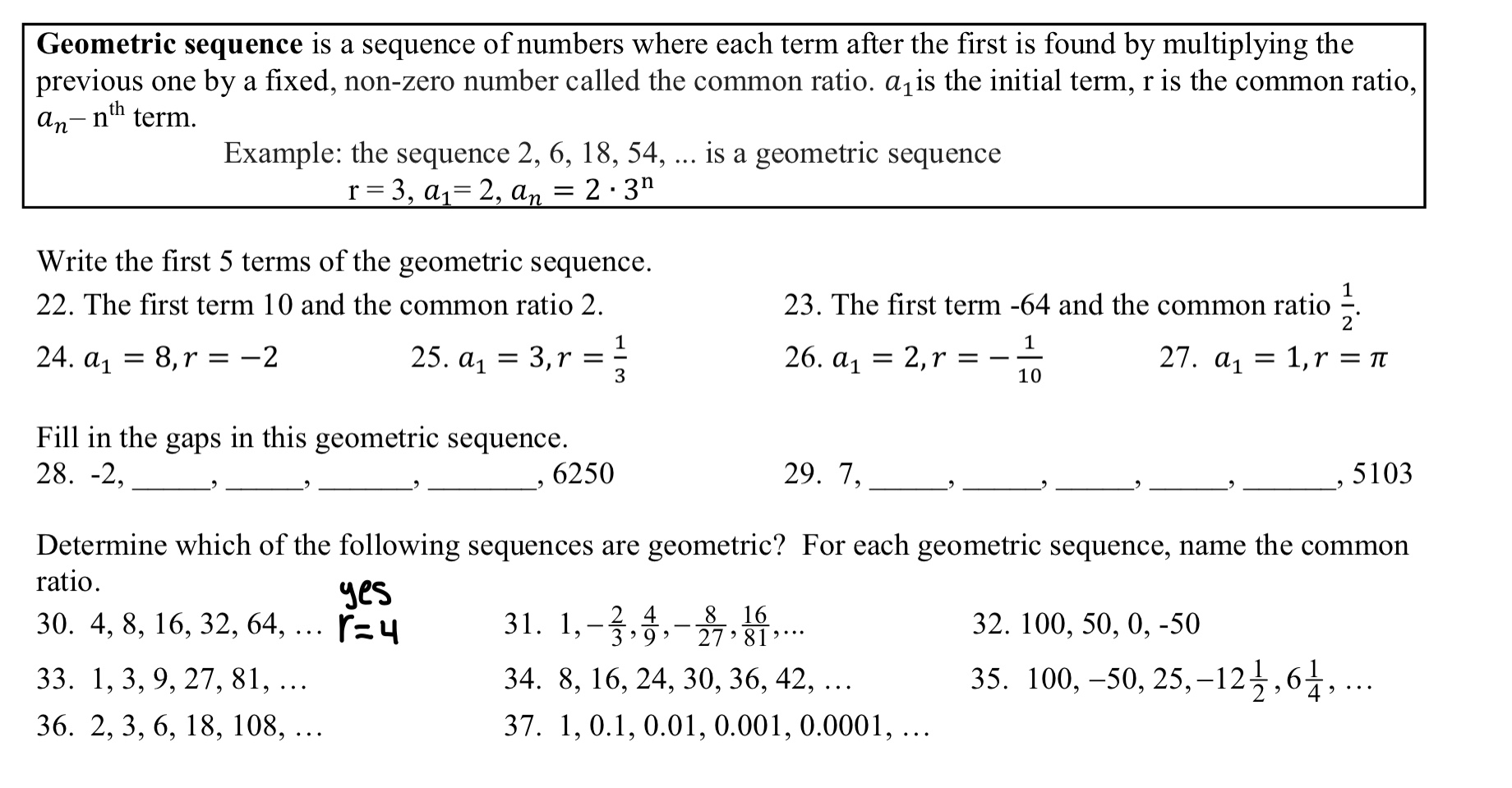
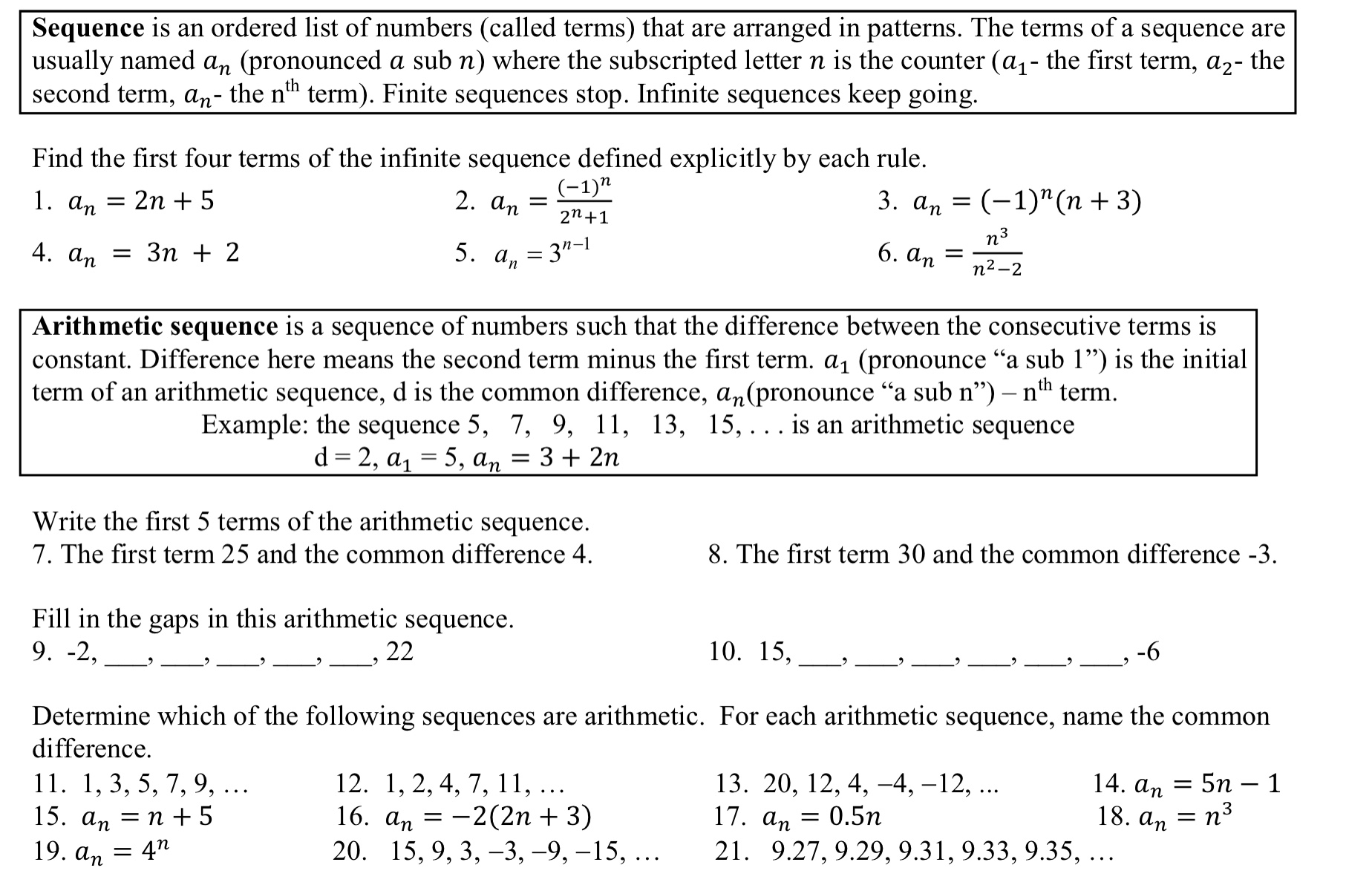
An Explicit Formula for a sequence states the nth term (a general term) as a function of n. Example: an = 3n-1 Example: a, = 4" Find an explicit formula for each sequence. 38. 3,7,11,15, 19, . .. 39. 1,1 1 1 1 ... 40. IN 4 8 4' 9' 16'25' 9'27 81 1 .. 41. 2,1, - 5 6 7 ' 5' 7' 9' 11' 42. 1, 4, 9, 16, 25, ... 43. 1, 7, 4, 8' 16,... The general term an of an arithmetic sequence with common difference d may be expressed explicitly as an = a1 + d(n -1) Write the explicit formula for the nth term. 44. a] = 5, d = 7 45. a1 = -3, d = -2 46. a, = 1.5, d = -.2 Find an explicit formula for the sequence and use it to find the 16th term. 47. 62, 64, 66, 68, 70, ... 48. 15, 9, 3, -3, -9, -15, ... 49. Find the 102nd term of the sequence 5, 13, 21, 29, 37, 45, ... Find an explicit formula for the general term of the arithmetic sequence with the given terms. 50. as = 24 and a9 = 40 51. a3 = 14 and a6 = 29 52. a7= -10 and a13 = -46 53. a4=-4 and a10 =-22Geometric sequence is a sequence of numbers where each term after the first is found by multiplying the previous one by a fixed, non-zero number called the common ratio. a, is the initial term, r is the common ratio, an- nt term. Example: the sequence 2, 6, 18, 54, ... is a geometric sequence r = 3, a1= 2, an = 2 . 30 Write the first 5 terms of the geometric sequence. 22. The first term 10 and the common ratio 2. 23. The first term -64 and the common ratio NIK 24. a1 = 8,r = -2 25. a1 = 3,r = 26. a1 = 2,r = - 1 10 27. a1 = 1, r = n Fill in the gaps in this geometric sequence. 28. -2, , 6250 29. 7, 5103 Determine which of the following sequences are geometric? For each geometric sequence, name the common ratio. yes 30. 4, 8, 16, 32, 64, ... = 4 31. 1, -2 4 _ 8 16 3'9' -27, 81,." 32. 100, 50, 0, -50 33. 1, 3, 9, 27, 81, ... 34. 8, 16, 24, 30, 36, 42, ... 35. 100, -50, 25, -12- , 64. 4 , ... 36. 2, 3, 6, 18, 108, ... 37. 1, 0.1, 0.01, 0.001, 0.0001, ...Sequence is an ordered list of numbers (called terms) that are arranged in patterns. The terms of a sequence are usually named an (pronounced a sub n) where the subscripted letter n is the counter (a,- the first term, a2- the second term, an- the n" term). Finite sequences stop. Infinite sequences keep going. Find the first four terms of the infinite sequence defined explicitly by each rule. 1. an = 2n + 5 2. an = - (-1)n 2n + 1 3. an = (-1)" (n + 3) 4. an = 3n + 2 5. a, = 3"-1 6. an = n3 n 2 - 2 Arithmetic sequence is a sequence of numbers such that the difference between the consecutive terms is constant. Difference here means the second term minus the first term. a1 (pronounce "a sub 1") is the initial term of an arithmetic sequence, d is the common difference, an (pronounce "a sub n") - nth term. Example: the sequence 5, 7, 9, 11, 13, 15, .. . is an arithmetic sequence d = 2, a1 = 5, an = 3 + 2n Write the first 5 terms of the arithmetic sequence. 7. The first term 25 and the common difference 4. 8. The first term 30 and the common difference -3. Fill in the gaps in this arithmetic sequence. 9 . - 2 , 22 10. 15, -6 Determine which of the following sequences are arithmetic. For each arithmetic sequence, name the common difference. 1 1. 1, 3, 5, 7, 9, ... 12. 1, 2, 4, 7, 1 1, ... 13. 20, 12, 4, -4, -12, ... 14. an = 5n - 1 15. an = n+5 16. an = -2(2n + 3) 17. an = 0.5n 18. an = n3 19. an = 4n 20. 15, 9, 3, -3, -9, -15, ... 21. 9.27, 9.29, 9.31, 9.33, 9.35