Answer both 1 and 2 with full *handwritten* solutions
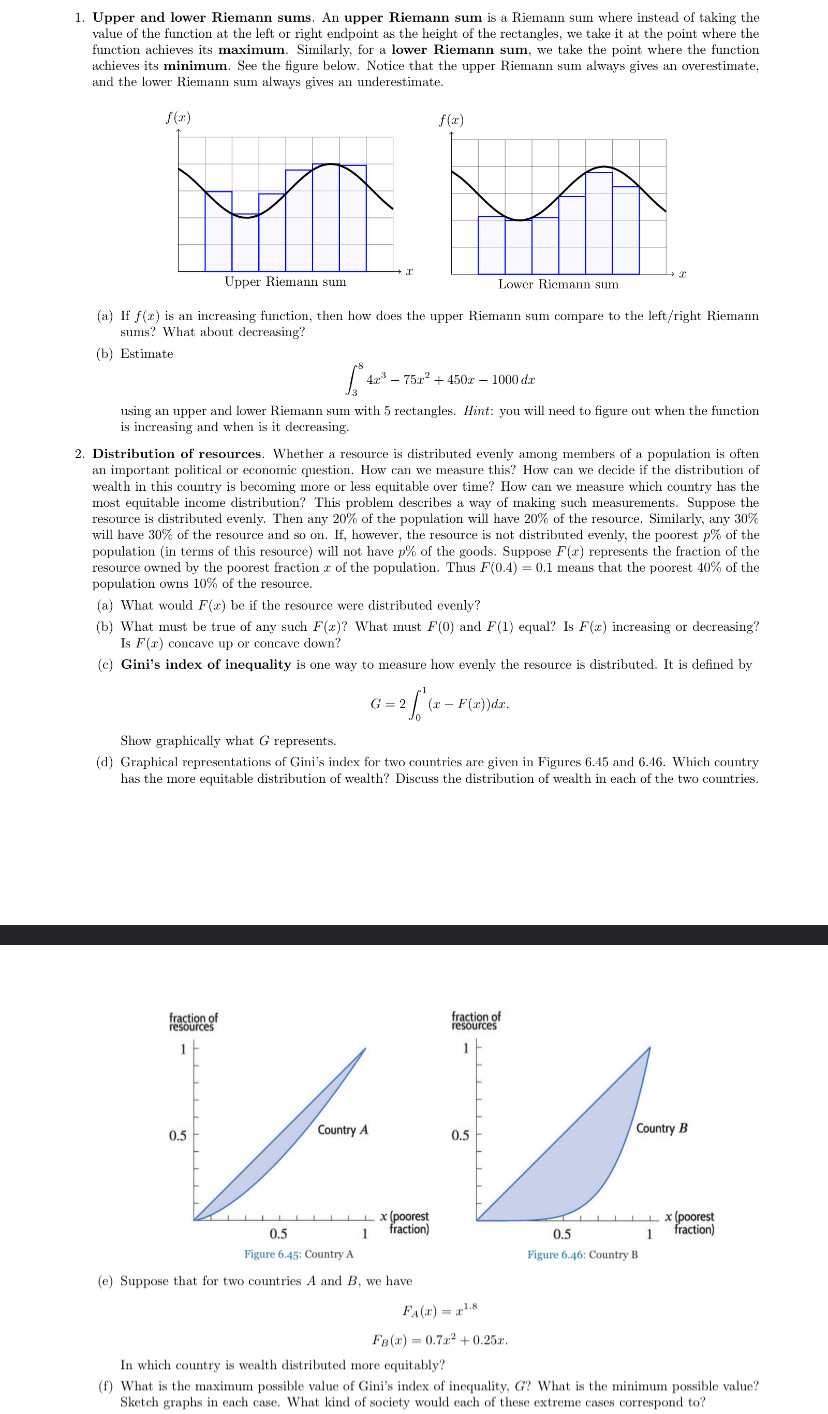
1. Upper and lower Riemann sums. An upper Riemann sum is a Riemann sum where instead of taking the value of the function at the left or right endpoint as the height of the rectangles, we take it at the point where the function achieves its maximum. Similarly, for a lower Riemann sum, we take the point where the function achieves its minimum. See the figure below. Notice that the upper Riemann sum always gives an overestimate, and the lower Riemann sum always gives an underestimate. f(x) f() Upper Riemann sum Lower Riemann sum (a) If f(x) is an increasing function, then how does the upper Riemann sum compare to the left/ right Riemann sums? What about decreasing? (b) Estimate fax' - 7527 + 450x - 1000 da using an upper and lower Riemann sum with 5 rectangles. Hint: you will need to figure out when the function is increasing and when is it decreasing. 2. Distribution of resources. Whether a resource is distributed evenly among members of a population is often an important political or economic question. How can we measure this? How can we decide if the distribution of wealth in this country is becoming more or less equitable over time? How can we measure which country has the most equitable income distribution? This problem describes a way of making such measurements. Suppose the resource is distributed evenly. Then any 20% of the population will have 20% of the resource. Similarly, any 30% will have 30% of the resource and so on. If, however, the resource is not distributed evenly, the poorest p% of the population (in terms of this resource) will not have p% of the goods. Suppose F(a) represents the fraction of the resource owned by the poorest fraction a of the population. Thus F(0.4) = 0.1 means that the poorest 40% of the population owns 10% of the resource. (a) What would F() be if the resource were distributed evenly? (b) What must be true of any such F(x)? What must F(0) and F(1) equal? Is F() increasing or decreasing? Is F(r) concave up or concave down? (c) Gini's index of inequality is one way to measure how evenly the resource is distributed. It is defined by G =2 (x - F(x])dr. Show graphically what G represents. (d) Graphical representations of Gini's index for two countries are given in Figures 6.45 and 6.46. Which country has the more equitable distribution of wealth? Discuss the distribution of wealth in each of the two countries. fraction of fraction of resources 1 0.5 Country A 0.5 Country B x (poorest 1 x (poorest 0.5 fraction) 0.5 1 fraction) Figure 6.45: Country A Figure 6.46: Country B (e) Suppose that for two countries A and B, we have FA(x) = $ FB(x) = 0.7x2 + 0.25x. In which country is wealth distributed more equitably? (f) What is the maximum possible value of Gini's index of inequality, G? What is the minimum possible value? Sketch graphs in each case. What kind of society would each of these extreme cases correspond to








