Answer the following

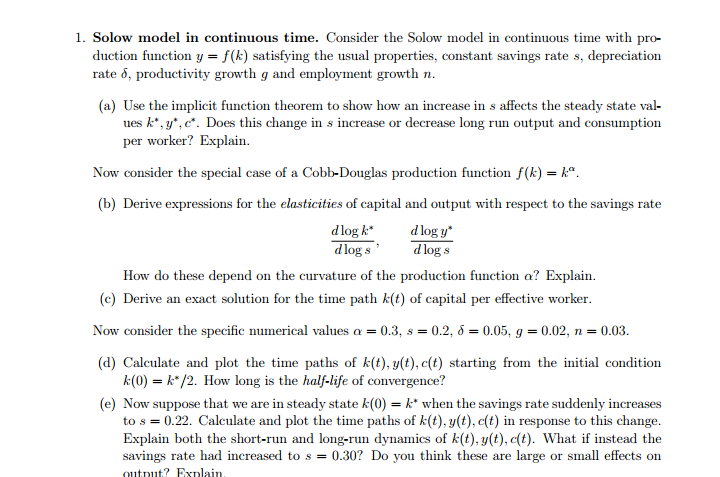
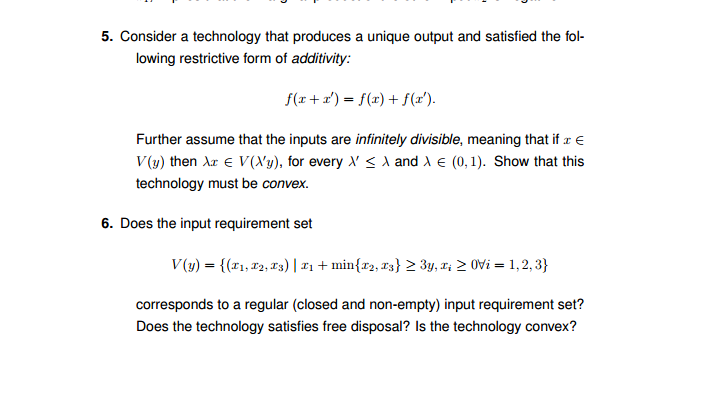
1. Solow model in continuous time. Consider the Solow model in continuous time with pro- duction function y = f(k) satisfying the usual properties, constant savings rate s, depreciation rate 6, productivity growth g and employment growth n. (a) Use the implicit function theorem to show how an increase in s affects the steady state val- ues k*, y', c'. Does this change in s increase or decrease long run output and consumption per worker? Explain. Now consider the special case of a Cobb-Douglas production function f(k) = k". (b) Derive expressions for the elasticities of capital and output with respect to the savings rate d log k* d logy* dlog s * d log s How do these depend on the curvature of the production function a? Explain. (c) Derive an exact solution for the time path k(t) of capital per effective worker. Now consider the specific numerical values a = 0.3, s = 0.2, 6 = 0.05, g = 0.02, n = 0.03. (d) Calculate and plot the time paths of k(t), y(t), c(t) starting from the initial condition k(0) = k*/2. How long is the half-life of convergence? (e) Now suppose that we are in steady state k(0) = k* when the savings rate suddenly increases to s = 0.22. Calculate and plot the time paths of k(t), y(t), c(t) in response to this change. Explain both the short-run and long-run dynamics of k(t), y(t), c(t). What if instead the savings rate had increased to s = 0.307 Do you think these are large or small effects on output? Explain1. Is it correct to state that 'if a firm has constant returns to scale, then the marginal products of labour and capital depend only on the ratio of labour and capital'? Explain. Can you state the same for an homothetic produc- tion function? Explain 2. Consider a Cobb-Douglas Production function: f(x) = 1i12, a>0, 8>0. (i) Sketch the production possibility set Z in each of the three cases; 1. a+ 3 1; 3. a+3=1. For each case, sketch three typical 'slices' through Z perpendicular to each of the axes in turn. Also draw the marginal product, average prod- uct, and marginal rate of technical substitution, as functions of $1. (ii) Is the production function homothetic and/or homogeneous? If homo- geneous, homogeneous in what, and to what degree? (ii) What returns to scale are there? 3. Consider the following four production plans obtained from the same tech- nology (production possibility set). Each plan yields one unit of output using two inputs. The plans are described by the following vectors: Plan 1: (1, -1, -6) Plan 2: (1, -2, -5) Plan 3: (1, -3, -3) Plan 4: (1, -5, -2) Is any of these production plans inefficient, in the sense that there exists no input price ratio at which a cost minimizing producer will choose the production plan in question? 4. Is it correct to state that for an homogeneous of degree one production function y = f(21, 12), an increasing average product for one input, say 1, implies that the marginal product of the other input r2 is negative? 5. Consider a technology that produces a unique output and satisfied the fol- lowing restrictive form of additivety: f(x + x') = f(x) + f(z'). Further assume that the inputs are infinitely divisible, meaning that if a e V(y) then Ar E V(Xy), for every >' and A E (0, 1). Show that this technology must be convex.5. Consider a technology that produces a unique output and satisfied the fol- lowing restrictive form of additivety: f(x + 1') = f(x) + f(x'). Further assume that the inputs are infinitely divisible, meaning that if r e V(y) then Ar E V()'y), for every >' and A E (0, 1). Show that this technology must be convex. 6. Does the input requirement set V(y) = {(21, 12, 23) | 21 + min( 12, x3} 2 3y, 1, 2 0Vi = 1,2,3} corresponds to a regular (closed and non-empty) input requirement set? Does the technology satisfies free disposal? Is the technology convex?1. Assume that the cost function associated with a given technology is different tiable. Show that average cost is rising (falling) as marginal cost is higher (lower) than average cost. 2. Consider a Cobb-Douglas Production function: f(x) = nix, where a > 0, 8 > 0 and a + B 0, 8 > 0 and make no assumptions on a + B. (i) Derive the conditional factor demands hi (w, y) and h2(w, y). (ii) Find the 2 x 2 matrix of marginal price effects. Confirm the signs (and, where appropriate, relative magnitudes) of these effects. (iii) Find the cost function c(w, y). Confirm its properties. (iv) Sketch c(w, y) as a function of y for each of the three cases a + 8 > 1, a + 0 and B > 0. (i) Identify the Walrasian Equilibrium of this economy in terms of endow- ments and the parameters of the utility function. [Hint: is there some principle of consumer theory and of general equilib rium theory that can simplify things a little?] 2. In a two-persons (Ms. A and Mr. B), two-commodities (apples and oranges), pure exchange economy, Ms. A likes only apples and does not care how many oranges she has. On the other hand, Mr. B likes only oranges and does not care how many apples he has. Both people behave as price-takers. (i) Suppose that A owns all the apples and B all the oranges. Is there a Walrasian equilibrium? If so, what is (are) the equilibrium price and allocation(s)? (ii) What are the Pareto optimal allocations in such an economy? (iii) Suppose now that the initial endowments are such that Ms. A owns some oranges and Mr. B some apples. Is there a Walrasian equilibrium? If so, describe any equilibrium price and allocation. 3. In a two-persons (Ms. A and Mr. B), two-goods (good X] and good X2), pure exchange economy, Mr. A likes only good X, and does not care how many units of good X2 he has. In other words, Mr. A's preferences are represented by the utility function: UA(XA, XA) = Xf. On the other hand, Ms. B likes only good X2 she does not care how many units of good X, she consumes, but she suffers from a negative externality imposed on her by Mr. A's consumption of good X1. In other words, Ms. B's preferences are represented by the following utility func- tion: UB(XP, XP, XA) = X5 - X4. Finally, assume that Mr. A owns 5 units of good X, and 15 units of good X2, while Ms. B owns 15 units of good X', and 5 units of good X2. (i) Is there any Walrasian equilibrium in this economy? ii) If so, what is (are) the Walrasian equilibrium price(s) and allocation(s)? (ii) Find the Pareto efficient allocation(s) in such an economy. (vi) Is the Walrasian equilibrium allocation Pareto efficient? Explain your