ANswer the following questions.

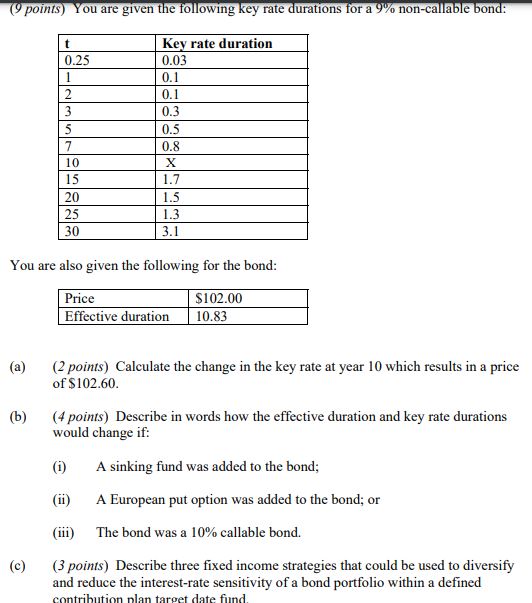
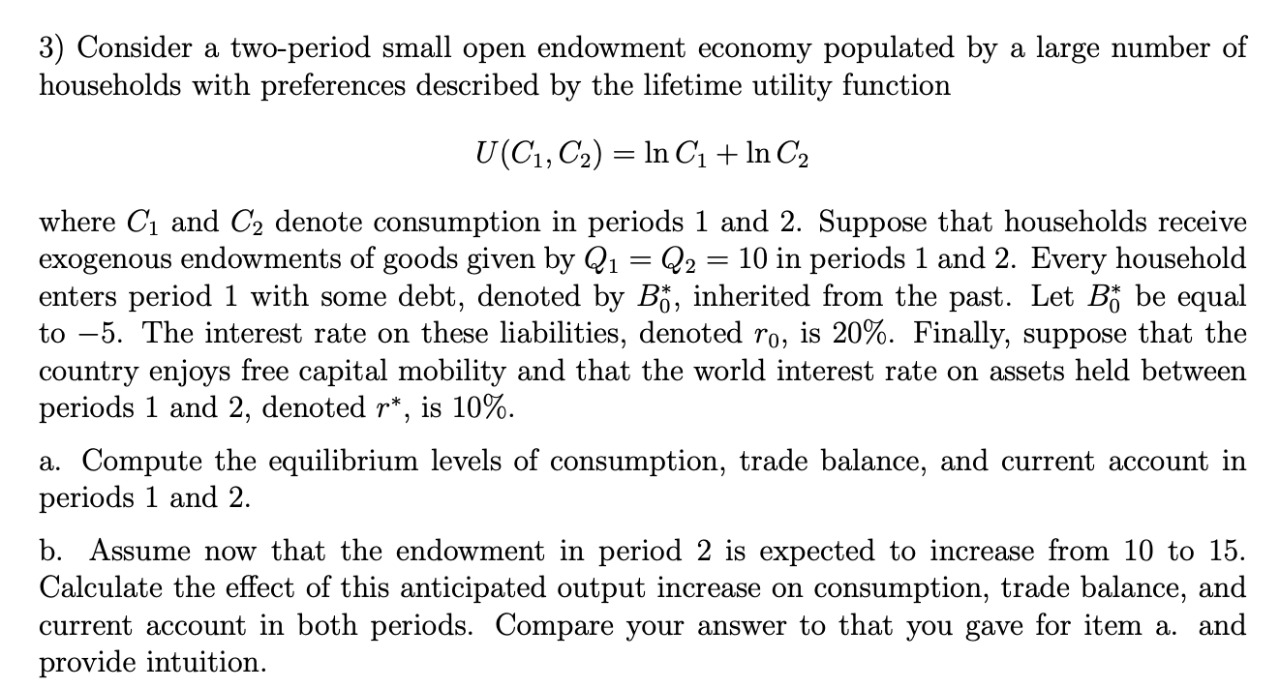
(9 points) You are given the following key rate durations for a 9% non-callable bond: Key rate duration 0.25 0.03 0. 1 2 0.1 3 0.3 5 0.5 7 0.8 10 X 15 1.7 20 1.5 25 1.3 30 3.1 You are also given the following for the bond: Price $102.00 Effective duration 10.83 (a) (2 points) Calculate the change in the key rate at year 10 which results in a price of $102.60. (b) (4 points) Describe in words how the effective duration and key rate durations would change if: (i) A sinking fund was added to the bond; (ii) A European put option was added to the bond; or (iii) The bond was a 10% callable bond. (c) (3 points) Describe three fixed income strategies that could be used to diversify and reduce the interest-rate sensitivity of a bond portfolio within a defined contribution plan target date fund.3) Consider a two-period small open endowment economy populated by a large number of households with preferences described by the lifetime utility function U(01,Cz) = 11101 +1nC'2 where 01 and 02 denote consumption in periods 1 and 2. Suppose that households receive exogenous endowments of goods given by Q1 = Q2 = 10 in periods 1 and 2. Every household enters period 1 with some debt, denoted by BS, inherited from the past. Let 35 be equal to 5. The interest rate on these liabilities, denoted To, is 20%. Finally, suppose that the country enjoys free capital mobility and that the world interest rate on assets held between periods 1 and 2, denoted r", is 10%. a. Compute the equilibrium levels of consumption, trade balance, and current account in periods 1 and 2. b. Assume now that the endowment in period 2 is expected to increase from 10 to 15. Calculate the effect of this anticipated output increase on consumption, trade balance, and current account in both periods. Compare your answer to that you gave for item a. and provide intuition. A simple NCD system has four levels of discount - 0%, 20%, 40% and 60%. A new policyholder starts on 0% discount. At the end of each policy year, policyholders will change levels according to the following rules: At the end of a claim free year, a policyholder moves up one level, or remains on the maximum discount. At the end of a year in which exactly one claim was made, a policyholder drops back one level, or remains at 0%. At the end of a year in which more than one claim was made, a policyholder drops back to zero discount. For a particular policyholder in any year, the probability of a claim free year is fo, the probability of exactly one claim is y, and the probability of more than one claim is X10 (i Write down the transition matrix for this time homogeneous Markov chain. [2] (ii) Calculate the 2-step transition probabilities from state i to state j , p,". [3] (iii) If the policyholder starts with no discount, calculate the probability that this policyholder is at the maximum discount level 5 years later. [5] (iv) If a large number of people having the same claim probabilities take out policies at the same time, calculate the proportion would you expect to be in each discount category in the long run. [5] [Total 15]A simple NCD system has four levels of discount - 0%, 20%, 40% and 60%. A new policyholder starts on 0% discount. At the end of each policy year, policyholders will change levels according to the following rules: At the end of a claim free year, a policyholder moves up one level, or remains on the maximum discount. At the end of a year in which exactly one claim was made, a policyholder drops back one level, or remains at 0%. At the end of a year in which more than one claim was made, a policyholder drops back to zero discount. For a particular policyholder in any year, the probability of a claim free year is fo, the probability of exactly one claim is y, and the probability of more than one claim is X10 (i Write down the transition matrix for this time homogeneous Markov chain. [2] (ii) Calculate the 2-step transition probabilities from state i to state j , p,". [3] (iii) If the policyholder starts with no discount, calculate the probability that this policyholder is at the maximum discount level 5 years later. [5] (iv) If a large number of people having the same claim probabilities take out policies at the same time, calculate the proportion would you expect to be in each discount category in the long run. [5] [Total 15]4. In August 2014, Tesla announced a new "Infinite Mile Warranty". A Forbes article reported: "Tesla announced that it would extend the warranty on the drive unit of its Model S cars to match the battery pack's 8 years and unlimited miles with no limit on the number of owners. This will apply to new Model S models and all ever produced." (Chuck Jones, Forbes, Aug 18, 2014) (a) Suppose that before this warranty is in place, demand for Tesla's model S car specifically is given by: P = 200 - 20, where P is in thousands of dollars per car, and @ is in thousands of cars. Suppose that prior to the new warranty, Tesla's marginal cost of production was constant at 32 thousand dollars per car. Based on these assumptions, prior to the offer of the new warranty what is profit maximizing price per car for Tesla? What quantity is sold? What is the consumer and producer surplus from sale of the model S? (b) Offering this warranty costs Tesla money (since they now have to fix things that break). Specif- ically, Tesla estimates that it will cost them $1 thousand per ten thousand miles driven. Tesla's estimates show that the average customer of their Model S would drive 60 thousand miles in eight years. So all-in-all the expected cost to Tesla of the extended warranty is $6 thousand per car. Assuming that Tesla is risk neutral, what is Tesla's new marginal cost per car? (c) Assume that all buyers of Teslas are risk averse. What will the introduction of this warranty do to the demand curve for this car? (You can't give me an exact equation, but you can tell me something about what happens to the demand curve.) (d) Specifically, suppose that offering the eight year, unlimited mileage warranty increases each po- tential customer's willingness to pay by 10 thousand dollars. With this, calculate the new profit maximizing price that Tesla will charge for a Model S with the warranty. What are consumers and producer surplus now? Did they individually go up or down (from part (a))? Explain, intuitively, why. (e) Recent articles suggest that Tesla's extended warranty may be costing the company more than anticipated. An article in October 2017 on Seeking Alpha noted that in particular, taxi and ride sharing service drivers (who drive a lot more miles than the average driver) are buying the model S because of the unlimited mileage warranty. Suppose that, due to larger than expected purchases by drivers who drive a lot of miles, Tesla revises its estimate of the mileage usually driven during the eight year warranty period to 120 thousand miles (up from 60 thousand miles). Suppose that demand, the cost of the warranty per thousand miles, and all other costs stay the same as above. Under the revised cost assumptions would Tesla's surplus increase or decrease or remain the same if they offered the warranty compared to if they didn't? You can answer qualitatively here (just say "increase" or "decrease" or "remain the same"), but be sure to explain why. Is the nature of the problem described here that of adverse selection or moral hazard? Explain