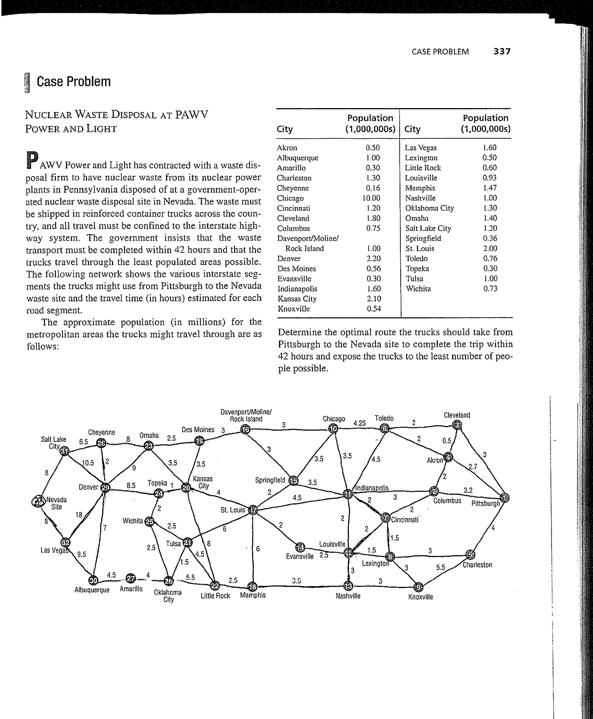
Case Problem THE DRAPERTON PARKS AND RECREATION DEPARTMENT'S FORMATION OF GIRLS' BASKETBALL TEAMS Each fall the Draperton Parks and Recreation Depart- ment holds a series of tryouts for its boys and girls' youth basketball leagues. All leagues are formed by age group. each usually encompassing a couple of ages. One such grouping is the 12- to 13-year-old girls' league. This is a particularly competitive age group because the girls are all trying to improve their skills in hopes of making the high school junior varsity team in the immediate future and the high school varsity in several years. Some of the girls even hope to make the high school varsity next year, when they are freshmen. This is also the first age group in which not all girls who try out are placed on teams; some are cut. In the younger age groups, all players are assigned to teams. However, at this age group, four teams are formed, each with only seven players. This policy was agreed upon by a committee consisting of the Recreation Department direc- tor, a group of parents of past players, and the high school boys and girls' basketball coaching staff. The small roster size allows each player to get a lot of playing time and makes the games more competitive. It also makes the teams more competitive in tournaments with teams from other towns and cities. This makes the girls very competitive, and it makes their parents even more compet- itive. Sandy Duncan, the Recreation Department's director of team sports, knows from past experience that parents do a lot of jockeying and politicking during tryouts. This has given rise to parental protests regarding the tryout process and about how players are selected and assigned to teams. Sandy and the player evaluators have frequently been ac- cused of showing favoritism and stupidity. This year, in an effort to blunt some of this criticism, Sandy has devised a tryout process that will hopefully re- move her (and any other person) from direct involvement with the player selection and team formation process. First, she conducted tryouts for all 36 players who were trying out over two weekends that included four sessions tach weekend: one on Friday evening, two on Saturday. M 1 and one on Sunday afternoon. She formed an evaluation team of several coaches from other towns and some of the current players and assistant coaches from the nearby State University women's basketball team. Each player was as- signed a tryout number and evaluated for different skills and ability, and at the end of the tryouts, each player was given an overall score from 1 to 10. with 10 being the high- est, as follows: Player 1 2 3 4 4 5 6 7 8 Q 10 11 12 Score 7 6 9 C 5 7 8 3 2 3 4 6 4 8 Player 13 14 15 * 16 17 18 19 20 21 22 23 CASE PROBLEM 24 Score 10 9 9 4 5 5 6 H 3 2 1 S Player 25 26 229 27 28 29 30 31 32 33 34 35 36 Score 8 4 9 9 3 3 6 6 7 8 10 Sandy took a course in management science while she was a student at State University, and she wants to develop an integer linear programming model to use these evaluation scores to select the players and assign them to the four teams in a fair and equitable manner. She wants to select the best players, and in order to have competitive, equally matched teams, she wants each team to have an average. overall player evaluation score between 6 and 7. As she sat down one evening to work on this problem, she quickly re- alized that it would require a relatively complex integer programming model. Help Sandy formulate and solve an integer programming model for this problem to select the best players, assign seven players to each team, and achieve the average team evaluation score Sandy wants for each team. Compare the teams formed by your model and determine whether you think they are competitive (i.e.. whether the model has achieved Sandy's objectives). Also, determine whether any deserving players were unfairly cut by the model. 230 CHAPTER 5 INTEGER PROGRAMMING Case Problem DEVELOPING PROJECT TEAMS FOR MANAGEMENT 4394 All students in the College of Business at State Univer- sity are required to take a capstone course, Management 4394, the last semester of their senior year. This course consists primarily of a semester-long case project that pulls together all the business disciplines to test the students' knowledge in the major business areas of man- agement, marketing, finance, accounting, quantitative methods, and operations. A difficult task for the course in- structor is dividing the students in a class into equitable teams that will enhance the learning process and engender teamwork. The project teams should be relatively equal in terms of student ability and performance. Further, the teams should reflect different functional skills; that is, the teams should include a mix of different majors. Also, the college would like the teams to be diverse in terms of gender and nationalities in order to prepare the students for working with diverse individuals when they graduate Student 1 2 3 5 9 10 11 12 13 14 15 16 17 18 GPA Gender International (yes or no). M 3.04 2.35 M M M F F 2.26 2.15 2.15 3.23 3.95 2.87 2.65 3.12 3.08 3.35 2.78 2.56 2.91 3.40 3.12 2.75 3.06 M F M M M M F F F M M F Yes No Yes Yes No No No No No Yes Yes No Yes No Yes Yes Yes No Note that only two students are both female and interna- tional, and that student 2 is a double major. Formulate and solve an integer linear programming model for this problem to determine project teams that will meet the instructor's guidelines for team formation. How successfully do you think your model achieves the instruc- tor's guidelines? In other words, do you consider the teams. to be diverse and relatively equitable in terms of functional. and take a job. Consider one course section of Management 4394 with 18 students. The instructor wants to divide the class into six project teams of three members each. The in- structor's main objective for the teams is that they be rela- tively equal in terms of academic capability. Since the only means of assessing capability is a student's grade point average (GPA), the instructor has determined that one way to achieve this objective is for the teams. to have a minimum average GPA of 2.80 while also striv ing to achieve the maximum overall average team GPA possible. It is also important that the teams have a mix of members with different majors to enhance the teams' functional capabilities, but the instructor does not want there to be more than two of the same major on any single team. In order to achieve some degree of diversity among team members the instructor wants there to be at least one female and one international student on each team, but not more than two females or two international students on a team. Following is a table that shows the breakdown of GPAS, majors, gender, and internationality of each student in the class. Major FIN FIN/ACCT MGT MKTG MGT FIN FIN FIN FIN MKTG MKTG ACCT MKTO ACCT MGT FIN ACCT MGT and academic capability? If not, how do you think you might need to change your model to better meet the team formation guidelines? This case problem is based on R. Cutshall, S. Gavineni, and K. Sch "Indiana University's Kelley School of Business Uses Integer Prog ming to Form Equitable, Cohesive Student Teams." Interfaces 37, (May-June 2007): 265-76. Case Problem SCHEDULING THE LEAD BALLOON'S SUMMER TOUR The Lead Balloon is a popular '80s rock band that broke up in the 1990s but recently got back together to publish a new CD and go on tour. They want to schedule a summer tour that will encompass 16 possible cities in the eastern half of the United States. However, because of family and business commitments, plus the fact they are not so young and energetic as they used to be, the tour will encompass only 12 weeks, from mid-May to mid-August, when their children will be out of school, so that their families can join them on the tour most of the time. The band also wants to limit the number of concerts each week. Follow- ing is a list of the possible cities with the weeks that the City Atlanta Boston Cincinnati. Charlotte Cleveland Detroit DC Philadelphia Dallas Miami Orlando Houston Indianapolis Nashville Chicago New York 3 May X X X X X X X Case Problem 4 X X X X X X X X X 1 X X X X X 2 June X X X X X X 3 X X X X X 4 X X X X X DEVELOPING KATHLEEN TAYLOR'S 401(K) PLAN Kathleen Taylor has been working for a government con- tractor, Summit Solutions, in Washington, DC, for over a year. She is now eligible to participate in the company's local arenas in each city will be available (marked with an X) during the tour. Also included is the concert capac- ity of the arena in each city. Based on its popularity and fame it is a foregone con- clusion that the band will sell out everywhere it goes. Thus, the band wants to schedule the cities that will maxi- mize the total attendance at its concerts, which basically means it wants to schedule the cities with the largest are- nas, if possible. The band has also stipulated that it wants. to perform at least one concert but no more than two con- certs each week. Also, because of the band's high-tech. equipment and gear, and sound and stage setup require- ments, logistical constraints limit the travel between two cities in any one week to 500 miles. 1 X Develop a summer tour schedule for the band that will maximize total attendance. X X X 2 X July X X X X X 3 X X X X X 4 X X X X X August 1 X X CASE PROBLEM X X X X X 2 X X X X X X X X 231 Capacity 19,500 18,500 16,200 17,600 22,700 24,200 21,500 21,300 17,400 15,700 16,300 18,200 25,400 16.500 22,800 20,700 401(k) retirement plan. The company has provided Kathleen with the information in the table on the following page about the various funds that are available for her to invest in. 232 CHAPTER 5 INTEGER PROGRAMMING International Parham International Value Jarus Overseas U.S. Fund EuroPacific Admiral Foreign Investor SPS Emerging Market Small-Cap Maxam Small Cap Return U.S. Small Cap Select Veritas Small Cap Value Oak Small Cap Growth Mid-Cap Federal Mid Cap Growth T. Row Price Growth Draper Structured Mid Cap Jarus Mid Cap Value Maxam Mid Cap Opportunity Large-Cap T. Row Price Bluechip Growth Jarus Diversified Equity Income Draper Strategic Growth Centennial Common Stock Growth Marius Stock Growth Bond Maxam Global Bond Parham High Yield Madison Federated Bond Portfolio Draper U.S. Government Bond Federated High Income Evening Star Rating 4 3 4 wawww 3 5-Year Return. 12.58 6.94 7.86 7.54 5.57 Since Kathleen is young and expects to build her 401(k) plan over a long period of time, she wants to 5.87 3.99 2.97 3.8 4.13 6.30 7.73 4.05 5.76 6.01 3.22 7.81 2.16 9.88 International funds invest in global/overseas compa- nies; small-cap funds invest in companies that have a mar- ket capitalization (i.e., the number of outstanding shares multiplied by the stock price per share), in general, be- tween $300 million and $2 billion; mid-cap funds invest in companies with a market capitalization between $2 and $10 billion; and large-cap funds invest in companies over $10 billion. The Evening Star rating is developed by an in- dependent investment analysis firm, and it rates funds based on their risk-adjusted performance over various time periods. "5" is best. "I" is worst; stocks trading close to their analysts' fair value estimates receive a "3", while stocks trading at large discounts compared to their ana- lysts' fair value estimate receive a "4" or "5" rating. The expense ratio is the total percentage of fund assets used for operating expenses (i.e.. administrative, management. advertising, etc.). 8.37 6.25 5.66 7.41 5.85 Fund Size ($1,000,000s) 2.066 7,404 19.859 42,035 11.139 1.302 770 3.049 4.320 729 4,145 1.467 4,632 2.537 6.374 962 9.991 20,194 19,648 12,942 3.106 8,402 1.045 11,211 Expense Ratio 1.67 1.97 1.19 300 1.32 1.15 1.43 1.21 1.74 1.49 1.05 1.33 1.31 1.52 1.17 1.23 1.05 1.16 0.99 1.12 1.03 1.00 1.14 0.90 0.82 employ a relatively aggressive investment strategy. She has read investment literature that suggests a relatively aggres sive plan would invest 5% to 35% in international funds, 5% to 25% in small-cap funds, 5% to 35% in mid-cap funds, 20% to 50% in large-cap funds, and 5% to 10% in bond funds. Kathleen plans to contribute $900 of her salary each month to her plan, which the company will match. She has also developed a few of her own invest- ment guidelines: to diversify, she wants to invest in five funds, one in cach investment category, she wants to achieve an average Evening Star rating of at least 3.7; she wants to invest in funds that average at least $10.000 mil- lion in size; she wants to achieve an average expense ratio for her five funds of no more than 1.10; and she wants to maximize the average 5-year return of the five funds she selects, weighted by the amount she invests in each. Develop an investment plan for Kathleen using linear programming. How would her investment plan change if she wanted to maximize the Evening Star rating? Case Problem NUCLEAR WASTE DISPOSAL AT PAWV POWER AND LIGHT PAWV Power and Light has contracted with a waste dis- posal firm to have nuclear waste from its nuclear power plants in Pennsylvania disposed of at a government-oper- ated nuclear waste disposal site in Nevada. The waste must be shipped in reinforced container trucks across the coun- try, and all travel must be confined to the interstate high- way system. The government insists that the waste transport must be completed within 42 hours and that the trucks travel through the least populated areas possible. The following network shows the various interstate seg- ments the trucks might use from Pittsburgh to the Nevada waste site and the travel time (in hours) estimated for each road segment. The approximate population (in millions) for the metropolitan areas the trucks might travel through are as follows: Salt Lake Chye 8 Nevada Site Cheyenne 6.5 20 10.5 2 Denver 18 Las Vega 5.5 (7 4,5 9 8.5 Omaha 25 Wichita 25 Albuquerque Amarillo 3.5 3.5 Kansas Topela Cy 24 /2 2.5 Des Moines 3 2.5 Tulsa 21 18 45) 15 Oklahoma City Davenport/Moline Rock Island 5.5 St. Louis City Akron Albuquerque Amarillo Charleston Cheyenne Chicago Cincinnati Cleveland Columbus Davenport/Moline/ Rock Island Denver Des Moines Evansville Indianapolis Kansas City Knoxville 2.5 Little Rock Memphis Springfield 2 4.5 3.5 3.5 Chicago Population (1,000,000s) City 3.5 3.5 Evansvile 25 2 Louisville 0.50 1.00 0.30 1.30 0.16 10.00 4.25 3 Nashvi 1.20 1.80 0.75 1.00 2.20 Determine the optimal route the trucks should take from Pittsburgh to the Nevada site to complete the trip within 42 hours and expose the trucks to the least number of peo- ple possible. 0.56 0.30 1.60 2.10 0.54 Andanarolis Toledo 2 4.5 CASE PROBLEM 1.5 1.5 Lexington 3 Las Vegas Lexington Little Rock Louisville Memphis Nashville Oklahoma City Omaha Salt Lake City Springfield St. Louis Toledo Topeka Tulsa Wichita 2 Cincinnati Akron 0.5. Kale Population (1,000,000s) Cleveland 27 3.2 337 1.60 0.50 0.60 0.93 1.47 1.00 1.30 1.40 1.20 0.36 2.00 0.76 0.30 1.00 0.73 3 Columbus Pisburgh 5.5 Charleston