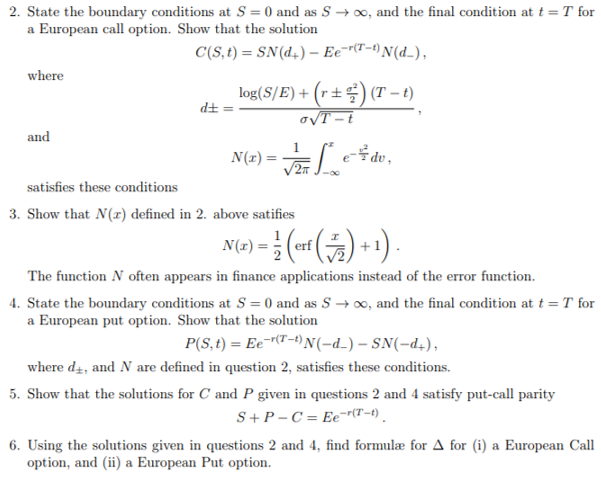
Answers needed for part 2, 3, 4, 5 & 6 with step by step solution thanks :)

2. State the boundary conditions at S= 0 and as S 00, and the final condition at t= T for a European call option. Show that the solution C(S. t) = SN (dt) - Ee-(T-)(d). where log(S/E)+(-+*) (1 t) d+= OT-7 and N() = M2Le*dv, satisfies these conditions 3. Show that N () defined in 2. above satifies N(r) = } (erf () +1). The function N often appears in finance applications instead of the error function. 4. State the boundary conditions at S = 0 and as s oo, and the final condition at t = T for a European put option. Show that the solution P(S,t) = Ee-(T-N(-d.) - SN(-), where d, and N are defined in question 2, satisfies these conditions. 5. Show that the solutions for C and P given in questions 2 and 4 satisfy put-call parity S+P-C = Ee- (T-1) 6. Using the solutions given in questions 2 and 4, find formul for A for (i) a European Call option, and (ii) a European Put option. 2. State the boundary conditions at S= 0 and as S 00, and the final condition at t= T for a European call option. Show that the solution C(S. t) = SN (dt) - Ee-(T-)(d). where log(S/E)+(-+*) (1 t) d+= OT-7 and N() = M2Le*dv, satisfies these conditions 3. Show that N () defined in 2. above satifies N(r) = } (erf () +1). The function N often appears in finance applications instead of the error function. 4. State the boundary conditions at S = 0 and as s oo, and the final condition at t = T for a European put option. Show that the solution P(S,t) = Ee-(T-N(-d.) - SN(-), where d, and N are defined in question 2, satisfies these conditions. 5. Show that the solutions for C and P given in questions 2 and 4 satisfy put-call parity S+P-C = Ee- (T-1) 6. Using the solutions given in questions 2 and 4, find formul for A for (i) a European Call option, and (ii) a European Put option