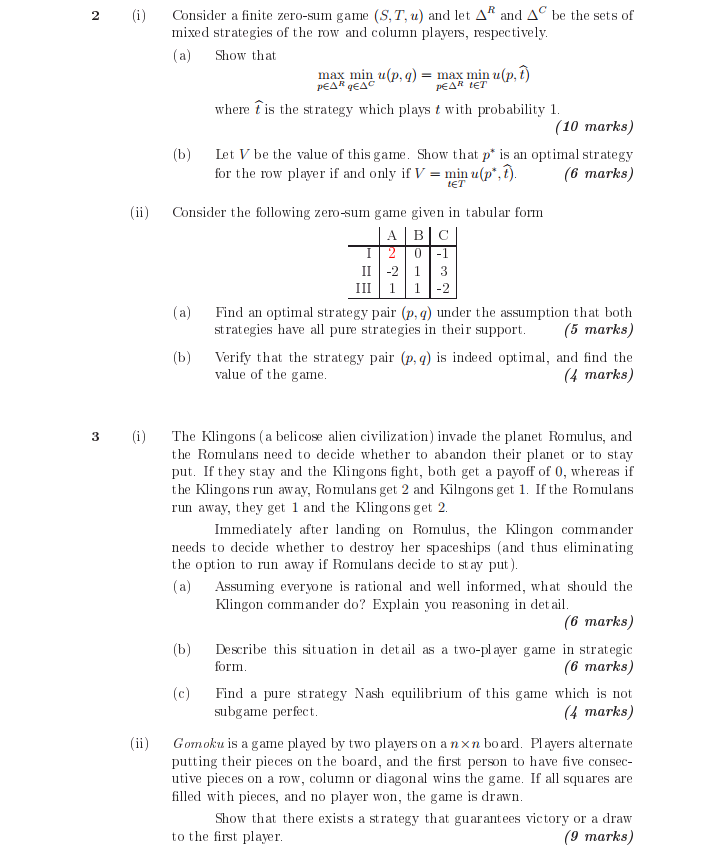
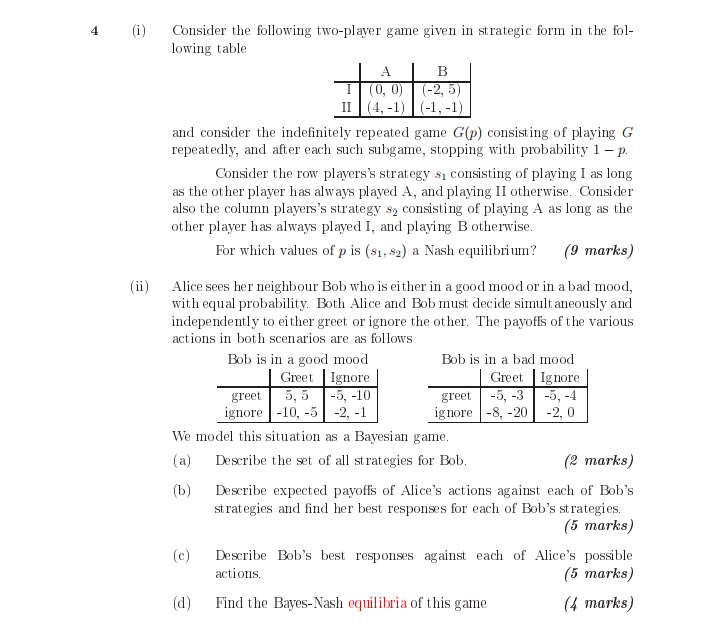
Appropriate answers

3 (i) Alice manufactures microprocessors and Bob manufactures computers using these components. Bob can buy Alice's microprocessors or produce his own. Alice has the option of launching an ad campaign promoting her products, or not doing so. She also has the choice between charging Bob a high price or a low price. In the event that Alice advertises and Bob buys from her, the profits for Alice and Bob are f15,000,000 and f12,000,000, respectively, if she charges a high price, and f12,000,000 and $15,000,000, respectively, if she charges a low price. In the event that Alice does not advertise and Bob buys from her, the profits for Alice and Bob are f12,000,000 and $8,000,000, respectively, if she charges a high price, and f10,000,000 and $10,000,000, respectively, if she charges a low price. Regardless of whether Alice decided to launch the ad campaign, if Bob decided to manufacture his own microprocessors, Alice's profits will be nil and Bob's will be 19,000,000. (a) Describe this game using a tree, carefully labelling all its compo- nents. (7 marks) (b) Solve this game using backward induction. (5 marks) (c) List all the subgames of this game. (5 marks) (d) Describe the game in strategic form, find all its pure-strategy Nash equilibria and indicate which of these is subgame perfect. (8 marks)1 (i) Alice and Bob are given $4 to divide among themselves and they agree to do so as follows. Both pick simultaneously a non-negative integer less or equal to 4 and . if the integers add up to 4 or less, each gets the amount they named, . if the integers are different and add up to more than 4, the person who chose the smaller number gets that amount and the other person gets the rest, and . if the integers are equal and add up to more than 4, each gets $2. (a) Describe this situation as a game in strategic form. (5 marks) (b) Find all strategies which are strictly dominated, and all strategies that are weakly dominated. (3 marks) (c) Find all pure-strategy Nash-equilibria of this game. (4 marks) (d) Show that the sequential elimination of weakly dominated strategies can lose Nash equilibria. (3 marks) (ii) Suppose that two firms produce similar but not identical products, and that the unit costs of these products are f10 for firm 1 and 620 for firm 2. The prices of each of these two products depend on the production profile (g1, 42) of both products: p1 = 100 - 6q1 - 292 and p2 = 200 - 591 - 7q2. Assume that each firm i controls its production profile qi. (a) Find each company's production which is the best response to the other company's production. (6 marks) (b) Find the production profile which is a Nash-equilibrium. (4 marks)[ii [iii Cbnsider a finite zerosum game {3, T, u} and let .\" and g be the sets of mixed strategies of the row and column players, respectively. [a] Show that maxminu =maxmiuu ,t pERth" {PM} pEAlET {1.3"} where fis the strategy which plays t with probability 1. (If! marks} [b] Let V be the value of this game. Show that p\" is an optimal strategy for the row player if and only if V =1gig1upfl { marks} Chnsider the following zero-sum game given in tabular form [a] Find an optimal strategy pair {go} under the assumption that both strategies have all pure strategies in their support. {5 marks} [b] Verify that the strategy pair {1), q} is indeed optimal, and find the value of the game. ["41 marks} The Klingons [a belicose alien civilization] invade the planet Romulus, and the Homulans need to decide whether to abandon their planet or to stay put. If they stay and the Klingons ght, both get a payoff of I], whereas if the Klingons run away, Romulans get 2 and Kilngons get 1. If the Eomulans run away, they get 1 and the Klingons get 2. Immediately after landing on Romulus, the Klingon commander needs to decide whether to destroy her spaceships [and thus eliminating the option to run away if Romulans decide to stay put]. [a] Assuming everyone is rational and well informed, what should the Klingon commander do? Explain you reasoning in detail. {5 marks} [b] Describe this situation in detail as a two-pl ayer game in strategic form. {If marks} [c] Find a pure strategy Nash equilibrium of this game which is not subgame perfect. {4 marks} Gomoku is a game played by two players on a nxn board. Players alternate putting their pieces on the board, and the first person to have five consec utive pieces on a row, column or diagonal wins the game. If all squares are filled with pieces, and no player won, the game is drawn. Show that there exists a strategy that guarantees victory or a draw to the rst player. {9 marks} 4 (i) Consider the following two-player game given in strategic form in the fol- lowing table B (0, 0) (-2, II (4, -1) (-1, -1 and consider the indefinitely repeated game G(p) consisting of playing G repeatedly, and after each such subgame, stopping with probability 1 - p. Consider the row players's strategy s, consisting of playing I as long as the other player has always played A, and playing II otherwise. Consider also the column players's strategy s, consisting of playing A as long as the other player has always played I, and playing B otherwise. For which values of p is ($1, $2) a Nash equilibrium? (9 marks) (ii) Alice sees her neighbour Bob who is either in a good mood or in a bad mood, with equal probability. Both Alice and Bob must decide simultaneously and independently to either greet or ignore the other. The payoffs of the various actions in both scenarios are as follows Bob is in a good mood Bob is in a bad mood Greet Ignore Greet Ignore greet 5, 5 -5, -10 greet -5, -3 -5, -4 ignore -10, -5 -2. ignore -8, -20 -2, 0 We model this situation as a Bayesian game. (a) Describe the set of all strategies for Bob. (2 marks) (b) Describe expected payoffs of Alice's actions against each of Bob's strategies and find her best responses for each of Bob's strategies. (5 marks) (c) Describe Bob's best responses against each of Alice's possible actions. (5 marks) (d) Find the Bayes-Nash equilibria of this game (4 marks)