Question: Archimedes devised a method for computing by approximating a circle of radius 1/2 by successive polygons and computing the perimeter of these polygons. This

Archimedes devised a method for computing by approximating a circle of radius

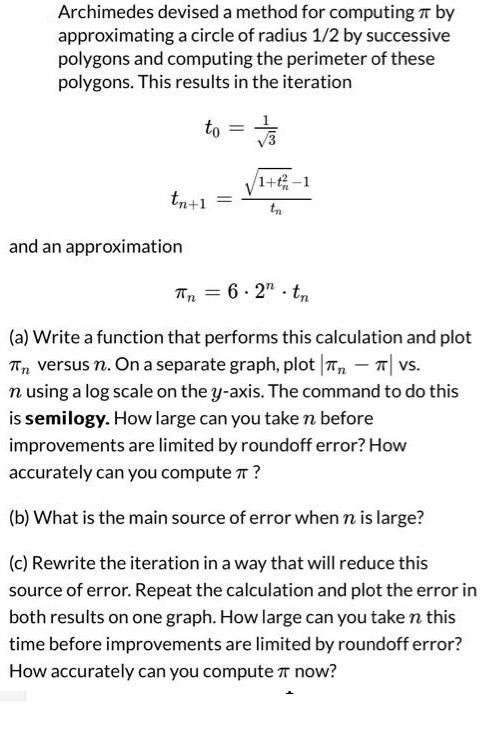
Archimedes devised a method for computing by approximating a circle of radius 1/2 by successive polygons and computing the perimeter of these polygons. This results in the iteration to 1+-1 tn+1 = tn and an approximation Tin = 6 -2 (a) Write a function that performs this calculation and plot n versus n. On a separate graph, plot | | vs. n using a log scale on the y-axis. The command to do this is semilogy. How large can you take n before improvements are limited by roundoff error? How accurately can you compute ? (b) What is the main source of error when n is large? (c) Rewrite the iteration in a way that will reduce this source of error. Repeat the calculation and plot the error in both results on one graph. How large can you take n this time before improvements are limited by roundoff error? How accurately can you compute now?

Step by Step Solution

★★★★★

3.57 Rating (154 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

To address this problem well implement the given iteration and analyze the errors involved Well then suggest an improvement to reduce the error a Impl... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Planning is one of the most important management functions in any business. A front office managers first step in planning should involve determine the departments goals. Planning also includes...

Do some amendment and enhance the given research paper: Table of Content Abstract..3 Action Research.4 Research Methodology and Design...5 Literature Review: NoSQL Database7 Proposal.7 Iteration 1..8...

A parametrization of a circle of radius 1 centered at (1, 0) in the xy-plane is given by x = 1 + cos t, y = sin t, for 0 t 2. Find the surface area when this curve is revolved about the y-axis.

Using the data presented in P10-21, prepare a worksheet to develop a consolidated statement of cash flows using the direct method for computing cash flows from operations. In p10-21, WEATHERBEE...

In each part of this problem, we have provided a scenario for a confidence interval. Decide whether the appropriate method for obtaining the confidence interval is the z-interval procedure, the...

In this section we have presented a commonly accepted way to compute the first, second, and third quartiles. Some statisticians, however, advocate an alternative method for computing Q1 and Q2 This...

Multiple Choice Questions The following questions concern the audit of liabilities or income and expense accounts. Choose the best response. a. The auditor may note that annual depreciation expense...

Suppose that trees are distributed in a forest according to a two-dimensional Poisson process with parameter a, the expected number of trees per acre, equal to 80. a. What is the probability that in...

The waiting times between a subway departure schedule and the arrival of a passenger are uniformly distributed between 0 and 9 minutes. Find the probability that a randomly selected passenger has a...

Accounting Change Ramirez Co. decides at the beginning of 2010 to adopt the FIFO method of inventory valuation. Ramirez had used the LIFO method for financial reporting since its inception on January...

The relationship of which variable with mileage seems to be the weakest? Scatterplot 100 300 500 2 3 4 5 35 30 25 mpg 20 15 10 500 400 300 Displacement of engine 200 100 350 300 250 Horsepower 200...

What milk or dairy preferences do you and your family have? Have they changed? Why or why not?

When should you avoid using exhaust brake select all that apply

A customer order entered into a Manufacturing system or Distribution system will document which the warehouse will need to action. What is the name of this Document?

Overview To become an effective Security Analyst, you need to understand how to use network analysis tools like Wireshark. A carpenter needs to know when to use his table saw vs. when to use his...

Book: An Intro to Combustion 4th ed. by Turns and Haworth Write down the 1-step global reaction for propane-air combustion at stoichiometric conditions. The reaction rate (in kmol/ms) of the fuel...

Which of the following statements is true? (Check all that apply.) A. Cost-effective controls should be implemented to reduce risk. B. Risk should never be accepted, even if it is within the companys...

Which, if any, of the dichloroethene molecules drawn in Data Table II (3.) (4.) and (5.) are geometric isomers? A. B. C. D. cis-1,2-dichloroethene and trans-1,2-dichloroethene...

For the following exercises, the cost of producing x cellphones is described by the function C(x) = x 2 4x + 1000. Find the average rate of change in the total cost as x changes from x = 10 to x =...

For the following exercises, convert the complex number from polar to rectangular form. z = 3cis(240)

For the following exercises, simplify the first trigonometric expression by writing the simplified form in terms of the second expression. cot x; sin x

8. Which area is the main source of input to the cerebral cortexpg109 The drug phenylephrine is sometimes prescribed for people suffering from a sudden loss of blood pressure or other medical...

11. Which lobe of the cerebral cortex includes the primary somatosensory cortexpg109

12. Which lobe of the cerebral cortex includes the primary visual cortexpg109