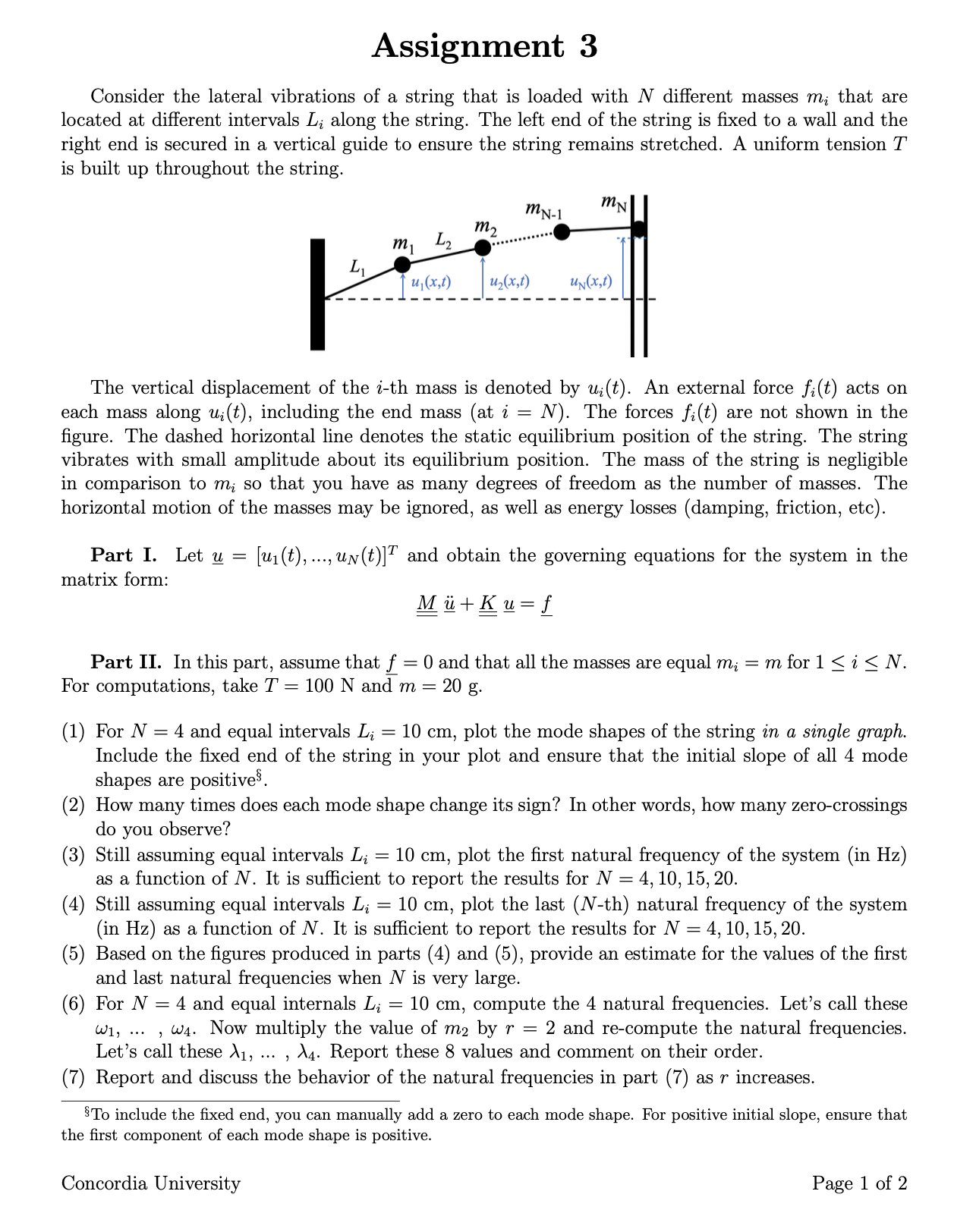
Assignment 3 Consider the lateral vibrations of a string that is loaded with N different masses m; that are located at different intervals L along the string. The left end of the string is fixed to a wall and the right end is secured in a vertical guide to ensure the string remains stretched. A uniform tension T is built up throughout the string. MN-1 MN m2 m1 L2 u(x,t) u2(x,t) UN(x,t) The vertical displacement of the i-th mass is denoted by ui(t). An external force fi(t) acts on each mass along u(t), including the end mass (at i = = N). The forces fi(t) are not shown in the figure. The dashed horizontal line denotes the static equilibrium position of the string. The string vibrates with small amplitude about its equilibrium position. The mass of the string is negligible in comparison to mi so that you have as many degrees of freedom as the number of masses. The horizontal motion of the masses may be ignored, as well as energy losses (damping, friction, etc). Part I. Let u = [u(t), ..., un(t)] and obtain the governing equations for the system in the M+Ku = = f matrix form: Part II. In this part, assume that f = 0 For computations, take T = 100 N and m = (1) For N = 4 and equal intervals Li = and that all the masses are equal m; = m for 1 i N. 20 g. 10 cm, plot the mode shapes of the string in a single graph. Include the fixed end of the string in your plot and ensure that the initial slope of all 4 mode shapes are positive. (2) How many times does each mode shape change its sign? In other words, how many zero-crossings do you observe? (3) Still assuming equal intervals Li as a function of N. It is sufficient (4) Still assuming equal intervals Li = = 10 cm, plot the first natural frequency of the system (in Hz) to report the results for N = 4, 10, 15, 20. 10 cm, plot the last (N-th) natural frequency of the system (in Hz) as a function of N. It is sufficient to report the results for N = 4, 10, 15, 20. (5) Based on the figures produced in parts (4) and (5), provide an estimate for the values of the first and last natural frequencies when N is very large. (6) For N = 4 and equal internals L = 10 cm, compute the 4 natural frequencies. Let's call these W1, , w4. Now multiply the value of m2 by r = 2 and re-compute the natural frequencies. Let's call these \1, 4. Report these 8 values and comment on their order. (7) Report and discuss the behavior of the natural frequencies in part (7) as r increases. To include the fixed end, you can manually add a zero to each mode shape. For positive initial slope, ensure that the first component of each mode shape is positive. Concordia University Page 1 of 2