Answered step by step
Verified Expert Solution
Question
1 Approved Answer
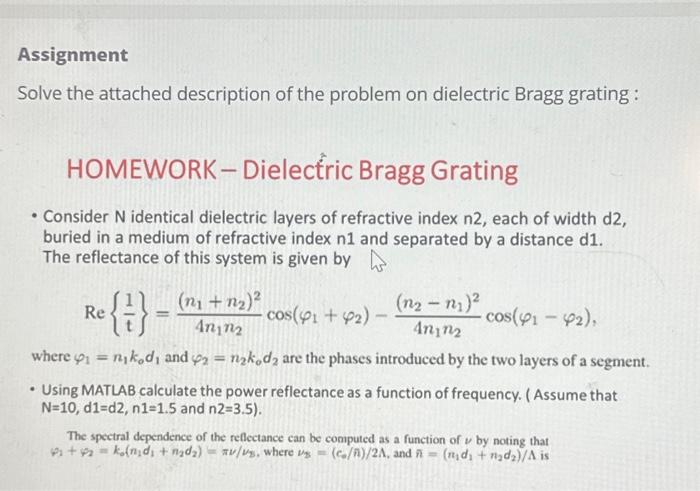
Assignment Solve the attached description of the problem on dielectric Bragg grating : HOMEWORK - Dielectric Bragg Grating - Consider ( mathrm{N} ) identical dielectric
Assignment Solve the attached description of the problem on dielectric Bragg grating : HOMEWORK - Dielectric Bragg Grating - Consider ( mathrm{N} ) identical dielectric layers of refractive index ( mathrm{n} 2 ), each of width ( mathrm{d} 2 ), buried in a medium of refractive index ( mathrm{n} 1 ) and separated by a distance ( mathrm{d} 1 ). The reflectance of this system is given by [ operatorname{Re}left{rac{1}{t} ight}=rac{left(n_{1}+n_{2} ight)^{2}}{4 n_{1} n_{2}} cos left(arphi_{1}+arphi_{2} ight)-rac{left(n_{2}-n_{1} ight)^{2}}{4 n_{1} n_{2}} cos left(arphi_{1}-arphi_{2} ight) ] where ( arphi_{1}=n_{1} k_{o} d_{1} ) and ( arphi_{2}=n_{2} k_{o} d_{2} ) are the phases introduced by the two layers of a segment. - Using MATLAB calculate the power reflectance as a function of frequency. (Assume that ( mathrm{N}=10, mathrm{~d} 1=mathrm{d} 2, mathrm{n} 1=1.5 ) and ( mathrm{n} 2=3.5 ) ). The spectral dependence of the reflectance can be computed as a function of ( u ) by noting that ( arphi_{1}+arphi_{2}=k_{0}left(n_{1} d_{1}+n_{2} d_{2} ight)=pi u / u_{3} ), where ( u_{3}=left(c_{0} / ilde{n} ight) / 2 Lambda ), and ( ar{n}=left(n_{1} d_{1}+n_{2} d_{2} ight) / Lambda ) is
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


