.Assist me with the following macroeconomic questions.

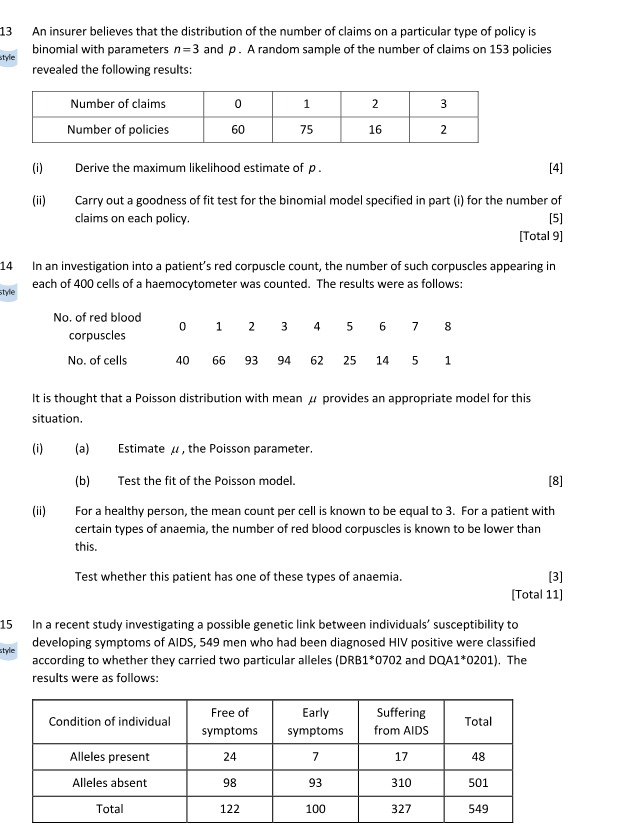
13 An insurer believes that the distribution of the number of claims on a particular type of policy is tyle binomial with parameters n=3 and p. A random sample of the number of claims on 153 policies revealed the following results: Number of claims 0 1 2 3 Number of policies 60 75 16 2 (i) Derive the maximum likelihood estimate of p. [4] (ii) Carry out a goodness of fit test for the binomial model specified in part (i) for the number of claims on each policy. [5] [Total 9] 14 In an investigation into a patient's red corpuscle count, the number of such corpuscles appearing in style each of 400 cells of a haemocytometer was counted. The results were as follows: No. of red blood 1 2 3 4 5 6 7 8 corpuscles No. of cells 40 66 93 94 62 25 14 5 1 It is thought that a Poisson distribution with mean / provides an appropriate model for this situation. (i) (a) Estimate / , the Poisson parameter. (b) Test the fit of the Poisson model. [8] (ii) For a healthy person, the mean count per cell is known to be equal to 3. For a patient with certain types of anaemia, the number of red blood corpuscles is known to be lower than this. Test whether this patient has one of these types of anaemia. (3] [Total 11] 15 In a recent study investigating a possible genetic link between individuals' susceptibility to tyle developing symptoms of AIDS, 549 men who had been diagnosed HIV positive were classified according to whether they carried two particular alleles (DRB1*0702 and DOA1*0201). The results were as follows: Free of Early Condition of individual Suffering from AIDS Total symptoms symptoms Alleles present 24 7 17 48 Alleles absent 98 93 310 501 Total 122 100 327 549The effectiveness of a tablet containing 11 mg of drug 1 and 1:2 mg of drug 2 was being tested. In trials the following results were obtained: 92.5 23.3 94.9 39.5 25.2 94.1 49.2 m 95.: Z}: =4s99 21:1 = 235 252 = 232.3 21:11:11,23233 25.3 =12.335.42 Zyxl = 22112393 2292 =19,39o.22 21:11:: =3.935.95 (i) Using the multiple linear least square regression model: }' = 51' +5111 + 3212 +3 [3] Show that the least squares estimates of or , ,61 and g satisfy: 2 I": =H+ 1512111+ 15221712 2 F1111 = IWEI-"1'1 + 31213 + 1522112111 ZFrIrz = 92112 + 1512111112 + 3221122 (b) Hence, using the above data, nd their numerical values. ['1'] [ii] Pmdict the percentage effectiveness for a tablet containing 51.3 mg of drug 1'1 and 13.3 mg of drug 1:2. [2] [Total 9] A company examining its employee retention rates considers the number of complete years a new employee works for a particular division before leaving. The results are shown in the table below: Division A 6 Division B Division C 8 10 5 7 (i) Perform a one-way analysis of variance at the 5% level to compare the retention rates for the three divisions. [5] (ii) Present the data in a simple diagram and hence comment briefly on the validity of the assumptions required for the analysis of variance. [2] (iii) Concern is expressed over the variability of the results in Division B. It is thought that this might be significantly different from the assumed common underlying variance. (a) Write down an estimate for the underlying common variance for all three divisions. (b) Calculate an unbiased estimate for the variance in Division B based on the data for Division B only. (c) Test at the 5% level whether the variance in Division B is significantly different from your estimate in (iii)(a). [5] [Total 12]7. Based on this model collect the data and answers the given Questions (to obtain the data you can go to https://www.bnm.gov.my/msb-2021 or https://www.mof.gov.my/arkib/ekonomi/ek main.html) you can also access other sources as well The size of the sample must be N > 30 or more than 30 observations ( it is preferable if you can collect up to 40 or more observations) - you can choose either quarterly or yearly data - the data can be up to 2020. Linear Model Semilog linear model Where = Private Investment -Private fixed capital formation (Total Private Investment To GDP (PS/GDP constant price) = Government Budget deficit (the difference between government revenue and government expenses to GDP or GDP constant price ) = BDEF/GDP constant price = Inflation Rate = Government expenditure = Public Investment Total Public Investment (Government Fixed capital formation) to GDP (PA/GDP deflator or GDP constant ) = M3 to GDP (M3/GDP deflator or GDP constant ) = Real GDP (GDP deflator or GDP constant ) Answer these questions a. Estimate the regression coefficients, their standard errors, and obtain R and adjusted R for both models (Semi log linear and linear model) b. Interpret the various regression coefficients for both models (linear and semilog-linear models) c. Are the estimated partial regression coefficients individually statistically significant? Use the p value to answer the question for both estimated models d. From the ANOVA table from both models, test the hypothesis that all independent variables equal to zero. e. Compare the R? values of the linear and semilog-linear regressions. What conclusion do you draw from these computations