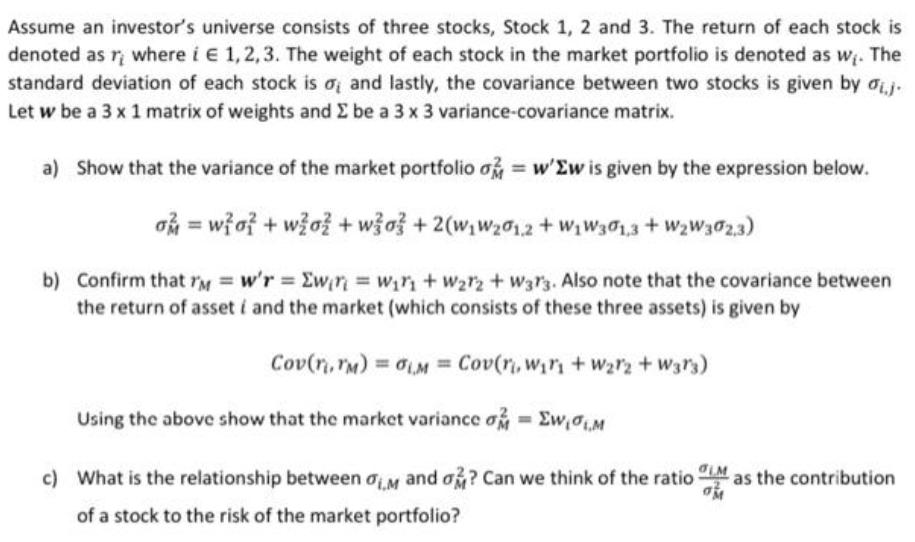
Assume an investor's universe consists of three stocks, Stock 1, 2 and 3. The return of each stock is denoted as r where i 1,2,3. The weight of each stock in the market portfolio is denoted as w. The standard deviation of each stock is o, and lastly, the covariance between two stocks is given by aij. Let w be a 3 x 1 matrix of weights and E be a 3 x 3 variance-covariance matrix. a) Show that the variance of the market portfolio o = w'Ew is given by the expression below. ok = wfof + w?o? + wo + 2(w,w201.2+ W,W301,3+W2W302.3) b) Confirm that rM = w'r Ewrn win+w2r2 +w3r3. Also note that the covariance between the return of asset i and the market (which consists of these three assets) is given by Cov(n, rm) = 0LM = Cov(r, win + wz2 + W3r3) Using the above show that the market variance o = Ew,aM c) What is the relationship between a M and o? Can we think of the ratioM as the contribution of a stock to the risk of the market portfolio?