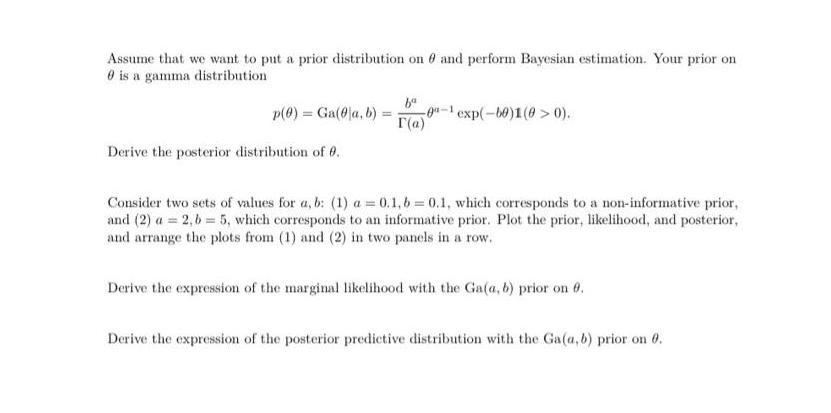
Assume that we want to put a prior distribution on and perform Bayesian estimation. Your prior on is a gamma distribution ba p(0) = Ga(0|a, b) = -0-1 exp(-60)1(0 > 0). r(a) Derive the posterior distribution of 0. Consider two sets of values for a, b: (1) a= 0.1,6=0.1, which corresponds to a non-informative prior, and (2) a = 2,b= 5, which corresponds to an informative prior. Plot the prior, likelihood, and posterior, and arrange the plots from (1) and (2) in two panels in a row. Derive the expression of the marginal likelihood with the Ga(a, b) prior on 9. Derive the expression of the posterior predictive distribution with the Ga(a, b) prior on 0.