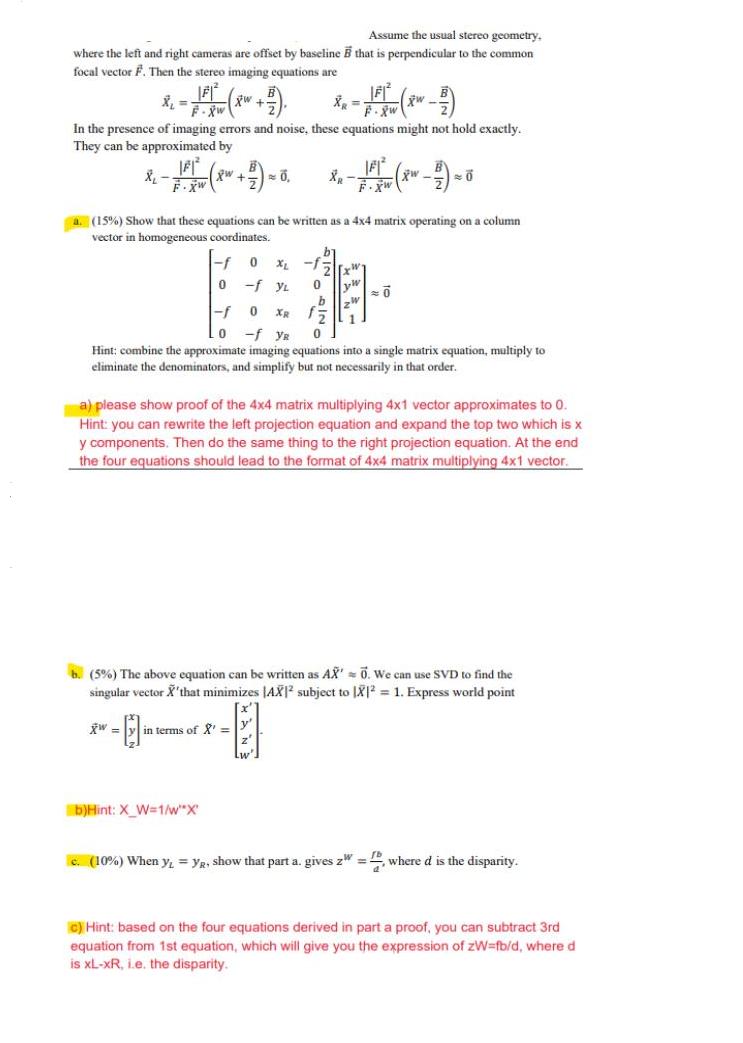
Assume the usual stereo geometry. where the left and right cameras are offset by baseline B that is perpendicular to the common focal vector F. Then the stereo imaging equations are |F| (xw 3.xw In the presence of imaging errors and noise, these equations might not hold exactly. They can be approximated by |A zw = a. (15%) Show that these equations can be written as a 4x4 matrix operating on a column vector in homogeneous coordinates. 0 XL -1 [x" Br 0 -f YL 0 yw b zW -f 0 XR 0 -f YR 0 yw b)Hint: X_W=1/w"X" Hint: combine the approximate imaging equations into a single matrix equation, multiply to eliminate the denominators, and simplify but not necessarily in that order. w= in terms of X'= -1 20 a) please show proof of the 4x4 matrix multiplying 4x1 vector approximates to 0. Hint: you can rewrite the left projection equation and expand the top two which is x y components. Then do the same thing to the right projection equation. At the end the four equations should lead to the format of 4x4 matrix multiplying 4x1 vector. 20 b. (5%) The above equation can be written as AX'= 0. We can use SVD to find the singular vector X'that minimizes |AX| subject to X12= 1. Express world point c. (10%) When y = YR, show that part a. gives z where d is the disparity. c) Hint: based on the four equations derived in part a proof, you can subtract 3rd equation from 1st equation, which will give you the expression of ZW=fb/d, where d is xL-XR, i.e. the disparity.