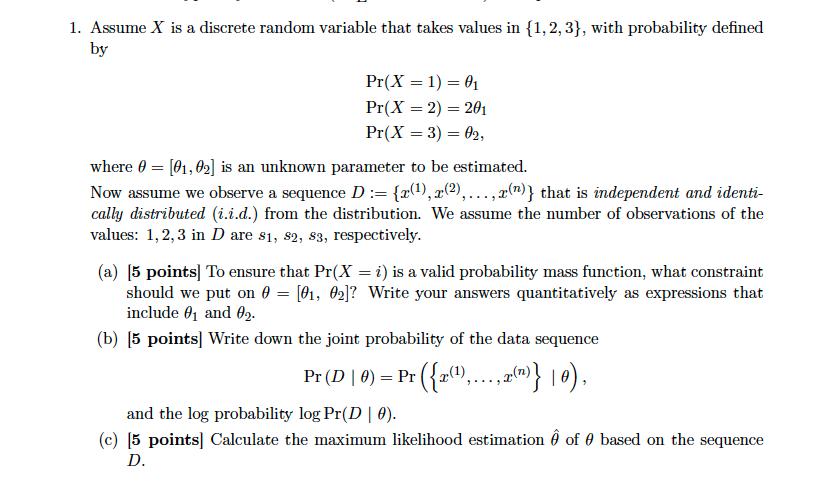
1. Assume X is a discrete random variable that takes values in {1, 2, 3}, with probability defined by Pr(X = 1) = 01 Pr(X = 2) = 201 Pr(X = 3) = 02, = where [01, 02] is an unknown parameter to be estimated. Now assume we observe a sequence D := {x(1), (2),...,x(n)} that is independent and identi- cally distributed (i.i.d.) from the distribution. We assume the number of observations of the values: 1,2,3 in D are 81, 82, 83, respectively. (a) [5 points] To ensure that Pr(X = i) is a valid probability mass function, what constraint should we put on = [01, 02]? Write your answers quantitatively as expressions that include 01 and 02. (b) [5 points] Write down the joint probability of the data sequence Pr (D | 0) = Pr ({2(),..., 2 (n)} |0), and the log probability log Pr(D | 0). (c) [5 points] Calculate the maximum likelihood estimation of based on the sequence D.