Attached image of the problem, only do part b, d and e.

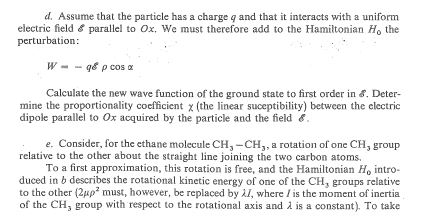
4. Consider a particle P of mass , constrained to move in the xOy plane in a circle centered at O with fixed radius p (a two-dimensional rotator). The only variable of the system is the angle = = (Ox, OP), and the quantum state of the particle is defined by the wave function va) (which represents the probability amplitude of finding the particle at the point of the circle fixed by the angle a). At each point of the circle, (a) can take on only one value, so that: ( + 2x) = (a) Wla) is normalized if: *** Max? da = 1 4. Consider the operator - Is M Hermitian? Calculate the eigen- values and normalized eigenfunctions of M. What is the physical meaning of M? b. The kinetic energy of the particle can be written: H M2 2up? Calculate the eigenvalues and eigenfunctions of Ho Are the energies degenerate? ? c. At i = 0, the wave function of the particle is N cosa (where N is a normal ization coefficient). Discuss the localization of the particle on the circle at a subsequent time d. Assume that the particle has a charge q and that it interacts with a uniform electric field parallel to Ox, We must therefore add to the Hamiltonian Ho the perturbation: W -- 98 p cosa Calculate the new wave function of the ground state to first order in & Deter- mine the proportionality coefficient (the linear suceptibility) between the electric dipole parallel to Ox acquired by the particle and the field & e. Consider, for the ethane molecule CH, -CH,, a rotation of one CH, group relative to the other about the straight line joining the two carbon atoms. To a first approximation, this rotation is free, and the Hamiltonian H, intro duced in b describes the rotational kinetic energy of one of the CH, groups relative to the other (2upa must, however, be replaced by XI, where / is the moment of inertia of the CH, group with respect to the rotational axis and is a constant), To take account of the electrostatic interaction energy between the two CH, groups, we add to H, a term of the form: w b cos 3a where b is a real constant. Give a physical justification for the a-dependence of W. Calculate the energy and wave function of the new ground state (to first order in b for the wave function and to second order for the energy). Give a physical interpretation of the result. 4. Consider a particle P of mass , constrained to move in the xOy plane in a circle centered at O with fixed radius p (a two-dimensional rotator). The only variable of the system is the angle = = (Ox, OP), and the quantum state of the particle is defined by the wave function va) (which represents the probability amplitude of finding the particle at the point of the circle fixed by the angle a). At each point of the circle, (a) can take on only one value, so that: ( + 2x) = (a) Wla) is normalized if: *** Max? da = 1 4. Consider the operator - Is M Hermitian? Calculate the eigen- values and normalized eigenfunctions of M. What is the physical meaning of M? b. The kinetic energy of the particle can be written: H M2 2up? Calculate the eigenvalues and eigenfunctions of Ho Are the energies degenerate? ? c. At i = 0, the wave function of the particle is N cosa (where N is a normal ization coefficient). Discuss the localization of the particle on the circle at a subsequent time d. Assume that the particle has a charge q and that it interacts with a uniform electric field parallel to Ox, We must therefore add to the Hamiltonian Ho the perturbation: W -- 98 p cosa Calculate the new wave function of the ground state to first order in & Deter- mine the proportionality coefficient (the linear suceptibility) between the electric dipole parallel to Ox acquired by the particle and the field & e. Consider, for the ethane molecule CH, -CH,, a rotation of one CH, group relative to the other about the straight line joining the two carbon atoms. To a first approximation, this rotation is free, and the Hamiltonian H, intro duced in b describes the rotational kinetic energy of one of the CH, groups relative to the other (2upa must, however, be replaced by XI, where / is the moment of inertia of the CH, group with respect to the rotational axis and is a constant), To take account of the electrostatic interaction energy between the two CH, groups, we add to H, a term of the form: w b cos 3a where b is a real constant. Give a physical justification for the a-dependence of W. Calculate the energy and wave function of the new ground state (to first order in b for the wave function and to second order for the energy). Give a physical interpretation of the result