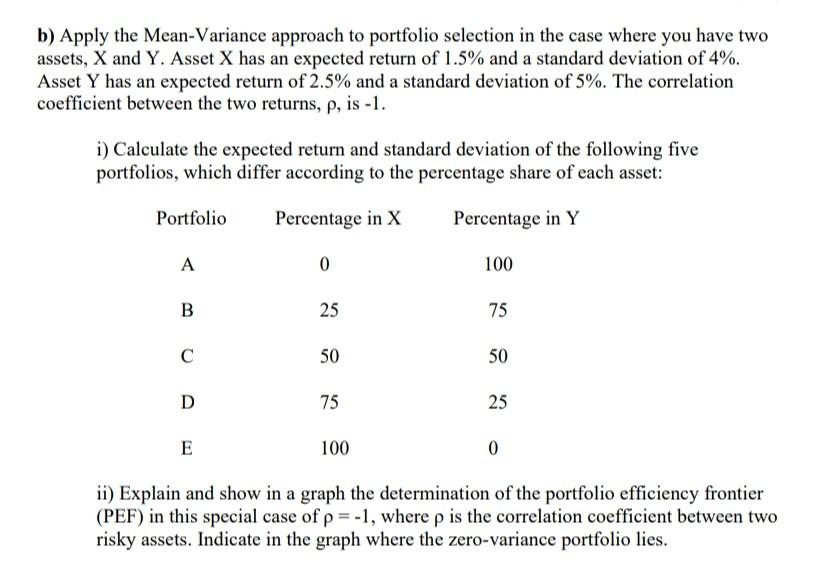
b) Apply the Mean-Variance approach to portfolio selection in the case where you have two assets, X and Y. Asset X has an expected return of 1.5% and a standard deviation of 4%. Asset Y has an expected return of 2.5% and a standard deviation of 5%. The correlation coefficient between the two returns, , is -1. i) Calculate the expected return and standard deviation of the following five portfolios, which differ according to the percentage share of each asset: Portfolio Percentage in X Percentage in Y A 0 100 B 25 75 C 50 50 D 75 25 E 100 0 ii) Explain and show in a graph the determination of the portfolio efficiency frontier (PEF) in this special case of = -1, where is the correlation coefficient between two risky assets. Indicate in the graph where the zero-variance portfolio lies.

want the answer for part b especially.

question b part 2 is needed. the graphical explanation and the details related to it.

b) Apply the Mean-Variance approach to portfolio selection in the case where you have two assets, X and Y. Asset X has an expected return of 1.5% and a standard deviation of 4%. Asset Y has an expected return of 2.5% and a standard deviation of 5%. The correlation coefficient between the two returns, p, is -1. i) Calculate the expected return and standard deviation of the following five portfolios, which differ according to the percentage share of each asset: Portfolio Percentage in X Percentage in Y A 0 100 B 25 75 C 50 50 D 75 25 E 100 0 ii) Explain and show in a graph the determination of the portfolio efficiency frontier (PEF) in this special case of p= -1, where p is the correlation coefficient between two risky assets. Indicate in the graph where the zero-variance portfolio lies. b) Apply the Mean-Variance approach to portfolio selection in the case where you have two assets, X and Y. Asset X has an expected return of 1.5% and a standard deviation of 4%. Asset Y has an expected return of 2.5% and a standard deviation of 5%. The correlation coefficient between the two returns, p, is -1. i) Calculate the expected return and standard deviation of the following five portfolios, which differ according to the percentage share of each asset: Portfolio Percentage in X Percentage in Y A 0 100 B 25 75 C 50 50 D 75 25 E 100 0 ii) Explain and show in a graph the determination of the portfolio efficiency frontier (PEF) in this special case of p= -1, where p is the correlation coefficient between two risky assets. Indicate in the graph where the zero-variance portfolio lies