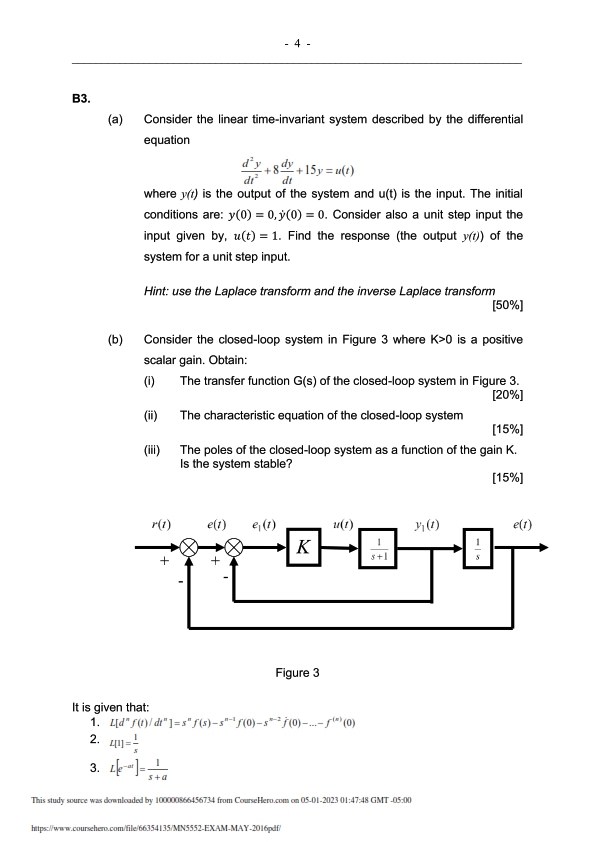
B3. (a) Consider the linear time-invariant system described by the differential equation d'y dr +8dy dt +15y=u(t) where y(t) is the output of the system and u(t) is the input. The initial conditions are: y(0) = 0,(0) = 0. Consider also a unit step input the input given by, u(t) = 1. Find the response (the output y(t)) of the system for a unit step input. Hint: use the Laplace transform and the inverse Laplace transform [50%] (b) Consider the closed-loop system in Figure 3 where K>0 is a positive scalar gain. Obtain: (i) The transfer function G(s) of the closed-loop system in Figure 3. [20%] (ii) The characteristic equation of the closed-loop system [15%] (iii) The poles of the closed-loop system as a function of the gain K. Is the system stable? [15%] r(1) e(1) e(1) u(t) y (1) e(1) K 1 3+1 + Figure 3 It is given that: 1. L[d" f(t)/dt"]=s"(s)-s"(0)-s* (0) -...-(*) (0) 2. 41 3. L s+a This study source was downloaded by 100000866456734 from Course Hero.com on 05-01-2023 01:47:48 GMT-05:00 https://www.coursehero.com/file/66354135/MN5552-EXAM-MAY-2016pdf/